A disconnected capacitor with two dielectrics in parallel

[lorenz0]

Homework Statement

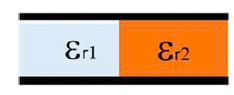
A parallel plate square capacitor with plates of area $A$ at distance $d$ is connected to a battery until there is a potential difference of $V$ between its plates and then is disconnected from the battery. Then half of it is filled with a dielectric with dielectric constant $\varepsilon_1$ and, in parallel, the other half is filled with a dielectric of dielectric constant $\varepsilon_2$.
Calculate the capacitance of the capacitor.
Also calculate the electrostatic field inside the capacitor.

Relevant Equations

$C=Q/V=\varepsilon_0 A/d$, $V=Ed$

I considered the capacitor as two capacitors in parallel, so the total capacitance is $C=C_1+C_2=\frac{\varepsilon_0\varepsilon_1 (A/2)}{d}+\frac{\varepsilon_0\varepsilon_2 (A/2)}{d}=\frac{\varepsilon_0 A}{2d}(\varepsilon_1+\varepsilon_2).$

Since the parallel component of the electric field is continuous, the electric field inside the capacitor is $E=Vd=\frac{Q}{C}d=\frac{Q}{\frac{\varepsilon_0 A}{2d}(\varepsilon_1+\varepsilon_2)}d=\frac{2Qd^2}{\varepsilon_0 A (\varepsilon_1+\varepsilon_2)}$.

Is this correct? I have a doubt; the fact that someone inserted the dielectric should have changed (lowered) the potential $V$ between the plates to $V/\varepsilon_1$ on the left and $V/\varepsilon_2$ on the right: how then can it be that $E_1=E_2=E$ (i.e. that $V_1/d=V_2/d$) across the capacitor?
Thanks

 

[TSny]

I considered the capacitor as two capacitors in parallel, so the total capacitance is $C=C_1+C_2=\frac{\varepsilon_0\varepsilon_1 A}{d}+\frac{\varepsilon_0\varepsilon_2 A}{d}=\frac{\varepsilon_0 A}{d}(\varepsilon_1+\varepsilon_2).$

You have the correct idea here. But, in the statement of the problem, the symbol $A$ is defined as the total area of one of the plates. Does the $A$ in your equation above still represent the total area of one of the plates?

the fact that someone inserted the dielectric should have changed (lowered) the potential $V$ between the plates to $V/\varepsilon_1$ on the left and $V/\varepsilon_2$ on the right: how then can it be that $E_1=E_2=E$ (i.e. that $V_1/d=V_2/d$) across the capacitor?
Thanks

The potential difference between the plates will change when the dielectrics are inserted. If a single uniform dielectric is inserted between the plates of a charged, isolated capacitor with potential $V$, then it does turn out that the potential difference changes to $V/\varepsilon$. But, that doesn't necessarily mean that for the case where you have two different dielectrics, the potential difference would change to $V/\varepsilon_1$ for the left and $V/\varepsilon_2$ for the right. As you noted, this leads to a contradiction as the potential difference between any point of the upper plate and any point of the lower place must be the same independent of the choice of points.

There will be free charge on the plates. This free charge can move around on the plates. So, you need to consider the possibility that the charge per unit area might be different for the left half of the plates compared to the right half. That's how the potential difference can be the same for both halves. You don't need to actually work out the charge densities. Instead, you can just use your result for the equivalent capacitance to find the new potential difference.

 

[lorenz0]

You have the correct idea here. But, in the statement of the problem, the symbol $A$ is defined as the total area of one of the plates. Does the $A$ in your equation above still represent the total area of one of the plates?The potential difference between the plates will change when the dielectrics are inserted. If a single uniform dielectric is inserted between the plates of a charged, isolated capacitor with potential $V$, then it does turn out that the potential difference changes to $V/\varepsilon$. But, that doesn't necessarily mean that for the case where you have two different dielectrics, the potential difference would change to $V/\varepsilon_1$ for the left and $V/\varepsilon_2$ for the right. As you noted, this leads to a contradiction as the potential difference between any point of the upper plate and any point of the lower place must be the same independent of the choice of points.

There will be free charge on the plates. This free charge can move around on the plates. So, you need to consider the possibility that the charge per unit area might be different for the left half of the plates compared to the right half. That's how the potential difference can be the same for both halves. You don't need to actually work out the charge densities. Instead, you can just use your result for the equivalent capacitance to find the new potential difference.

Thank you very much, your answer has been very helpful: I now understand how there could be only one potential difference across the capacitor.
I have corrected my calculations to account for the fact that each dielectric occupies only half of the space and I have also calculated the final electric field across the capacitor: would you mind checking my work out? Thanks again.

 

[TSny]

I considered the capacitor as two capacitors in parallel, so the total capacitance is $C=C_1+C_2=\frac{\varepsilon_0\varepsilon_1 (A/2)}{d}+\frac{\varepsilon_0\varepsilon_2 (A/2)}{d}=\frac{\varepsilon_0 A}{2d}(\varepsilon_1+\varepsilon_2).$

OK

Since the parallel component of the electric field is continuous, the electric field inside the capacitor is $E=Vd=\frac{Q}{C}d=\frac{Q}{\frac{\varepsilon_0 A}{2d}(\varepsilon_1+\varepsilon_2)}d=\frac{2Qd^2}{\varepsilon_0 A (\varepsilon_1+\varepsilon_2)}$.

You made a careless slip when you wrote $E = Vd$.

You should write your final answer for $E$ in terms of the given quantities. In particular, write the answer in terms of the original $V$ instead of $Q$.

 

[TSny]

As a general rule, if the work you show in your original post has some errors, then your corrections should be made in a separate post in the thread. If you make the corrections by editing your original post after others have responded to your original post, then it can be confusing. For now, I would leave things as they are currently in the first post.

 

[lorenz0]

OK

You made a careless slip when you wrote $E = Vd$.

You should write your final answer for $E$ in terms of the given quantities. In particular, write the answer in terms of the original $V$ instead of $Q$.

OK, then $E=\frac{Q}{\frac{\varepsilon_0 A}{2d}(\varepsilon_1+\varepsilon_2)}=\frac{Q}{\frac{\varepsilon_0 A}{d}(\frac{\varepsilon_1+\varepsilon_2}{2})}=\frac{2V}{\varepsilon_1+\varepsilon_2}$, since $V=\frac{Q}{C}$ and $C=\frac{\varepsilon_0 A}{d}$.

 

[TSny]

OK, then $E=\frac{Q}{\frac{\varepsilon_0 A}{2d}(\varepsilon_1+\varepsilon_2)}=\frac{Q}{\frac{\varepsilon_0 A}{d}(\frac{\varepsilon_1+\varepsilon_2}{2})}=\frac{2V}{\varepsilon_1+\varepsilon_2}$

Did you mean to write $V'$ instead of $E$ on the far left? Otherwise, the equation is not dimensionally consistent. I'm using $V'$ to denote the final potential difference.

 

[lorenz0]

$E'=\frac{V'}{d}=\frac{1}{d}\frac{Q}{C'}=\frac{1}{d}\frac{Q}{\varepsilon\frac{A}{2d}(\varepsilon_1 +\varepsilon_2)}=\frac{2Qd}{\varepsilon A (\varepsilon_1+\varepsilon_2)}\frac{1}{d}=\frac{2V}{d(\varepsilon_1+\varepsilon_2)}$ where $E',\ V'$ are the final electric field and potential respectively, and $V$ is the initial potential.

 

[TSny]

$E'=\frac{V'}{d}=\frac{1}{d}\frac{Q}{C'}=\frac{1}{d}\frac{Q}{\varepsilon\frac{A}{2d}(\varepsilon_1 +\varepsilon_2)}=\frac{2Qd}{\varepsilon A (\varepsilon_1+\varepsilon_2)}\frac{1}{d}=\frac{2V}{d(\varepsilon_1+\varepsilon_2)}$ where $E',\ V'$ are the final electric field and potential respectively, and $V$ is the initial potential.

That looks good to me.