Closure & Interior as Dual Notions .... Proving Willard Theorem 3.11 ...

In summary, the conversation discusses the need for help in proving Theorem 3.11 Part 1-a using the duality relations between closure and interior. The definitions of closure and Theorem 3.11 are mentioned, as well as the dual result in Theorem 3.7. The conversation also mentions an attempt to prove the theorem using the dual relations and asks for assistance in proceeding with the proof. The expert advises to start the argument with ##X-A\subset\overline{X-A}## and reminds that, by definition, ##A^{\circ}## is a subset of ##A##.
  • #1
Math Amateur
Gold Member
MHB
3,990
48
TL;DR Summary
I am reading Stephen Willard: General Topology ... ... and am studying Chapter 2: Topological Spaces and am currently focused on Section 3: Fundamental Concepts ... ...

I need help in order to prove Theorem 3.11 Part 1-a using the duality relations between closure and interior ... ..
I am reading Stephen Willard: General Topology ... ... and am studying Chapter 2: Topological Spaces and am currently focused on Section 3: Fundamental Concepts ... ...

I need help in order to prove Theorem 3.11 Part 1-a using the duality relations between closure and interior ... ..The definition of interior and Theorem 3.11 read as follows:
Willard - Interior ... Defn 3.9, Lemma 3.10 and Theorem 3.11 .png
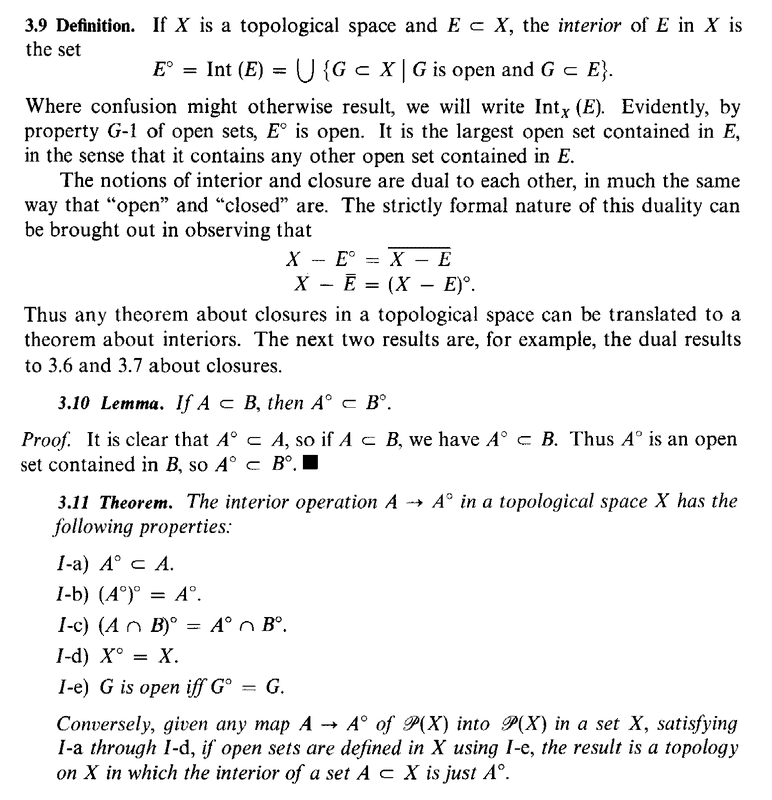Readers of this post necessarily need access to the "dual" theorem ... namely Theorem 3.7 ...

Theorem 3.7 (together with Willard's definition of closure and a relevant lemma) reads as follows:
Willard - Defn 3.5, Lemma 3..6 and Theorem 3.7 .png
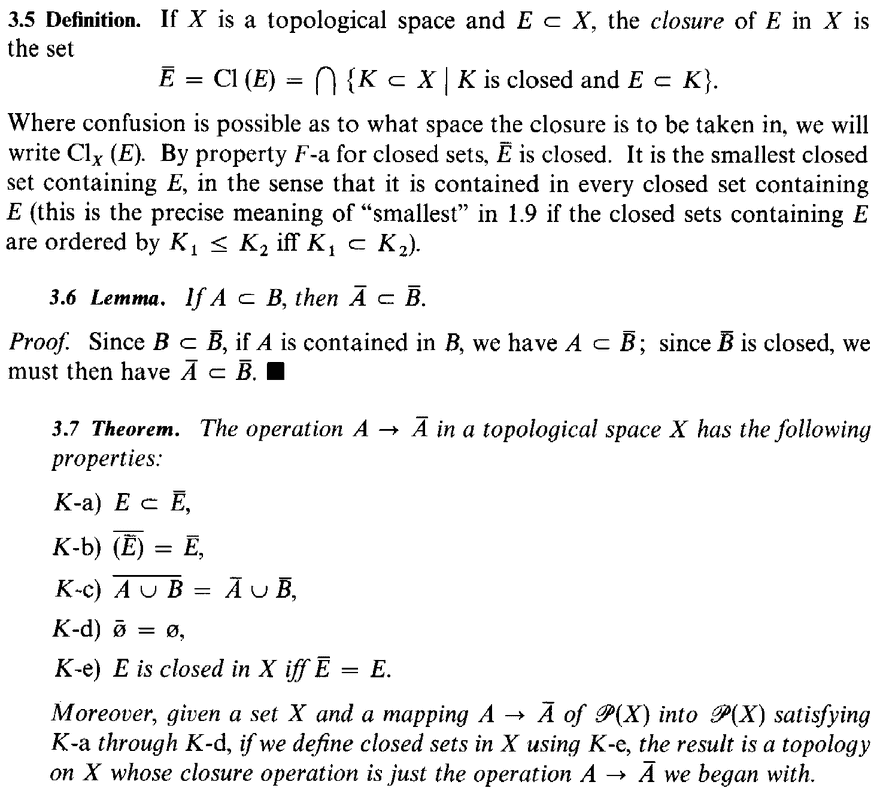So ... I need help in order to prove Theorem 3.11 1-a assuming the dual result in Theorem 3.7 ( that is K-a or ##A \subset \overline{A}## ) using only the definitions of closure and interior and the dual relations: ##X - A^{ \circ } = \overline{ X - A }## and ##X - \overline{ A} = ( X - A)^{ \circ }## ...

My attempt so far is as follows:

To show ##A^{ \circ } \subset A## ...

Proof:

Assume ##A \subset \overline{ A}## ..

Now we have that ...

##A \subset \overline{ A}##

##\Longrightarrow X - \overline{ A} \subset X - A##

##\Longrightarrow (X - A)^{ \circ } \subset X - A## ...But how do I proceed from here ... ?Help will be much appreciated ... ...

Peter
 
Physics news on Phys.org
  • #2
Try your argument again, but starting with ##X-A\subset \overline{X-A}## instead of with ##A\subset\overline{A}##.

But there's no need to use any previous theorems here: by your definition, ##A^{\circ}## is a union of sets all of which are subsets of ##A##, so ##A^{\circ}## is a subset of ##A##.
 
Last edited:
  • Like
Likes member 587159
  • #3
Thanks ...

I understand there is no need to use previous theorems ... just wanted to understand how duality between closure and interior worked ...

Will try your suggestion ...

Thanks again ...

Peter
 

1. What is the significance of Closure and Interior as Dual Notions in mathematics?

Closure and Interior are two fundamental concepts in topology that are considered dual notions, meaning they are closely related and can be used to prove theorems about each other. Closure refers to the set of all points that are either in a given set or are limit points of that set, while Interior refers to the set of all points that are contained within a given set. These concepts are essential in understanding the structure and properties of topological spaces.

2. What is Willard Theorem 3.11 and how does it relate to Closure and Interior?

Willard Theorem 3.11 is a fundamental theorem in topology that states that the closure of a set is equal to the complement of the interior of the complement of that set. In simpler terms, this means that the closure of a set is equal to the set of all limit points of that set. This theorem is closely related to the dual notions of Closure and Interior, as it provides a way to prove properties of one concept using the other.

3. How is Willard Theorem 3.11 proven?

Willard Theorem 3.11, also known as the "Closure-Interior Theorem," can be proven using basic set theory and the definitions of Closure and Interior. The proof involves showing that the closure of a set is contained within the complement of the interior of the complement of that set, and vice versa. This can be done by considering the definitions of Closure and Interior and using logical arguments to show their equivalence.

4. What are some applications of Closure and Interior as Dual Notions?

Closure and Interior as Dual Notions have numerous applications in mathematics, particularly in the fields of topology and analysis. They are used to prove theorems and properties of topological spaces, such as compactness and connectedness. They also have applications in real analysis, where they are used to define and study concepts such as closure and interior of sets.

5. Can Closure and Interior be used in other areas of science?

Yes, the concepts of Closure and Interior have applications in various areas of science, including physics, computer science, and engineering. In physics, they are used to study the behavior of physical systems, while in computer science, they are used to analyze algorithms and data structures. In engineering, they are used to design and analyze complex systems and structures.

Similar threads

I Interior and Closure in a Topological Space .... .... remark by Willard
    • Topology and Analysis
Replies
2
Views
1K
I Closure in a Topological Space .... Willard, Theorem 3.7 .... ....
    • Topology and Analysis
Replies
5
Views
1K
I Munkres Chapter 5: Problem involving the Tychonoff Theorem
    • Topology and Analysis
Replies
7
Views
2K
MHB Checking Proof of Theorem 6.2.8 Part (ii)
    • Topology and Analysis
Replies
2
Views
2K
A Baire Category Theorem .... Stromberg, Theorem 3.55 .... ....
    • Topology and Analysis
Replies
5
Views
3K
MHB Understanding Topology: Closure, Boundary & Open/Closed Sets
    • Topology and Analysis
Replies
2
Views
1K
I Limit Points & Closure in a Topological Space .... Singh, Theorem 1.3.7
    • Topology and Analysis
Replies
4
Views
2K
MHB Open Sets in R .... .... Willard, Example 2.7 (a) .... ....
    • Topology and Analysis
Replies
3
Views
1K
I Baire Category Theorem: Question About Countable Dense Open Sets
    • Topology and Analysis
Replies
3
Views
2K
MHB Baire Category Theorem .... Stromberg, Theorem 3.55 .... ....
    • Topology and Analysis
Replies
7
Views
2K

Hot Threads

Recent Insights

Back
Top