Corollary to the Interior Extremum Theorem .... ....

[Math Amateur]

I am reading "Introduction to Real Analysis" (Fourth Edition) by Robert G Bartle and Donald R Sherbert ...

I am focused on Chapter 6: Differentiation ...

I need help in fully understanding the corollary to Theorem 6.2.1 ...

Theorem 6.2.1 and its corollary ... ... read as follows:

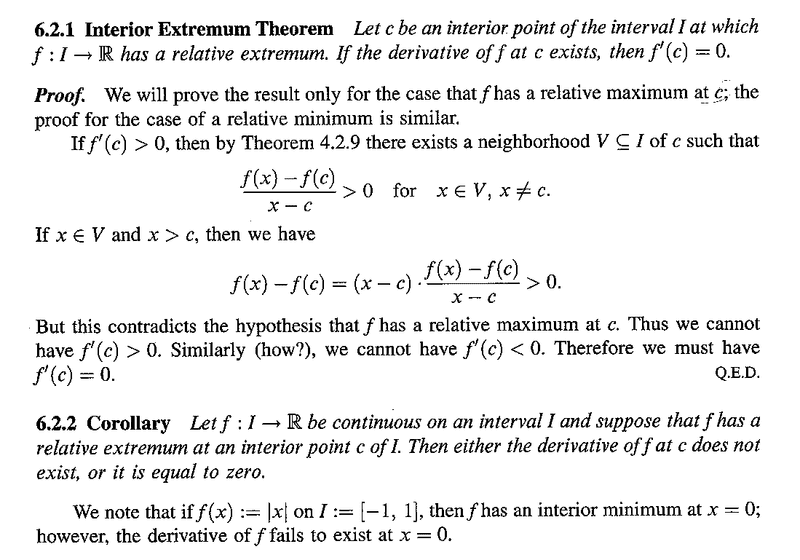I am trying to fully understand the proof of the corollary ...

I was given the following proof by GJA (Math Help Boards) ... ...

"Either the derivative of $f$ at $x=c$ exists or it doesn't, and these are the only two possibilities. If it does, then $f′(c)=0$ from the theorem."BUT ... GJA's proof does not use the Corollary's assumption of continuity of $f$ ...

Is something amiss with GJA's proof ... ?

Peter*** EDIT ***

Note that Manfred Stoll in his book "Introduction to Real Analysis" gives the same theorem and corollary (Theorem 5.2.2 and Corollary 5.2.3) and again gives the condition that $f$ is continuous ... in Stoll's case that f is continuous on $[a, b]$ ...

 

[andrewkirk]

The conclusion of 6.2.2 is identical to the conclusion of 6.2.1 because $P\to C$ is logically equivalent to $(\neg P)\vee C$. Indeed, in most logical languages that use both the $\to$ (implies) and $\vee$ (or) symbols, one of them is defined by that equivalence.

Further, the premises of 6.2.1 and 6.2.2 seem to be the same, except that 6.2.2 adds an additional premise, of continuity. Since the conclusions are the same, and the premises of 6.2.1 are weaker than those of 6.2.2, 6.2.2 follows automatically from 6.2.1. I cannot see any reason for adding the continuity assumption in 6.2.2.

I can't see anything wrong with what GJA wrote. What mystifies me is why the author even bothered to write 6.2.2 (which appears to me to add as much information as a theorem that 'a bald man is a man') and why they introduced continuity.

There is a non-trivial corollary that would use continuity, as follows:

Let $f:I\to\mathbb R$ be continuous on interval $(a,b)$ and suppose that $f(c)=\min f(I)$. Then either the derivative of $f$ at $c$ does not exist or $f'(c)=0$.

I have not proven the theorem but I suspect it's true. Note the crucial difference that $c$ is the location of the minimum over $I$, rather than just a relative minimum (minimum on an open neighbourhood containing $c$) as in 6.2.1.

Maybe the authors meant to write something like this instead.

 

[fresh_42]

I wouldn't give too much weight to it. At points where $f$ isn't continuous, $f'$ doesn't exist either, so it's boring to talk about those points. How does theorem 4.2.9 read? Does it use continuity, cause it's used to prove theorem 6.2.1, in which case the condition is missing here. On the other hand, theorem 6.2.1 only makes an assertion about points, where $f$ is differentiable, which means especially continuous. So if $f$ is not continuous, then theorem 6.2.1 cannot be applied at such a discontinuity.

 

[Math Amateur]

I wouldn't give too much weight to it. At points where $f$ isn't continuous, $f'$ doesn't exist either, so it's boring to talk about those points. How does theorem 4.2.9 read? Does it use continuity, cause it's used to prove theorem 6.2.1, in which case the condition is missing here. On the other hand, theorem 6.2.1 only makes an assertion about points, where $f$ is differentiable, which means especially continuous. So if $f$ is not continuous, then theorem 6.2.1 cannot be applied at such a discontinuity.

Andrew, fresh_42

Thanks for the helpful clarification...

Peter