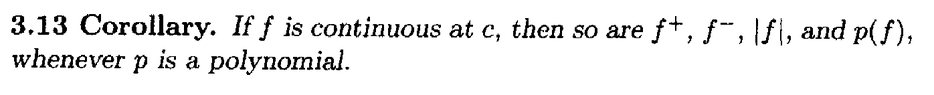Continuity of f^+ .... Browder Corollary 3.13

[Math Amateur]

I am reading Andrew Browder's book: "Mathematical Analysis: An Introduction" ... ...

I am currently reading Chapter 3: Continuous Functions on Intervals and am currently focused on Section 3.1 Limits and Continuity ... ...

I need some help with the proof of Corollary 3.13 ...Corollary 3.13 reads as follows:View attachment 9520Can someone help me to prove that if \(\displaystyle f\) is continuous then \(\displaystyle f^+ = \text{max} (f, 0)\) is continuous ...My thoughts are as follows:If \(\displaystyle c\) belongs to an interval where \(\displaystyle f\) is positive then \(\displaystyle f^+\) is continuous since \(\displaystyle f\) is continuous ... further, if \(\displaystyle c\) belongs to an interval where \(\displaystyle f\) is negative then \(\displaystyle f^+\) is continuous since \(\displaystyle g(x) = 0\) is continuous ... but how do we construct a proof for those points where \(\displaystyle f(x)\) crosses the \(\displaystyle x\)-axis ... ..

Help will be much appreciated ...

Peter

 

[HallsofIvy]

To prove $$f^+$$ is continuous at $$x_0$$ consider 3 cases:
1) $$f(x_0)> 0$$.
2) $$f(x_0)= 0$$.
3) $$f(x_0)< 0$$.

Since f is continuous, in case (1) there exist an interval around $$x_0$$ such that f(x)> 0

and $$f^+(x)= f(x)$$​

for all x in the interval

Since f is continuous, in case (1) there exist an interval around $$x_0$$ such that f(x)< 0 and $$f^+(x)= 0$$ for all x in the interval.​

 

[Math Amateur]

To prove $$f^+$$ is continuous at $$x_0$$ consider 3 cases:
1) $$f(x_0)> 0$$.
2) $$f(x_0)= 0$$.
3) $$f(x_0)< 0$$.

Since f is continuous, in case (1) there exist an interval around $$x_0$$ such that f(x)> 0

and $$f^+(x)= f(x)$$​

for all x in the interval

Since f is continuous, in case (1) there exist an interval around $$x_0$$ such that f(x)< 0 and $$f^+(x)= 0$$ for all x in the interval.​

Thanks for the help ...

BUT ... you do not describe what to do in case 2 ... and as I indicated it is when f(x) = 0, specifically when f crosses the x-axis that I am having trouble dealing with ...

Peter

 

[Math Amateur]

Thanks for the help ...

BUT ... you do not describe what to do in case 2 ... and as I indicated it is when f(x) = 0, specifically when f crosses the x-axis that I am having trouble dealing with ...

Peter

After reflecting on this problem for some time ... here is my proof for the situation where the point investigated is a point where \(\displaystyle f\) crosses the x-axis ...I think it will suffice to prove that \(\displaystyle f^+\) is continuous for the case where a point \(\displaystyle c_1 \in \mathbb{R}\) is such that for \(\displaystyle x \lt c_1, \ f(x) = f^+(x)\) is positive and for \(\displaystyle x \gt c_1, \ f^+(x) = 0\) ... ... while for some point \(\displaystyle c_2 \gt c_1\) we have that \(\displaystyle f^+(x) = 0\) for \(\displaystyle x \lt c_2\) and \(\displaystyle f(x) = f^+(x)\) is positive for \(\displaystyle x \gt c_2\) ... ... ... see Figure 1 below ...

View attachment 9522Now consider an (open) neighbourhood \(\displaystyle V\) of \(\displaystyle f^+(c_1)\) where...
\(\displaystyle V= \{ f^+(x) \ : \ -f^+(a_1) \lt f^+(c_1) \lt f^+(a_1)\) for some \(\displaystyle a_1 \in \mathbb{R} \}

\)

so ...\(\displaystyle V= \{ f^+(x) \ : \ -f^+(a_1) \lt 0 \lt f^+(a_1)\) for some \(\displaystyle a_1 \in \mathbb{R} \}\) ...

Then ... (see Figure 1) ...
\(\displaystyle (f^+)^{ -1 } (V) = \{ a_1 \lt x \lt a_2 \}\) which is an open set as required ...
Further crossings of the x-axis by \(\displaystyle f\) just lead to further sets of the nature \(\displaystyle \{ a_{ n-1 } \lt x \lt a_n \}\) which are also open ... so ...

\(\displaystyle (f^+)^{ -1 } (V) = \{ a_1 \lt x \lt a_2 \} \cup \{ a_3 \lt x \lt a_4 \} \cup \ldots \cup \{ a_{n-1} \lt x \lt a_n \}\)
which being a union of open sets is also an open set ...

The proof is similar if \(\displaystyle f\) first crosses the x-axis from below ...

Is that correct?Peter

 

[HallsofIvy]

To prove $$f^+$$ is continuous at $$x_0$$ consider 3 cases:
1) $$f(x_0)> 0$$.
2) $$f(x_0)= 0$$.
3) $$f(x_0)< 0$$.

Since f is continuous, in case (1) there exist an interval around $$x_0$$ such that f(x)> 0

and $$f^+(x)= f(x)$$​

for all x in the interval

Since f is continuous, in case (1) there exist an interval around $$x_0$$ such that f(x)< 0 and $$f^+(x)= 0$$ for all x in the interval.​

TYPO: in the last sentence "case (1)" should have been "case (2)".