Derivation of Cauchy-Schwarz Inequality

[member 731016]

For this,

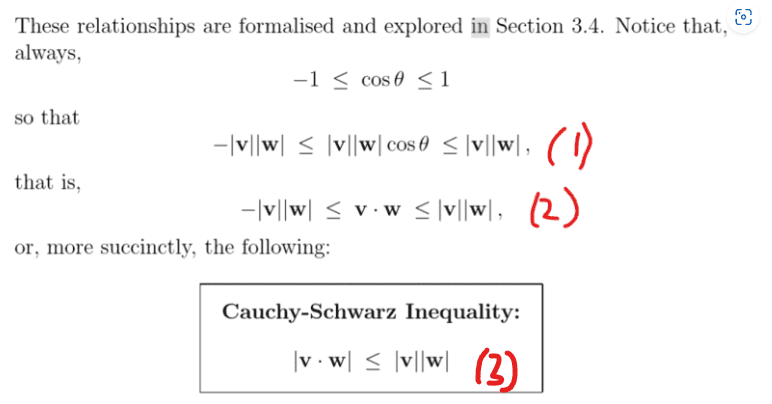
I don't understand how they got from (1) to (2)? Dose someone please know what binary operation allows for that?

I also don't understand how they algebraically got from line (2) to (3).

Many thanks!

 

[jedishrfu]

They used the geometric definition of vector dot product which is:

$u \cdot v = |u| |v| cos(\theta)$ where its understood that $\theta$ is the angle between u and v

https://en.wikipedia.org/wiki/Dot_product

 

[member 731016]

They used the geometric definition of vector dot product which is:

$u \cdot v = |u| |v| cos(\theta)$ where its understood that $\theta$ is the angle between u and v

https://en.wikipedia.org/wiki/Dot_product

Thank you for your reply @jedishrfu !

Sorry if I was not clear. How did they go from $-|v||w| ≤ v \cdot w ≤ |v||w|$ be equal to $|v \cdot w| ≤ |v||w|$? The textbook dose not show any immediate steps.

I also don't know what allowed them to multiply all of $-1 ≤ \cos\theta ≤ 1$ by $|v||w|$ to get line (2).

Many thanks!

 

[Ibix]

Sorry if I was not clear. How did they go from $-|v||w| ≤ v \cdot w ≤ |v||w|$ be equal to $|v \cdot w| ≤ |v||w|$? The textbook dose not show any immediate steps.

Try it the other way. What does $|x|\leq a$ tell you about the range of allowed values for $x$?

I also don't know what allowed them to multiply all of $-1 ≤ \cos\theta ≤ 1$ by $|v||w|$ to get line (2).

If one number (e.g. $\cos\theta$) is less than another (e.g. $1$) and I multiply both by some positive value (e.g. $|v||w|$), do you think the product with the smaller value ($|v||w|\cos\theta$) could ever be larger than the product with the larger value ($1|v||w|$)?

 

[member 731016]

Try it the other way. What does $|x|\leq a$ tell you about the range of allowed values for $x$?

Thank you for your reply @Ibix!

The range of allowed values of x have to such that their abs is less than or equal to a.

Many thanks!

 

[member 731016]

If one number (e.g. $\cos\theta$) is less than another (e.g. $1$) and I multiply both by some positive value (e.g. $|v||w|$), do you think the product with the smaller value ($|v||w|\cos\theta$) could ever be larger than the product with the larger value ($1|v||w|$)?

Thank you for your reply @Ibix!

No I don't think so.

Many thanks!

 

[malawi_glenn]

$-|v||w| ≤ v \cdot w ≤ |v||w|$ be equal to $|v \cdot w| ≤ |v||w|$?

By definition, if $-b \leq a \leq b$ then we write this as $|a| \leq b$

 

[member 731016]

By definition, if $-b \leq a \leq b$ then we write this as $|a| \leq b$

Thank you for your reply @malawi_glenn! Sorry, where did you read that is true? I am not sure where it is derived from. I have never seen that before sorry.

Many thanks!

 

[malawi_glenn]

Thank you for your reply @malawi_glenn! Sorry, where did you read that is true? I am not sure where it is derived from. I have never seen that before sorry.

Many thanks!

Any math textbook on pre calc should cover this

https://en.wikipedia.org/wiki/Absolute_value

And it is not derived it is a definition, a way of writing more compact

 

[member 731016]

Any math textbook on pre calc should cover this

https://en.wikipedia.org/wiki/Absolute_value

Thank you @malawi_glenn ! It looks like it called absolute value inequalities. https://openstax.org/books/college-...-inequalities-and-absolute-value-inequalities

 

[Ibix]

Thank you for your reply @malawi_glenn! Sorry, where did you read that is true? I am not sure where it is derived from. I have never seen that before sorry.

Draw a number line. Mark $0$ and $\pm b$. What region of this line can $a$ lie in if $|a|\leq b$? What region can it lie in if $-b\leq a\leq b$?

 

[member 731016]

Draw a number line. Mark $0$ and $\pm b$. What region of this line can $a$ lie in if $|a|\leq b$? What region can it lie in if $-b\leq a\leq b$?

Thank you for your reply @lbix ! I will do some thinking. Many thanks!

 

[Euge]

If $-b \le a \le b$, then $a \le b$ and $-b \le a$ (or, $-a \le b$). So $\pm a \le b$. Since $|a|$ is either $a$ or $-a$, then $|a| \le b$.

 

[member 731016]

If $-b \le a \le b$, then $a \le b$ and $-b \le a$ (or, $-a \le b$). So $\pm a \le b$. Since $|a|$ is either $a$ or $-a$, then $|a| \le b$.

Thank you for your reply @Euge!

Could you also equally just take the absolute value of the of both sides of the inequality $\pm a \le b$ instead of using the definition of absolute value? This would give $|a| \le |b|$

Many thanks!

 

[Euge]

Could you also equally just take the absolute value of the of both sides of the inequality $\pm a \le b$ instead of using the definition of absolute value? This would give $|a| \le |b|$

In general, the inequality $a \le b$ does not imply $|a| \le |b|$. For example, $-2 \le 1$ but $|-2| > |1|$ since $|-2| = 2$ and $|-1| = 1$.