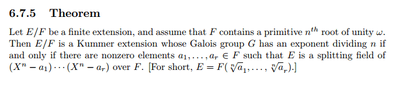E/F is an extension with radicals of order n

[mathmari]

Hey!

We have that $E/F$ is an extension Kummer of degree $n$ and that $F$ contains a $n$-th unit root $\omega$ with $\text{ord} (\omega)=n$.

I want to show that $E/F$ is an extension with radicals of order $n$. I have found the following theorem:

View attachment 6282

Could we maybe use that theorem in this case? So, do we have to show that the Galois group of the extension has an exponent dividing $n$ ? (Wondering)

 

[jvicens]

Hello! Yes, you are on the right track. The theorem you mentioned is the key to showing that $E/F$ is an extension with radicals of order $n$. We can use the fact that $\omega$ has order $n$ to show that the Galois group of $E/F$ has an exponent dividing $n$. This is because the Galois group acts on the $n$-th roots of unity in $F$, and since $\omega$ is a primitive $n$-th root of unity, it generates all the $n$-th roots of unity in $F$. Therefore, the Galois group has to have an exponent dividing $n$, which means that $E/F$ is an extension with radicals of order $n$. Hope this helps!