Undergrad Empty Lattice approximation/Nearly-free electron model

[WWCY]

Hi all, I am having trouble understanding the some ideas presented in some notes I've been reading, help is greatly appreciated!

I've uploaded screenshots of the material I'm referring to below, the last two images are what I'm mainly referencing, and the first few are to provide context (sufficiently I hope). Question 1: last image (empty-lattice approx)
By taking the potential terms to be 0, the energy was shown to be
$$E = \frac{\hbar^2 (\vec{q} - \vec{G'})}{2m}$$
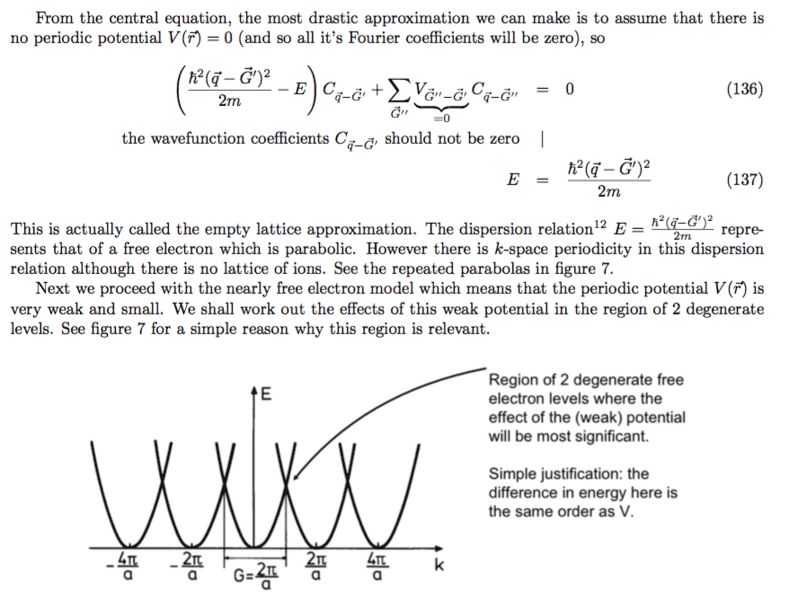
Where $\vec{q}$ is some vector in the first Brillouin Zone and $\vec{G'}$ is a reciprocal translation vector that maps wavevector $\vec{k}$ back to $\vec{q}$. The dispersion was then plotted out. However, how does one infer the periodicity of the dispersion from this energy equation? All I can tell is that $q \in [- \pi / a , \pi/a)$ and $G$ simply translates every $k$ outside of 1BZ back to some q, but all i can sketch out (E vs K) is a big parabola stretching to infinity as K gets larger. What should my thinking process be when making the sketch?

Question 2: last image (empty-lattice approx)

It was mentioned here on the figure that there were degenerate energy levels. However I can't see where this degeneracy comes from. In equation (136), all we have done is find out that the solution to the central equation (first image (131)),
$$ \psi( \vec{r} , \vec{q} ) = \sum_{ \vec{G'} } C_{ \vec{q}- \vec{G'} } e^{i ( \vec{q} - \vec{G'} ) \cdot \vec{r} } $$
has the energy dispersion given above. How does this imply any kind of degeneracy?

Many thanks in advance!

 

[DrDu]

You should see that states $\psi_k= \exp(ikx)$ and $\psi_{k'}=\exp(ik'x)$ with $k=\pi/a$ and $k'=-\pi/a$ are degenerate. This does seem trivial, as it holds for any a. But the point is that in a real lattice, in the simplest case, there will be atoms with a spacing of a, and while the combination $\psi_k+\psi_{k'}\sim \cos(kx)$ will have maxima at the positions x=0,a,..., the other combination $\psi_k-\psi_{k'}\sim \sin(kx)$ will be zero at these points. Hence once you go beyond the empty lattice approximation, the degeneracy will be split for the points on the boundary of the Brillouin zone.

 

[WWCY]

You should see that states $\psi_k= \exp(ikx)$ and $\psi_{k'}=\exp(ik'x)$ with $k=\pi/a$ and $k'=-\pi/a$ are degenerate. This does seem trivial, as it holds for any a. But the point is that in a real lattice, in the simplest case, there will be atoms with a spacing of a, and while the combination $\psi_k+\psi_{k'}\sim \cos(kx)$ will have maxima at the positions x=0,a,..., the other combination $\psi_k-\psi_{k'}\sim \sin(kx)$ will be zero at these points. Hence once you go beyond the empty lattice approximation, the degeneracy will be split for the points on the boundary of the Brillouin zone.

Hi , thank you for your reply. While I believe I see why $\psi_{\pm k}= \exp(\pm ikx)$ are degenerate states, I'm still slightly confused as to how the periodic graph was formed, all I can picture is a $k^2$ plot extending to infinity as $k \rightarrow \pm \infty$, rather than the periodic plot given above. Could you explain why?

Thanks!