Exploring the Inönü-Wigner Contraction of Poincaré $\oplus$ $\mathfrak{u}$(1)

[Jason Bennett]

Homework Statement

Poincaré $\oplus$ $\mathfrak{u}$(1)

Relevant Equations

Poincaré $\oplus$ $\mathfrak{u}$(1)

Please see https://physics.stackexchange.com/questions/552410/inönü-wigner-contraction-of-poincaré-oplus-mathfraku1Inönü-Wigner contraction of Poincaré $\oplus$ $\mathfrak{u}$(1)

Metric = (-+++), complex $i$'s are ignored.

_____________________________________________________________________

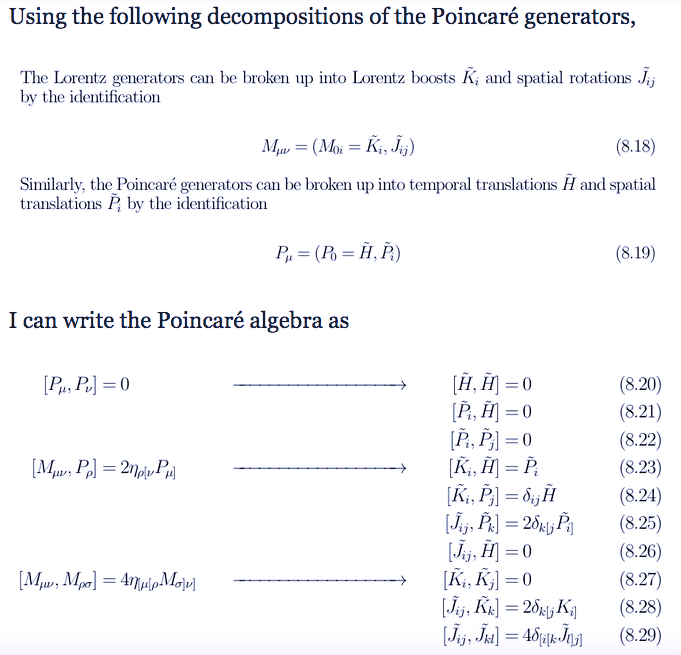
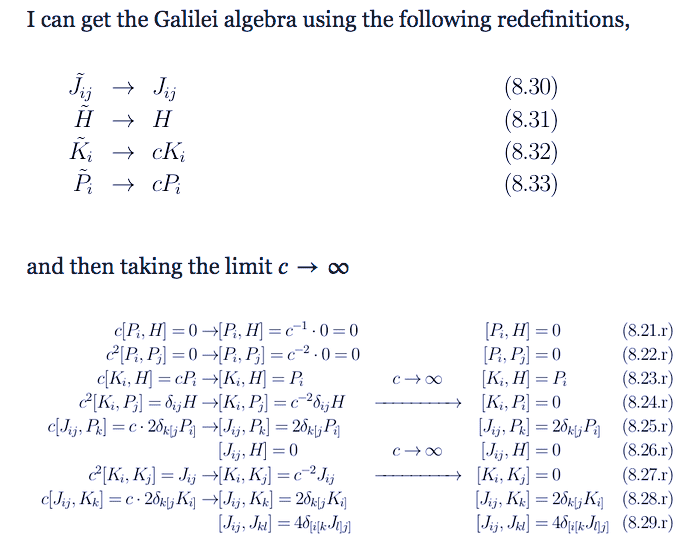
The centrally extended Galilei algebra is the Bargmann algebra.

One cannot go straight from Poincaré to Bargmann though

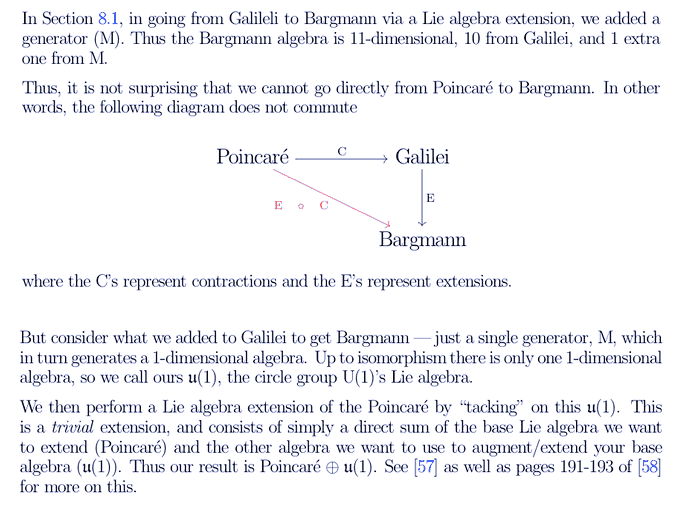
I am trying to work out the new contraction C ' so that the following diagram commutes

where after making those redefinitions and taking the limit c to infinity, I get

_________________________________________________________________

___________________________________________________

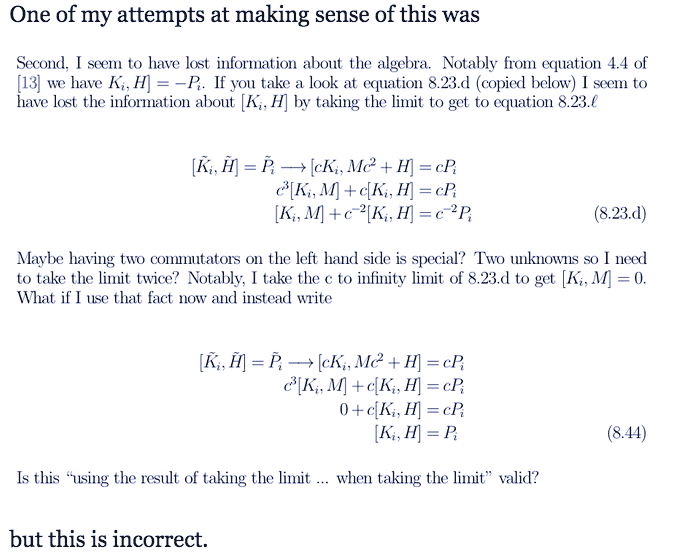
The correct answer is given in the following reference

E. Bergshoeff, J. Gomis, and P. Salgado-Rebolledo, “Non-relativistic limits and three-dimensional coadjoint Poincare gravity,” arXiv:2001.11790[hep-th].

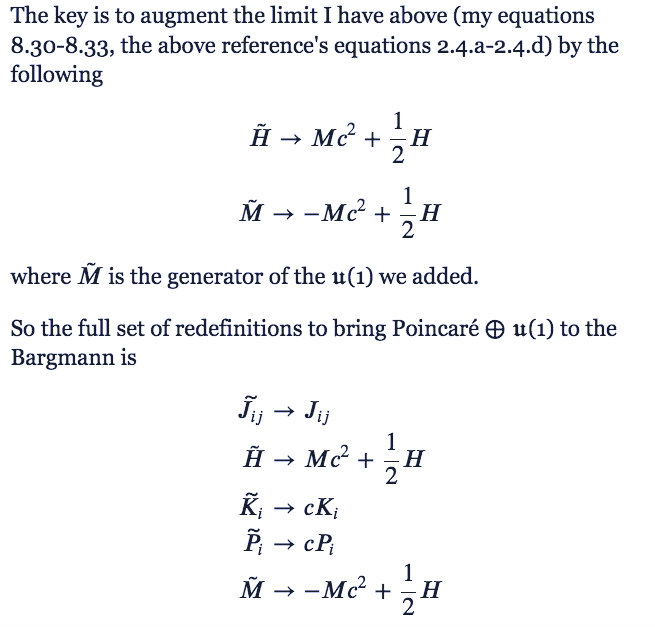
My confused lies in where I implement this redefinition of tilde M$. I was under the impression that this trivial extension of Poincaré did not alter the algebra. Since tilde M is in the center, it commutes with all the normal Poincaré generators and doesn't add any information.

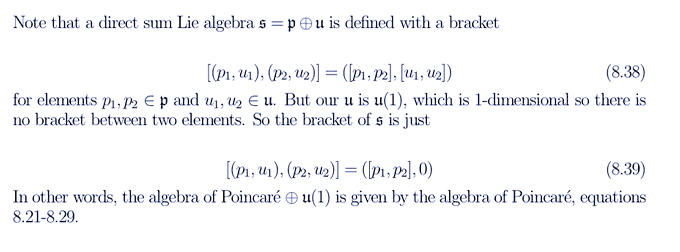
This is clearly false. The algebra is 11-dimensional now after all, not 10-dimensional.

Can anyone give me some instruction on how I implement the redefinition

\tilde{M} \rightarrow -Mc^2 + \frac{1}{2} H

in the Poincaré $\oplus$ $\mathfrak{u}$(1) Lie algebra?

 

[BigJDubs]

The answer is that the redefinition should be applied to the Poincaré \oplus \mathfrak{u}(1) Lie algebra, which can be written as:P_a, M_{ab}, J_a, H, \tilde{M}. The generator $\tilde{M}$ is then replaced with the new generator $-Mc^2 + \frac{1}{2} H$.