- #1
R3ap3r42
- 30
- 3
- Homework Statement
- Another exercise on Lorentz - particle decay
- Relevant Equations
- Lorentz transformations, conservation of momentum and energy
A π+ meson is an elementary particle with a mean lifetime, defined in its rest frame, of τ = 2.60×10−8 s. The meson decays to a muon (µ+) and a neutrino (νµ) via the reaction π+ → µ+ + νµ. A π+ traveling in the laboratory decays so that the µ+ travels in the same direction as the original π+ and the νµ travels in the opposite direction.
a) At what speed must a typical π+ be traveling if it is to move a distance of 20 m in the laboratory from the point where it is produced to the point where it decays?
See my work below. The expected result is 0.932 but I am getting 0.9916,
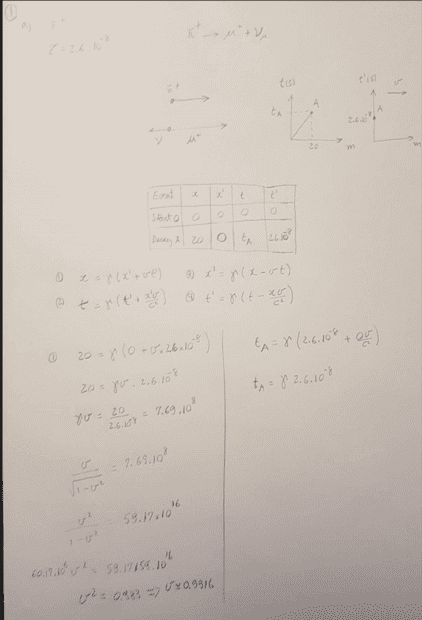
Any help will be much appreciated.
a) At what speed must a typical π+ be traveling if it is to move a distance of 20 m in the laboratory from the point where it is produced to the point where it decays?
See my work below. The expected result is 0.932 but I am getting 0.9916,
Any help will be much appreciated.