- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Paul E. Bland's book, "Rings and Their Modules".
I am focused on Section 6.1 The Jacobson Radical ... ...
I need help with the proof of Corollary 6.1.3 ...Corollary 6.1.3 (including the preceding Proposition) reads as follows:
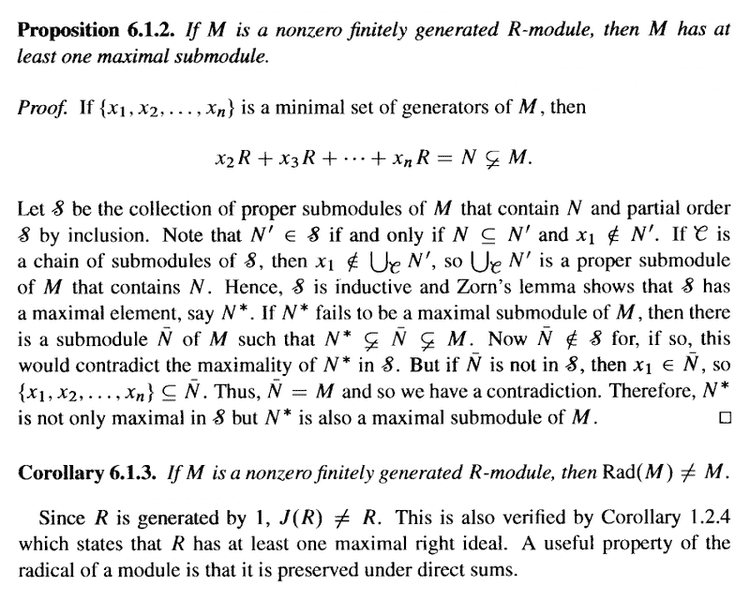
My questions are as follows:Question 1
In the proof of Corollary 6.1.3 above we read:
"... ... Since ##R## is generated by ##1, J(R) \neq R##. ... ...My question is as follows: why, given that ##R## is generated by ##1##, is it true that ##J(R) \neq R## ... ... ?
Question 2
Bland seems to argue that if we accept that ##J(R) \neq R##, then the Corollary is proved ... ... that is that
##J(R) \neq R \Longrightarrow \text{ Rad}(M) \neq M## ... ...
But ... why would this be true ...?Hope someone can help ... ...Peter
===========================================================================In order to give forum readers the notations, definitions and context of the above post, I am providing the first two pages of Chapter 6 of Bland ... ... as follows ... ... :
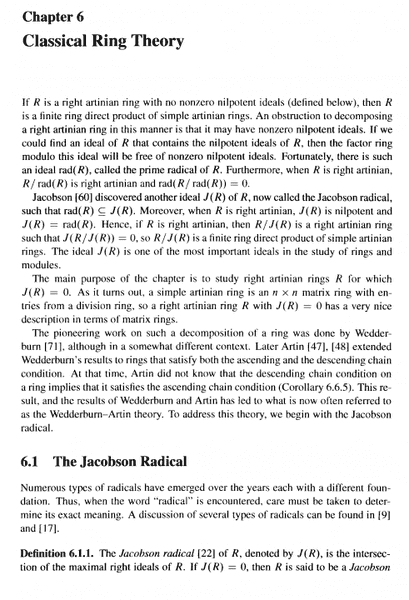
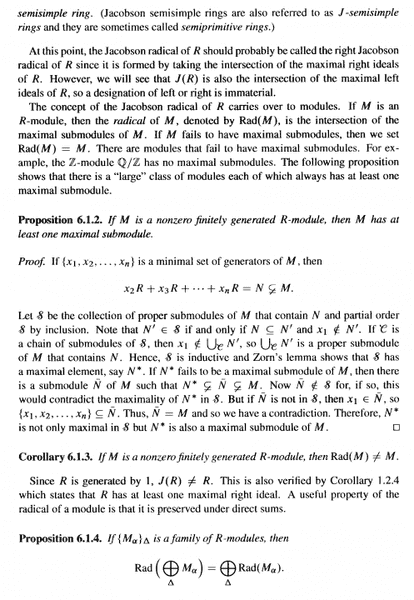
I am focused on Section 6.1 The Jacobson Radical ... ...
I need help with the proof of Corollary 6.1.3 ...Corollary 6.1.3 (including the preceding Proposition) reads as follows:
My questions are as follows:Question 1
In the proof of Corollary 6.1.3 above we read:
"... ... Since ##R## is generated by ##1, J(R) \neq R##. ... ...My question is as follows: why, given that ##R## is generated by ##1##, is it true that ##J(R) \neq R## ... ... ?
Question 2
Bland seems to argue that if we accept that ##J(R) \neq R##, then the Corollary is proved ... ... that is that
##J(R) \neq R \Longrightarrow \text{ Rad}(M) \neq M## ... ...
But ... why would this be true ...?Hope someone can help ... ...Peter
===========================================================================In order to give forum readers the notations, definitions and context of the above post, I am providing the first two pages of Chapter 6 of Bland ... ... as follows ... ... :


