Multivariable Analysis .... Directional & Partial Derivatives

[Math Amateur]

I am reading "Multidimensional Real Analysis I: Differentiation" by J. J. Duistermaat and J. A. C. Kolk ...

I am focused on Chapter 2: Differentiation ... ...

I need help with an aspect of the proof of Proposition 2.3.2 ... ...

Duistermaat and Kolk's Proposition 2.3.2 and its proof read as follows:

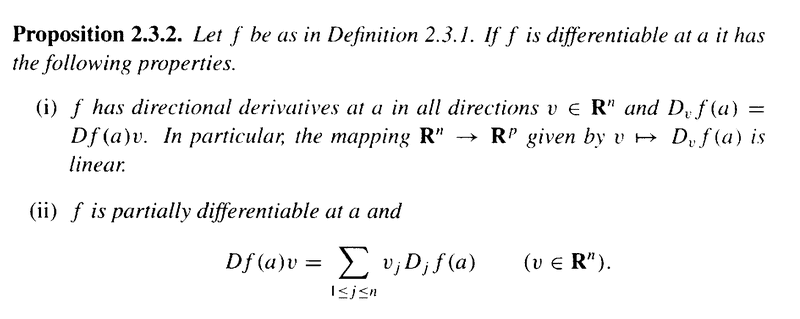
In the above proof by D&K we read the following:

" ... ... Assertion (i) follows from Formula (2.11). ... ..."Can someone please demonstrate (formally and rigorously) that this is the case ... that is that assertion (i) follows from Formula (2.11). ... ...Help will be appreciated ...

Peter==========================================================================================***NOTE***

It may help readers of the above post to have access to the start of Section "2.3: Directional and Partial Derivatives" ... in order to understand the context and notation of the post ... so I am providing the same ... as follows:

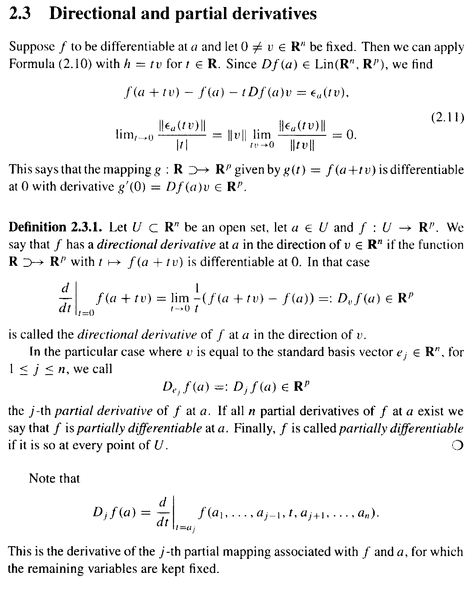
Hope that the above helps readers of the post understand the context and notation of the post ...

Peter

 

[fresh_42]

They are already the same, so I don't know what to show here. The assertion in 2.3.2 is:

• $f$ has a derivative in $a$
• in any direction $v$
• $D_v(f)(a) = \left. \dfrac{d}{dv}\right|_{x=a}f(x)$ is linear

and from equation 2.11 w have:

• $Df(a)v = Df(a).v = Df(a)(v) = D_vf(a) = D_{a;v}f = (D_af)(v)$ is the derivative in $x=a$ simply written in various different ways, depending on what is emphasized: point of evaluation, direction of change, linearity of $D$, function in $x$, etc. The introduction to this article: https://www.physicsforums.com/insights/the-pantheon-of-derivatives-i/ maybe helps a bit, and in this one (§1) I made the fun and gathered a couple of different views: https://www.physicsforums.com/insights/journey-manifold-su2mathbbc-part/ and it isn't even all of them.
• Since we have no restriction in equation 2.11 on $v$, it could be any direction, so all of them are valid.
• Derivatives are linear functions (in the argument $v$), the rest is only a different way of notation, see previous links.

 

[Math Amateur]

Thanks fresh_42 ...

Just now reflecting on your post ...

But I have say that your post is already very helpful ...

I was wondering why some texts give $D_v f(a) = Df(a) v$ and others give $D_v f(a) = D f(a) \cdot v$ ... but as you point out this is just two ways to express the same thing and $D_v f(a) = Df(a) v = D f(a) \cdot v$ ... ... (hope that's right ..) ...

Notation seems part of the difficulties in understanding differentiation of multivariable vector-valued functions ...

Peter

 

[fresh_42]

Unfortunately there is no common ground on notation here. It is always a differentiation $D$ in direction $v$ of a function $f$ evaluated at a point $a$. In the end it is a tangent at a curve at some point. That's why I wrote
$$
\left. \dfrac{d}{dv}\right|_{x=a}f(x)
$$
but this doesn't fit very well in text lines. We have an operator $D$ on a function $f$ at a point $a$ directing towards $v$. No wonder that different people arrange this differently. Except $D$ which stands for the differentiation process, all others can be variable. And even $D$ can be variable, as it is sometimes a certain derivation among many. A derivation is a linear map for which the Leibniz rule, resp. the Jacobi identity hold, which is the same, that is $D(f\cdot g) = D(f)\cdot g +f\cdot D(g)$.

The last statement was about the operator $D$ acting on functions: $f \mapsto Df$.
As differentiation, we have to evaluate it at certain point: $a \mapsto D_a(f)$.
With more than one direction as in school, we also must say in which direction, which gives us a linear map $v \mapsto D_a(f)(v)$

The first one is the most abstract and has to do with all the elaborated stuff: Lie algebras, vector bundles and similar.

The second is what is meant if people say, e.g. continuous differentiable. Continuity relates to the dependency of the location $a$. This dependency is usually neglected as people write e.g. $f\,'(x)= x^2$ and don't distinguish between the function $f\,'$ and the slope ${f\,'}|_{x=a}\,.$

The third one comes into play, if we don't have $\mathbb{R}^1$ as domain anymore, but $\mathbb{R}^n$ which obviously is more than one possible direction.

Another point we have to deal with is, that the function $f = (f_1,\ldots, f_p)$ itself has components. But this has nothing to do with the other aspects about location, direction and differentiated function considered as variable for $D$. So all these information has to be grouped around $D$.