- #1
Jolb
- 419
- 29
Penrose Diagrams -- Introduction
I'm woefully ignorant of a lot of things in GR, and now that I'm moving away from my comfortable spot in condensed matter, I'd like to know how to interpret Penrose diagrams. I'm having trouble understanding what it is they're actually showing and why they'd be useful. For example, take a look at this diagram:
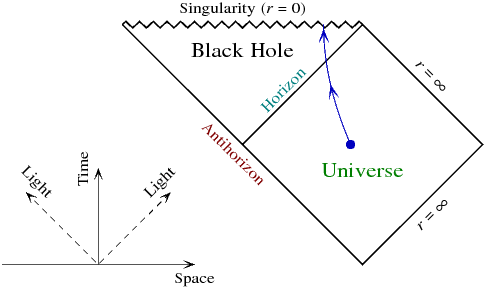
Why is it useful to squish down the geometry of spacetime so that infinity is on the edges? What significance does it have to cross from the lower diamond to the top left triangle, other than that now you're inside the event horizon?
Is the diagram showing us that the horizon is a coordinate singularity, and that's why it would be located toward the future at infinity? Is this also showing that the event horizon becomes light-like when viewed from some particular reference frame? At the point where the horizon and "anti-horizon" come together, does this indicate the black hole is born at that event?
I'm really looking for any kind of helpful introduction. I'm pretty lost and any tips or references would be greatly appreciated!
I'm woefully ignorant of a lot of things in GR, and now that I'm moving away from my comfortable spot in condensed matter, I'd like to know how to interpret Penrose diagrams. I'm having trouble understanding what it is they're actually showing and why they'd be useful. For example, take a look at this diagram:
Why is it useful to squish down the geometry of spacetime so that infinity is on the edges? What significance does it have to cross from the lower diamond to the top left triangle, other than that now you're inside the event horizon?
Is the diagram showing us that the horizon is a coordinate singularity, and that's why it would be located toward the future at infinity? Is this also showing that the event horizon becomes light-like when viewed from some particular reference frame? At the point where the horizon and "anti-horizon" come together, does this indicate the black hole is born at that event?
I'm really looking for any kind of helpful introduction. I'm pretty lost and any tips or references would be greatly appreciated!