- #1
BREAD
- 50
- 0
In the book of Modern Quantum physics by Sakurai
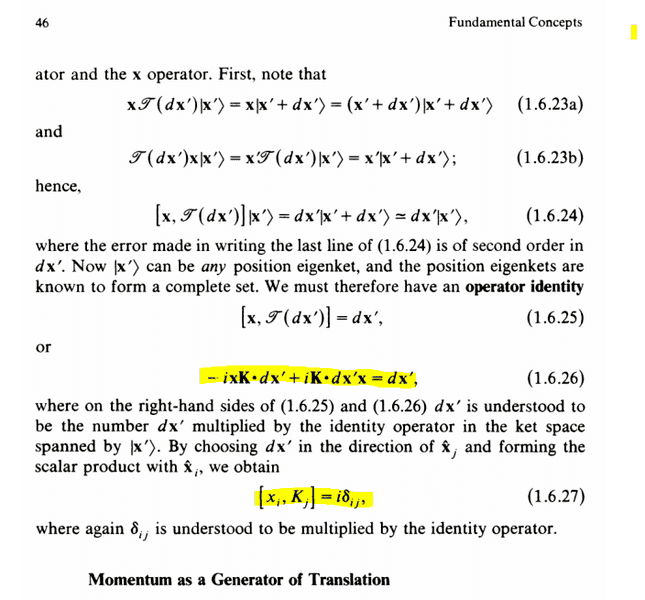
I wonder how 1.6.26 can be 1.6.27.
I wonder how 1.6.26 can be 1.6.27.
BvU said:Thanks, @PeroK. Out of curiosity: How did you know ##{\bf x K} \cdot d{\bf x'}## was to be interpreted as ##{\bf x} \left ({\bf K} \cdot d{\bf x'} \right)## and not as ##\left ({\bf x K} \right ) \cdot d{\bf x'}## ?
whyohwhy said:or I am misunderstanding what is meant by "the number dx'" which I am interpreting to mean a scalar
So this sounds like it could be right to me. But I don't get the distinction between elements belonging to Hilbert space and vectors in the ordinary sense. Looks like I have some more reading to do! Thanks so muchandresB said:In here the word vector has two meanings. dx is a vector in the ordinary sense of vector calculus(more precisely, it's a differential form).
The other meaning of the word vectors refers to the elements belonging to the Hilbert space spanned by the kets ##\left|\mathbf{x}\right\rangle## .
I recommend you to first get used to the derivation of the commutation relations with the translation operator for the 1d case (1 spatial dimension, the Hilbert space of square-integrable functions is still infinite-dimensional).whyohwhy said:So this sounds like it could be right to me. But I don't get the distinction between elements belonging to Hilbert space and vectors in the ordinary sense. Looks like I have some more reading to do! Thanks so much
Ah! This makes sense! But isn't mentioned in the textbook up to this point. Or at least not explicitly.andresB said:The position operator is a vector operator, loosely this means that it has components in each axis
$$\hat{\mathbf{X}}=\hat{x}\mathbf{i}+\hat{y}\mathbf{j}+\hat{z}\mathbf{k}$$
its actions on a 3d position ket is
$$\hat{\mathbf{X}}\left|\mathbf{x}\right\rangle =\left(x\mathbf{i}+y\mathbf{j}+z\mathbf{k}\right)\left|\mathbf{x}\right\rangle $$
The 3d vector notation is unusual, generally speaking, books write the action of each component individuallywhyohwhy said:Ah! This makes sense! But isn't mentioned in the textbook up to this point. Or at least not explicitly.
Modern Quantum physics is a branch of physics that studies the behavior and interactions of particles on a quantum level. It is a fundamental theory that explains the behavior of matter and energy at the smallest scales, such as atoms and subatomic particles. It is also known as quantum mechanics or quantum physics.
Sakurai is a renowned Japanese physicist who made significant contributions to the field of quantum mechanics. His full name is Jun John Sakurai, and he is best known for his textbook "Modern Quantum Mechanics," which is widely used in universities around the world.
Some key concepts in Modern Quantum physics include wave-particle duality, quantization of energy, uncertainty principle, superposition, and entanglement. These concepts help to explain the behavior of particles and their interactions on a quantum level.
Modern Quantum physics differs from classical physics in that it describes the behavior of particles in terms of probabilities rather than definite values. It also takes into account the wave-like nature of particles and allows for phenomena such as superposition and entanglement, which are not observed in classical physics.
Modern Quantum physics is important because it provides a deeper understanding of the fundamental laws of nature and helps to explain the behavior of matter and energy on a microscopic scale. It also has practical applications in various fields, such as electronics, computing, and cryptography.