Rotating bar hanging on a spring (oscillation)

[JulienB]

Homework Statement

Hi everybody! I'm trying to solve a basic problem about oscillations, but I struggle to get everything together when it comes to the differential equation...I hope someone can help me to understand it better :)

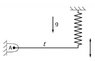
A thin bar of mass m and length l is pivotable about its hanging point A, and can oscillate with the help of a spring (with spring constant k) attached to its other end. Consider there is no deflection.
a) Put up the equation of movement and the resulting differential equation under the use of the displacement angle β.
b) What is the time period T of the system?
c) Give the amplitude A and the phase angle φ of the oscillation, if the bar is deflected at the angle β₀ at time t = 0s and has an angle velocity ψ₀.
A possible approach to the resolution of the oscillation equation is β(t) = A⋅cos(ω₀⋅t + φ).

(see attached picture)

Homework Equations

equations related to oscillation, moment of inertia of a bar, maybe torque and angular momentum

The Attempt at a Solution

Okay this is probably very wrong, but I don't want to post the problem without at least trying something:

a) I've learned how to derive the differential equation for a simple harmonic motion when the spring is horizontal, but I wonder if that still holds when it is vertical, because of the force of gravity? Maybe it is the case because "there is no deflection"? If so:

m⋅a = - k⋅x
⇔ m⋅(d²x/dt) = - k⋅x(t)
⇔ m⋅(d²x/dt) + k⋅x(t) = 0
k = ω₀²⋅m
⇒ d²x/dt + ω₀²⋅x(t) = 0

But I believes that gives me for solution x(t) = A⋅cos(ω₀⋅t + φ), right? Which is stated in the problem to the difference that it is for β(t)!

I also feel the moment of inertia of the bar should play a role, since L = I⋅ω = I⋅β'. Using the parallel axis theorem I get I = ⅓⋅m⋅l² but I don't dare to go any further because I don't really understand what I am doing.. Could someone give me a clue or briefly describe me how to tackle such problems?

Thank you very much in advance.Julien.

 

[Nathanael]

m⋅a = - k⋅x
⇔ m⋅(d²x/dt) = - k⋅x(t)
⇔ m⋅(d²x/dt) + k⋅x(t) = 0
k = ω₀²⋅m
⇒ d²x/dt + ω₀²⋅x(t) = 0

But I believes that gives me for solution x(t) = A⋅cos(ω₀⋅t + φ), right? Which is stated in the problem to the difference that it is for β(t)!

What does x represent? Aren't you looking for a differential equation for β?

Since β is the angle the rod about point A, you will want to consider the net torque about that point.

 

[haruspex]

I assume "no deflection" means that the equilibrium position is with the rod horizontal. So you need to define whether x is the spring extension or the extension relative to the equilibrium position; then figure out the relationship to the deflection angle.

 

[JulienB]

@Nathanael @haruspex Hi and thank you for your answers.

The net torque about point A is:

Στ = F_g⋅l/2 - F_s⋅l = ½⋅m⋅g⋅l + k⋅x⋅l

I am uncertain how I should define x: the most logical to me would be to say that x=0 describes the equilibrium position of the system, because I am searching an expression for β = sin^-1(x/l).

But then when I consider the equilibrium position I run into a problem: x=0 ⇔ F_s = 0 but I know it is not true (mg + kx = 0 would be true with x defined as the equilibrium position of the spring alone).

I can see the problem is in my definition of the restoring force and of x, but I can't seem to find a coherent/consistent way around it.

Also, the restoring force is changing constantly so the equalities I can obtain for Στ= 0 or ΣF = 0 don't seem to hold when there is an oscillation.Julien.

 

[haruspex]

@Nathanael @haruspex Hi and thank you for your answers.

The net torque about point A is:

Στ = F_g⋅l/2 - F_s⋅l = ½⋅m⋅g⋅l + k⋅x⋅l

I am uncertain how I should define x: the most logical to me would be to say that x=0 describes the equilibrium position of the system, because I am searching an expression for β = sin^-1(x/l).

But then when I consider the equilibrium position I run into a problem: x=0 ⇔ F_s = 0 but I know it is not true (mg + kx = 0 would be true with x defined as the equilibrium position of the spring alone).

I can see the problem is in my definition of the restoring force and of x, but I can't seem to find a coherent/consistent way around it.

Also, the restoring force is changing constantly so the equalities I can obtain for Στ= 0 or ΣF = 0 don't seem to hold when there is an oscillation.Julien.

So let the equilibrium extension be x₀ and x be the extension relative to that at some point.
What equation can you write relating x₀ to mg etc?
What equation for net torque and angular acceleration when at relative extension x?

 

[JulienB]

@haruspex Thank you for your answer. So when the bar is at point x₀, we can say that ΣF = 0 ⇔ mg = -kx₀
The net torque I believe is Στ = ½mgl + kxl, therefore the angular acceleration is α = Στ/I = (½mgl + kxl)/(⅓ml²) = (-3/2⋅kx₀ + kx)/ml when substituting mg by -kx₀.

Does that make sense before I go further?[/sub]

 

[haruspex]

the bar is at point x0, we can say that ΣF = 0 ⇔ mg = -kx₀

You are overlooking the support from the hinge. Use moments here.

Στ = ½mgl + kxl

I defined x as the relative extension, so the total extension would be x+x₀. I think you'll find that works out better.

 

[JulienB]

@haruspex Thank you again for your answer, it really helps me to make progress with this concept (hopefully).

Indeed I forgot to take in consideration the force of the support. I try again with the torque as starting point:

Στ = 0 ⇔ F_g⋅½⋅l - F_s⋅l = 0
⇔ ½⋅m⋅g⋅l + k⋅x⋅l = 0 (here I consider as I think you said x being the difference between the equilibrium position of the spring with charge and without charge)

Then I can define my angular acceleration:
α = Στ/I = [(3/2)⋅m⋅g + 3⋅k⋅x]/m⋅l

I also know that α = dω/dt = d²β/dt
⇒ d²β/dt + 3g/2l + 3k⋅x/ml = 0

Okay that already looks a little better, though I'm not sure what to do next. I could also say:
α = d²β/dt = -A⋅ω₀²⋅cos(ω₀⋅t + φ)
⇔ d²β/dt + A⋅(k/m)⋅cos(√(k/m)⋅t + φ) = 0

What do you think? Am I going in the right direction?Julien

 

[JulienB]

One of the guys studying with me just explained me that setting up x as the equilibrium position of the spring WITH the bar suspended on it makes possible to come up with an equation of motion without g! Is that true, and if so is that "always" the case with such problems? I'm preparing an exam, that's why I'm curious about general methods to solve oscillation problems.

 

[JulienB]

I attached a drawing I did from what I understood about the (non)role of the force of gravity in such oscillations. Can you tell me if that's in general the right way to consider the issue?

 

[haruspex]

One of the guys studying with me just explained me that setting up x as the equilibrium position of the spring WITH the bar suspended on it makes possible to come up with an equation of motion without g! Is that true, and if so is that "always" the case with such problems? I'm preparing an exam, that's why I'm curious about general methods to solve oscillation problems.

Yes, that's what I was trying to lead you towards but you seem to have misunderstood something.
In post #6, your equilibrium equation was nearly right, but you missed the factor 1/2 on the mg term. In post #8 you corrected that but reverted to x instead of x₀. x₀ is the extension in the equilibrium position.

In your angular acceleration equation in post #8, you have used x as though it is the total extension. Since it is only the extra extension, you need x₀+x there. When you get that right, you should see g and x₀ disappear.

 

[JulienB]

@haruspex Aha that's interesting, I rewrite the whole development:

Στ = 0 ⇔ ½⋅m⋅g = -k⋅x₀
⇒ α = [(3/2)⋅m⋅g + 3⋅k⋅(x+x₀)]/ml² = 3⋅k⋅x/m⋅l²

Then I can rewrite that equality in the form:

d²β/dt - 3⋅k⋅x/ml² = 0

Is that the equation of movement relative to β we are asked to find? Lol I'm not even sure what the question is anymore!

I could substitute d²β/dt by -A⋅ω₀²⋅cos(ω₀⋅t + φ), but I think that β(t) should be the solution to the differential equation, right? However substituting ω₀ by √(k/m) then leads me to:

-A⋅cos(√(k/m)⋅t + φ) - 3⋅x/l² = 0Julien.

 

[haruspex]

⇒ α = [(3/2)⋅m⋅g + 3⋅k⋅(x+x0)]/ml[SUP]2[/SUP] = 3⋅k⋅x/m⋅l[SUP]2[/SUP]

Then I can rewrite that equality in the form:

[B]d2β/dt - 3⋅k⋅x/ml[SUP]2[/SUP] = 0[/B]

You've missed cancelling an l, producing a dimensionally inconsistent equation. You had that right previously. The torque from the spring force needs a factor l.

In the differential equation, you need dt[SUP]2[/SUP] below the line, and you need to replace the x with a reference to beta.

 

[JulienB]

@haruspex Thank you for your answer and sorry for those mistakes, I had it right on my sheet. So the differential equation is now:

d²β/dt² - 3⋅k⋅x/(m⋅l) = 0

I can substitute k/m with ω₀², and could I say that x/l ≈ sin β ≈ β or is that a crime? We do it often in class when the angle β is very small, which seems to be the case here.

The resulting equation would then be:

d²β/dt² - 3⋅β⋅ω₀² = 0

I fear the worse as I just realized the triangle isn't even rectangle...

 

[haruspex]

@haruspex Thank you for your answer and sorry for those mistakes, I had it right on my sheet. So the differential equation is now:

d²β/dt² - 3⋅k⋅x/(m⋅l) = 0

I can substitute k/m with ω₀², and could I say that x/l ≈ sin β ≈ β or is that a crime? We do it often in class when the angle β is very small, which seems to be the case here.

The resulting equation would then be:

d²β/dt² - 3⋅β⋅ω₀² = 0

I fear the worse as I just realized the triangle isn't even rectangle...

That all looks fine, except that it is a bit misleading to use ω₀² for k/l. Omega is generally used for a frequency, and nothing here has that frequency. Better to use it for the entire coefficient of the β term in the equation, so it would be 3k/l.

 

[JulienB]

@haruspex thank you again for all your help, I really appreciate it. Yeah I realize the frequency is not always √(k/m) (is that only in case of simple harmonic motion?).

For question b), can I calculate the time period by using the formula T = 2π/ω₀? When I input β(t) = A⋅cos(ω₀⋅t + φ) in my equation of motion, I can solve for ω₀ but I get a complex number (ω₀ = i√(3k/m)). Is that normal?

Julien.

 

[haruspex]

@haruspex thank you again for all your help, I really appreciate it. Yeah I realize the frequency is not always √(k/m) (is that only in case of simple harmonic motion?).

For question b), can I calculate the time period by using the formula T = 2π/ω₀? When I input β(t) = A⋅cos(ω₀⋅t + φ) in my equation of motion, I can solve for ω₀ but I get a complex number (ω₀ = i√(3k/m)). Is that normal?

Julien.

When I wrote post #13, advising you to express x in terms of beta, I realized you needed to get the sign right. But this morning I forgot about that, and indeed you have the sign wrong. You are measuring x as an extension, so it increases as the rod descends, but beta decreases that way.

 

[JulienB]

@haruspex Aaah oh my god this is full of traps! In future situations I should probably define x with the same sign as β then.. Okay then I get a period T=2π√(m/3k). I guess (hope) that is correct.

For the last question, I imagine I have to solve β₀ = A⋅cos(φ) and ψ₀ = -A⋅φ²⋅cos(φ) for A and φ, right?

Thank you for your answer.Julien.

 

[haruspex]

@haruspex Aaah oh my god this is full of traps! In future situations I should probably define x with the same sign as β then.. Okay then I get a period T=2π√(m/3k). I guess (hope) that is correct.

For the last question, I imagine I have to solve β₀ = A⋅cos(φ) and ψ₀ = -A⋅φ²⋅cos(φ) for A and φ, right?

Thank you for your answer.Julien.

Your first equation is right (β₀) but there are at least two errors in the other. Please post your working.

With regard to getting the sign right, remember that the standard form of the SHM equation is $\ddot x+\omega^2x=0$. Thus, when x is positive the acceleration is negative, and vice versa. It is this that results in oscillation. If we change it to a minus sign then the system is unstable, accelerating away to infinity. The solution to the equation will be cosh, not cos.

 

[JulienB]

@haruspex Okay I've redone it with a friend and came up with those results:

β₀ = A⋅cos(φ)
ψ₀ = -A⋅ω₀⋅sin(φ)
I think ω₀ is the same as ψ₀, right? It's the angle velocity?

That takes me to:

A⋅sin(φ) = -1
⇒ φ = -tan^-1(1/β₀)
and
A = β₀⋅cos^-1(1/β₀)

Hopefully that makes sense. Thanks for your help.Julien.

 

[haruspex]

I think ω₀ is the same as ψ₀, right? It's the angle velocity?

No. It's a common mistake when dealing with oscillating angles to confuse the state 'angle' with the physical angle. The angle inside the trig function is not a physical angle. It only represents the state of the system in relation to its cycle. As that goes from 0 to 2π, the system goes through one cycle. If the entity that is oscillating is an angle, then that angle goes through its complete range values (usually much less than 2π), returning to its starting value.
In this case, your confusion is similar, confusing the rates of change of these two angles. ω₀ is the frequency with which the system oscillates, i.e. the rate of change of the state angle, while ψ₀ is the rate of change of the physical angle at time 0.

 

[JulienB]

@haruspex Oh sorry I guess that was a desperate move :) I try something else:

I rewrite β₀ = A⋅cos(φ) as A = β₀/cos(φ) and input that in my second expression to solve for φ. I get:

-β₀⋅√(3k/m)⋅tan(φ) = ψ₀

which transforms into an elegant φ = tan^-1(-ψ₀⋅√m / (β₀⋅√(3k))

Is that something? Can I check the result somehow in such situations? I prefer to wait for the next answer before solving for A as it looks very messy...

Thanks a lot for your help, I appreciate it.Julien.

 

[JulienB]

Well actually I could quickly solve for A using trig identities so I'll just post it although I am not sure about φ:

A = β₀/(cos(tan^-1(φ)) = √(β₀² + (ψ₀/ω₀)²)

Not too bad looking, but is that right? Maybe I should go back to my art studies

 

[haruspex]

Well actually I could quickly solve for A using trig identities so I'll just post it although I am not sure about φ:

A = β₀/(cos(tan^-1(φ)) = √(β₀² + (ψ₀/ω₀)²)

Not too bad looking, but is that right? Maybe I should go back to my art studies

Your expression for phi in post #22 looks right, but there is an easier way to find A. The initial conditions give you one equation with sinφ and another with cosφ. What trig relationship allows you to eliminate φ in one step?

 

[JulienB]

@haruspex Mm I'm still searching, but I can't think of anything that doesn't cancel A out as well But I will give it some thought (and research) to see what I can find. Thank you

 

[JulienB]

Is that it? I write both equations with sin²(Φ) and cos²(Φ) on the left side and add them up to get 1. On the right side I get the same result as post #23 then, but it is indeed much easier. Thanks a lot!Julien.

 

[haruspex]

Is that it? I write both equations with sin²(Φ) and cos²(Φ) on the left side and add them up to get 1. On the right side I get the same result as post #23 then, but it is indeed much easier. Thanks a lot!Julien.

That is indeed it.