- #1
- 2,116
- 2,691
I am reading the A First Book of Quantum Field Theory. I have reached the chapter of renormalization, where the authors describe how the infinities of the self-energy diagrams can be corrected. They have also discussed later how the infrared and ultraviolet divergences are corrected. Just before this chapter, they have discussed the general EM vertex, ##\Gamma## and the EM form factors.
After reading all these, two things are not getting clear to me. The first one is trivial, but the second one is more important. I should have written what the authors have said, but that is too long, so I have attached some scanned pages of the book.
The main Feynman diagram that will be used is this one:
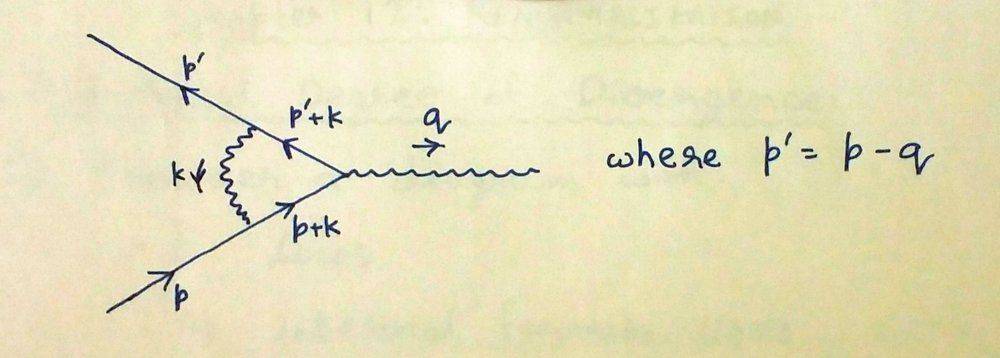
Fig. 1
Here are the questions.
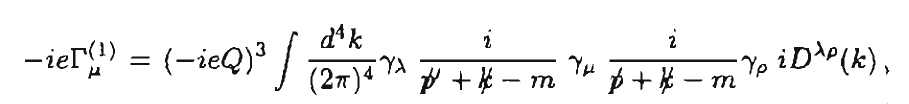
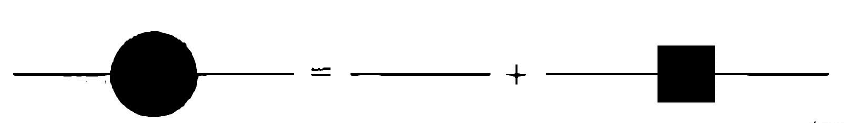
After reading all these, two things are not getting clear to me. The first one is trivial, but the second one is more important. I should have written what the authors have said, but that is too long, so I have attached some scanned pages of the book.
The main Feynman diagram that will be used is this one:
Fig. 1
Here are the questions.
1. The authors have used the terms "2-point function", "3-point function" and "2-point amplitude" in a number of places. What do these "points" mean? For example, if you refer to the attached pdf, on page 2, the authors write, "Let us first draw the total 2-point amplitude as the sum of the tree-level and the rest." Sometime later, they write, "Let us first evaluate the 1-loop corrections to the 2-point function for the fermions, i.e., the self-energy of the fermions." What does 2-point mean here?
2. Consider Fig. 1. The vertex function for the above diagram is:
Eqn. 1
where ##eQ## is the charge of the particle, and the superscript on ##\Gamma## denotes the number of loops.
The authors have said, "Let us first draw the total 2-point amplitude as the sum of the tree-level and the rest." And they have given the following diagram:
Fig. 2
The undecorated line on the RHS is the tree level diagram.
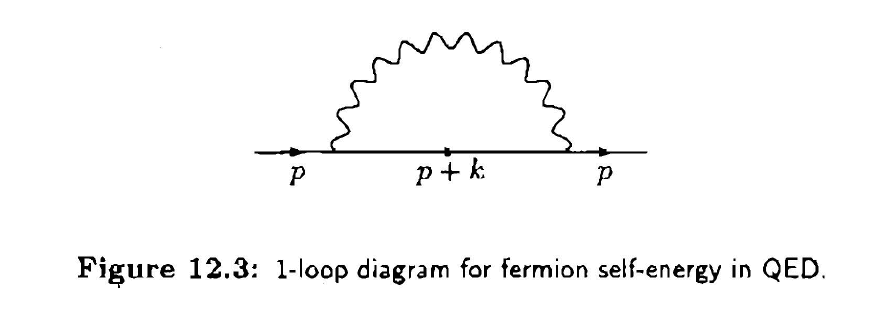
Next, consider this diagram (from the attached pdf):
Fig. 3
The contribution of this diagram can be written as: $$-i\Sigma^{(1)} (p) \ = \ (-ieQ)^2 \int \frac{d^4 k}{(2\pi)^4} \gamma_\mu \dfrac{i}{\not\! p + \not\! k - m} \ \gamma_\nu \ i D^{\mu \nu} (k), \quad \text{...Eqn. 2}$$ where ##iD^{\mu\nu}## is the photon propagator.
After some manipulations, what comes out is, $$q^\mu \Gamma_\mu ^{(1)} (p,p') \ = \ Q \left[ \left(-\Sigma^{(1)}(p)\right) - \left(-\Sigma^{(1)}(p')\right) \right] \quad \text{...Eqn. 3}$$ Then they wrote, "Adding this (Eqn. 3) to the tree level relation gives Eq. (12.13) up to 1-loop contributions." (I have highlighted this line in the pdf.) This is Eq. 12.13, the Ward identity: $$q^\mu \Gamma_\mu (p, \ p - q) \ = \ Q \left[S_F^{-1}(p) - S_F^{-1} (p - q)\right]$$ where ##S_F## is the fermionic propagator.
I just couldn't understand what they are saying above. What is "tree-level relation"? This one? $$q^\mu \Gamma_\mu ^{(0)} (p, \ p - q) \ = \ Q \left[S_F^{-1}(p) - S_F^{-1} (p - q)\right]^{(0)}$$ What does this mean in terms of Feynman diagrams?
Attachments
Last edited: