- #1
patrykh18
- 32
- 2
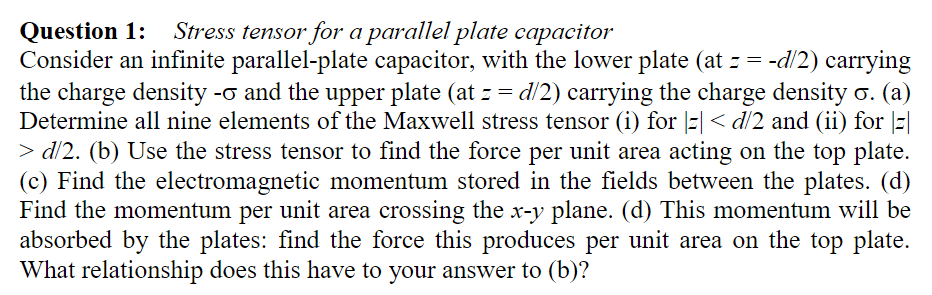
- Homework Statement
- Given infinitely large capacitors both with charge density sigma and minus sigma use the stress tensor to find the force on each.
- Relevant Equations
- None needed
The question is partially taken from Griffith's book. I am confused about the physical meaning of momentum in fields. I have determined the solution and found that in part d the momentum crossing the x-y plane is some value in the positive z direction. I don't however understand the physical interpretation of that. Does that mean that field transfer momentum upwards towards the positive plate? Does the momentum get absorbed by the capacitor? But then wouldn't it be going upwards? That of course makes no sense because the plates are oppositely charged and therefore they should attract.
Last edited by a moderator: