Sum of rational and irrational is irrational

[bigchaka]

Summary:: i get a proof that sum of rational and irrational is rational
which is wrong(obviously)

let a be irrational and q is rational. prove that a+q is irrational.
i already searched in the web for the correct proof but i can't seem to understand why my proof is false.
my proof:

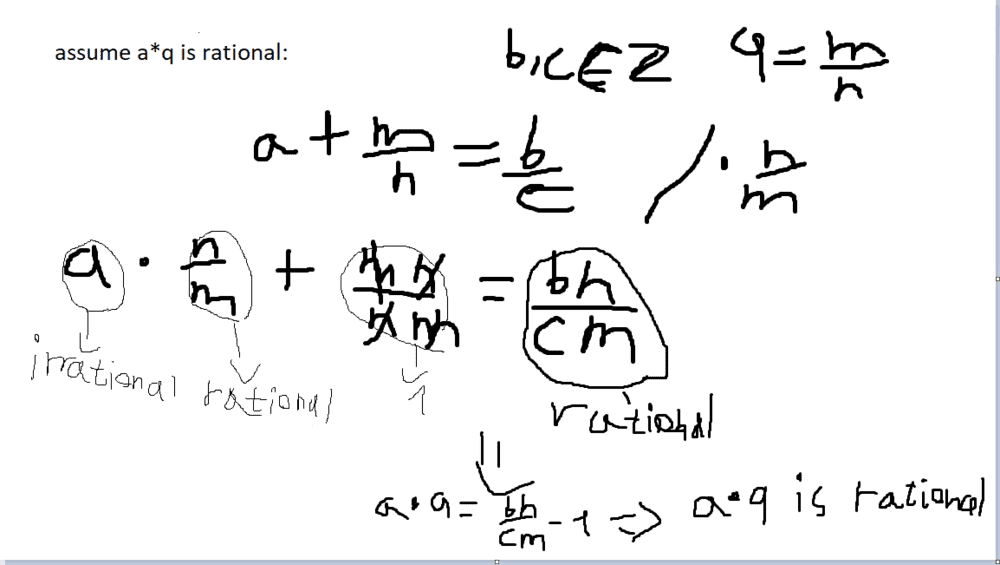
as you can see the proof i get is that the sum is rational.
can someone explain why thi

 

[fresh_42]

I don't understand why you talk about $a+q$ and write about $a\cdot q$.
Anyway. What you did is: If $a+q=q'$ then $a\in \mathbb{Q}$. This is correct. You wrote that $a$ is irrational, but you didn't use it. Quite the opposite is true: you started with an equation where $a$ is already rational.

This is the way to prove it anyway, both additive and multiplicative:

Given $a\notin \mathbb{Q}$ and $q\in \mathbb{Q}$.
Assume $a+q \in \mathbb{Q}$. Then $a+q=q'\in \mathbb{Q}$ i.e. $a=q'-q \in \mathbb{Q}$ since $\mathbb{Q}$ is closed under addition. But this contradicts $a\notin \mathbb{Q}$, hence the assumption $a+q \in \mathbb{Q}$ was wrong, i.e. $a+q \notin \mathbb{Q}$.

Multiplication goes the same way, except that you have to deal with $q=0$ as special case where the statement is wrong. The proof needs inverse elements and $0$ has no multiplicative inverse.

 

[bigchaka]

I don't understand why you talk about a+q and write about a⋅q.
Anyway. What you did is: If a+q=q′ then a∈Q. This is correct. You wrote that a is irrational, but you didn't use it. Quite the opposite is true: you started with an equation where a is already rational.

This is the way to prove it anyway, both additive and multiplicative:

Given a∉Q and q∈Q.
Assume a+q∈Q. Then a+q=q′∈Q i.e. a=q′−q∈Q since Q is closed under addition. But this contradicts a∉Q, hence the assumption a+q∈Q was wrong, i.e. a+q∉Q.

Multiplication is the same way.

sorry my bad. it was mixed inside my head i did proofs all the day..
you are right.

 

[mfb]

You have shown "if a+q is rational and q is rational then aq is rational". Which is correct, but a+q and q both being rational implies a is rational.

 

[bigchaka]

You have shown "if a+q is rational and q is rational then aq is rational". Which is correct, but a+q and q both being rational implies a is rational.

I got it, thanks!

 

[Vanadium 50]

It's really hard to follow the scribble, but in general, I would attack problems of this form with an indirect proof.

 

[PeroK]

... this is a case of "two out of three ain't possible".

A simple proof follows immediately from the sum of two rationals being rational.