- #1
- 1,254
- 141
If we restrict ourselves to a homogeneous, spatially flat LCDM universe model, the Friedman equations can be written a very simple form. Marcus posted several threads in a collaborative effort to develop the simplified approach. Here is a summary of the parts that seem the most informative, to me at least.
Part 1. The Hubble constant
Arguably the most important equation of the model is the evolution of the expansion rate over cosmological time. In other words, how the Hubble constant H has changed over time. If one knows this function, most of the other LCDM equations can be derived from it, because it fixes the expansion dynamics.
(1.1) [itex]\large H^2 - \frac{\Lambda c^2}{3} = \frac{8\pi G}{3 c^2} \rho [/itex]
Here H is the fractional expansion rate at time t, Λ is Einstein's cosmological constant, G is Newton's gravitational constant and ρ is the changing concentration of matter and radiation (at time t) expressed as a mass density. This density includes dark matter, but no 'dark energy', because Λ appears as a curvature on the left side of the equation.
As you can check, the right-hand side gives SI units of 1/s2, also the units of H2, as it is the square of an instantaneous fractional growth rate. Since Λ represents a constant curvature, its SI units would be reciprocal area 1/m2 and multiplying by c2 again gives a 1/s2 quantity. Hence both sides' units agree.
It is convenient to replace Λc2/3 with the square of a constant growth rate H∞2, representing the square of the Hubble constant of the 'infinite future', when cosmic expansion will effectively have reduced matter density to zero.
(1.2) [itex]\large H^2 - H_\infty^2 = \frac{8\pi G}{3 c^2} \rho [/itex]
Since we can measure the present value of H, labeled H0 (H-naught) and also how it has changed over time, it allows us to use Einstein's GR and his cosmological constant to determine the value of H∞. If we assume that present radiation energy is negligible compared to other forms (as is supported by observational evidence), then we can express eq. (1.2) as:
(1.3) [itex]\large H^2 - H_\infty^2 = (H_0^2 - H_\infty^2) S^3 [/itex]
H0 is the present observed rate of expansion per unit distance, which tells us that all large scale distances are presently increasing by 1/144 % per million years. This gives us a Hubble radius of 14.4 billion light years (Gly). S is the 'stretch factor' by which wavelengths of all radiation from galaxies have increased since they were emitted.[1]
The fractional distance growth rate H is declining and leveling out at the constant value H∞. The point of this equation is to understand how it is changing over time and how this effects the expansion history. But let's imagine that H remains constant (as it will in the distant future). Then, as you can check, the size a(t) of a generic distance would increase as eHt. The time interval 1/H would then be a natural time-scale of the expansion process. In that length of time distances would increase by a factor of e = 2.718. For constant H, the time 1/H can be called the "e-fold time", by analogy with "doubling time". An e-fold is like a doubling except by a factor of 2.718 instead of 2.
In the long run the universe's expansion process will be exponential at nearly the constant rate H∞, so eventually all large scale distances will undergo an e-fold expansion every 17.3 Gy. Or stated differently, all distances will eventually grow at about H∞ = 1/173 % per million years.
The 17.3 Gy 'e-fold time' is a natural time scale set by Einstein's cosmological constant. An informal study by a group of Physics-Forums contributors suggested that the 17.3 Gy time-span could be a natural timescale for the universe. For lack of an 'official name' for it, the group called it a 'zeit'. The longterm expansion rate, the reciprocal of the e-fold time, is therefore H∞ = 1 per zeit.
Here is a graph of the normalized H changing over time, expressed in zeits.
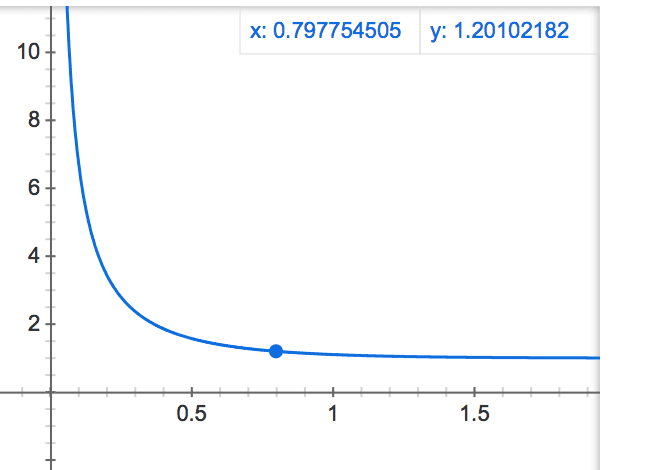
The blue dot represents our present time, 0.8 zeit and a Hubble constant of 1.2 zeit-1. The long term value of H approaches 1.
One light-zeit is 17.3 Gly in conventional terms. If the current rate H0 were to continue unchanged, distances would expand by a factor 'e' every 14.4/17.3 = 0.832 zeit. H0 = 17.3/14.4 =1.201 per zeit.[2] Our present time is 13.8/17.3 ~ 0.8 zeit.
We can easily normalize equation (1.3) to the new (zeit) scale by dividing through by H∞ (which then obviously equals 1).
(1.4) [itex]\large H^2 - 1 = (1.2012 -1) S^3 = 0.443 S^3[/itex]
or
(1.5) [itex]\large H^2 = 0.443 S^3 + 1[/itex]
This remarkably simple equation forms the basis of a surprisingly large number of modern cosmological calculations (at least for flat space), as will be discussed in Part 2.
=0=
End-notes:
[1] 'Stretch factor' S = 1/a, where a is the scale factor, as used in the LightCone calculator. S is also simply related to cosmological redshift z by S=z+1; S is the direct factor by which wavelengths have been increased, while z is a fractional change in wavelengths.
[2] The traditional unit of the Hubble constant as used by Edwin Hubble is kilometers per second per Megaparsec. From an educational p.o.v. it was an unfortunate choice, because it seems to indicate a recession speed, while it is really a fractional rate of increase of distance. It is a distance divided by a distance, all divided by time. So its natural unit is 1/time, or simply time-1.
Part 1. The Hubble constant
Arguably the most important equation of the model is the evolution of the expansion rate over cosmological time. In other words, how the Hubble constant H has changed over time. If one knows this function, most of the other LCDM equations can be derived from it, because it fixes the expansion dynamics.
(1.1) [itex]\large H^2 - \frac{\Lambda c^2}{3} = \frac{8\pi G}{3 c^2} \rho [/itex]
Here H is the fractional expansion rate at time t, Λ is Einstein's cosmological constant, G is Newton's gravitational constant and ρ is the changing concentration of matter and radiation (at time t) expressed as a mass density. This density includes dark matter, but no 'dark energy', because Λ appears as a curvature on the left side of the equation.
As you can check, the right-hand side gives SI units of 1/s2, also the units of H2, as it is the square of an instantaneous fractional growth rate. Since Λ represents a constant curvature, its SI units would be reciprocal area 1/m2 and multiplying by c2 again gives a 1/s2 quantity. Hence both sides' units agree.
It is convenient to replace Λc2/3 with the square of a constant growth rate H∞2, representing the square of the Hubble constant of the 'infinite future', when cosmic expansion will effectively have reduced matter density to zero.
(1.2) [itex]\large H^2 - H_\infty^2 = \frac{8\pi G}{3 c^2} \rho [/itex]
Since we can measure the present value of H, labeled H0 (H-naught) and also how it has changed over time, it allows us to use Einstein's GR and his cosmological constant to determine the value of H∞. If we assume that present radiation energy is negligible compared to other forms (as is supported by observational evidence), then we can express eq. (1.2) as:
(1.3) [itex]\large H^2 - H_\infty^2 = (H_0^2 - H_\infty^2) S^3 [/itex]
H0 is the present observed rate of expansion per unit distance, which tells us that all large scale distances are presently increasing by 1/144 % per million years. This gives us a Hubble radius of 14.4 billion light years (Gly). S is the 'stretch factor' by which wavelengths of all radiation from galaxies have increased since they were emitted.[1]
The fractional distance growth rate H is declining and leveling out at the constant value H∞. The point of this equation is to understand how it is changing over time and how this effects the expansion history. But let's imagine that H remains constant (as it will in the distant future). Then, as you can check, the size a(t) of a generic distance would increase as eHt. The time interval 1/H would then be a natural time-scale of the expansion process. In that length of time distances would increase by a factor of e = 2.718. For constant H, the time 1/H can be called the "e-fold time", by analogy with "doubling time". An e-fold is like a doubling except by a factor of 2.718 instead of 2.
In the long run the universe's expansion process will be exponential at nearly the constant rate H∞, so eventually all large scale distances will undergo an e-fold expansion every 17.3 Gy. Or stated differently, all distances will eventually grow at about H∞ = 1/173 % per million years.
The 17.3 Gy 'e-fold time' is a natural time scale set by Einstein's cosmological constant. An informal study by a group of Physics-Forums contributors suggested that the 17.3 Gy time-span could be a natural timescale for the universe. For lack of an 'official name' for it, the group called it a 'zeit'. The longterm expansion rate, the reciprocal of the e-fold time, is therefore H∞ = 1 per zeit.
Here is a graph of the normalized H changing over time, expressed in zeits.
The blue dot represents our present time, 0.8 zeit and a Hubble constant of 1.2 zeit-1. The long term value of H approaches 1.
One light-zeit is 17.3 Gly in conventional terms. If the current rate H0 were to continue unchanged, distances would expand by a factor 'e' every 14.4/17.3 = 0.832 zeit. H0 = 17.3/14.4 =1.201 per zeit.[2] Our present time is 13.8/17.3 ~ 0.8 zeit.
We can easily normalize equation (1.3) to the new (zeit) scale by dividing through by H∞ (which then obviously equals 1).
(1.4) [itex]\large H^2 - 1 = (1.2012 -1) S^3 = 0.443 S^3[/itex]
or
(1.5) [itex]\large H^2 = 0.443 S^3 + 1[/itex]
This remarkably simple equation forms the basis of a surprisingly large number of modern cosmological calculations (at least for flat space), as will be discussed in Part 2.
=0=
End-notes:
[1] 'Stretch factor' S = 1/a, where a is the scale factor, as used in the LightCone calculator. S is also simply related to cosmological redshift z by S=z+1; S is the direct factor by which wavelengths have been increased, while z is a fractional change in wavelengths.
[2] The traditional unit of the Hubble constant as used by Edwin Hubble is kilometers per second per Megaparsec. From an educational p.o.v. it was an unfortunate choice, because it seems to indicate a recession speed, while it is really a fractional rate of increase of distance. It is a distance divided by a distance, all divided by time. So its natural unit is 1/time, or simply time-1.
Last edited: