The Standard Metric on C^d .... Garling, Corollary 11.1.5 ....

[Math Amateur]

I am reading D. J. H. Garling's book: "A Course in Mathematical Analysis: Volume II: Metric and Topological Spaces, Functions of a Vector Variable" ... ...

I am focused on Chapter 11: Metric Spaces and Normed Spaces ... ...

I need some help with Corollary 11.1.5 ...

Corollary 11.1.5 reads as follows:

In the above proof by Garling we read the following:

" ... ... : using the inequality of the previous corollary,$d( z + w , 0 ) = \left( \sum_{ j = 1 }^d \mid z_j + w_j \mid^2 \right)^{ \frac{1}{2} } \le \left( \sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} }$$\le \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } + \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }= d(z,0) + d(w,0)$
My questions are as follows:Question 1

Can someone please explain exactly why we have:$d( z + w , 0 ) = \left( \sum_{ j = 1 }^d \mid z_j + w_j \mid^2 \right)^{ \frac{1}{2} } \le \left( \sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} }$How/why does this hold true?
Question 2

Can someone please explain exactly why we have: $\left( \sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} } \le \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } + \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }= d(z,0) + d(w,0)$How/why does this hold true?
Help will be appreciated ...

Peter========================================================================================Relevant to the above post is Proposition 11.2.3 and Corollary 11.1.4 ... so I am providing both ... as follows:

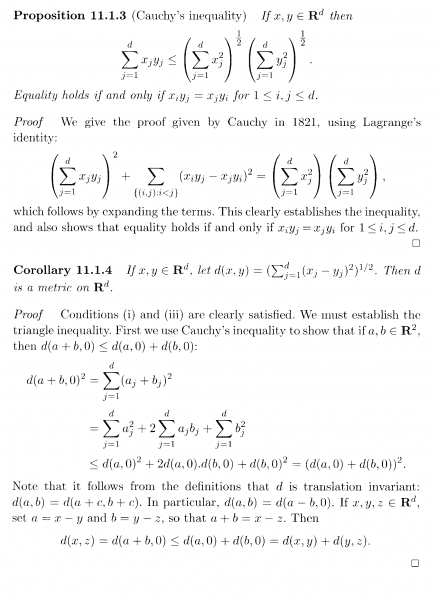
Hope the above scanned text helps readers understand the post ...

Peter

 

[fresh_42]

What do you know about the relation between $|a+b|$ and $|a|+|b|\,$?
And for the second question: square it and try to prove it by induction.

 

[andrewkirk]

Question 1

Can someone please explain exactly why we have:$d( z + w , 0 ) = \left( \sum_{ j = 1 }^d \mid z_j + w_j \mid^2 \right)^{ \frac{1}{2} } \le \left( \sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} }$How/why does this hold true?

It is sufficient to prove that, for any $j$,
$$ \mid z_j + w_j \mid^2 \le ( \mid z_j \mid + \mid w_j \mid )^2 $$
which is equivalent to
$$ \mid z_j + w_j \mid \le \mid z_j \mid + \mid w_j \mid $$

We can prove that by cases, as is often best with absolute values. Because things are squared, there are only two cases that need considering, where the signs of $z_j$ and $w_j$ are the same, and where they are different. Each case is easy to prove.

 

[andrewkirk]

The second one can be reduced to an instance of the Cauchy-Schwarz inequality by squaring both sides, then expanding the square inside the summation on the LHS, then cancelling terms that occur on both sides.

 

[Math Amateur]

Thanks Andrew and fresh_42 ...

Appreciate your help as always ...

Hmm ... should have remembered that for $z_1, z_2 \in \mathbb{C}$ we have $\mid z_1 + z_2 \mid \le \mid z_1 \mid + \mid z_2 \mid$ ...

But ... still reflecting on your posts ...

Peter

 

[Math Amateur]

Thanks Andrew and fresh_42 ...

Appreciate your help as always ...

Hmm ... should have remembers that for $z_1, z_2 \in \mathbb{C}$ we have $\mid z_1 + z_2 \mid \le \mid z_1 \mid + \mid z_2 \mid$ ...

But ... still reflecting on your posts ...

Peter

fresh_42, Andrew

Just a thought regarding Question 2 ... Garling suggests we use the previous Corollary (which is Corollary 11.1.4) ... but how do we use Corollary 11.1.4 to answer Question 2 ... ...

Peter

 

[fresh_42]

fresh_42, Andrew

Just a thought regarding Question 2 ... Garling suggests we use the previous Corollary (which is Corollary 11.1.4) ... but how do we use Corollary 11.1.4 to answer Question 2 ... ...

Peter

I only see that it gives you the equations for the $d(0,a)$ terms. The inequalities are the triangle inequality for question 1 and squaring the second should give you the one for question 2 by the help of Cauchy-Schwarz as @andrewkirk mentioned. It might be a little work to actually prove it, but it's a good exercise (you may assume Cauchy-Schwarz as given, as the Wiki link contains a proof):
$$
\left( \sum_{ j = 1}^n ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} } \le \left( \sum_{ j = 1 }^n \mid z_j \mid^2 \right)^{ \frac{1}{2} } + \left( \sum_{ j = 1 }^n \mid w_j \mid^2 \right)^{ \frac{1}{2} }
$$
Try it for $n=2,3$ and you should see how it works.

 

[Math Amateur]

Hi fresh_42, Andrew ...

... taking your advice regarding Question 2 ...

We have to show that ... :$\left( \sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} } \le \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } + \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }$ ... ... ... ... ... (1)Squaring both sides of (1) we get ...

$\sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 = \sum_{ j = 1}^d \mid z_j \mid^2 + \sum_{ j = 1}^d \mid w_j \mid^2 + 2 \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }$ ... ... ... ... ... (2)Now ... expanding the LHS of (2) we get

$\sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 = \sum_{ j = 1}^d \mid z_j \mid^2 + 2 \sum_{ j = 1}^d \mid z_j \mid \mid w_j \mid + \sum_{ j = 1}^d \mid w_j \mid^2$ ... ... ... ... ... (3)Substituting (3) into (2) we get ...:

$\sum_{ j = 1}^d \mid z_j \mid^2 + 2 \sum_{ j = 1}^d \mid z_j \mid \mid w_j \mid + \sum_{ j = 1}^d \mid w_j \mid^2 \ \le \ \sum_{ j = 1}^d \mid z_j \mid^2 + \sum_{ j = 1}^d \mid w_j \mid^2 + 2 \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }$$\Longrightarrow \ \ \sum_{ j = 1}^d \mid z_j \mid \mid w_j \mid \ \le \ \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }$ ...

... BUT ... this is just Cauchy's Inequality! ... QED ...Is the above correct ... ?

Peter

 

[andrewkirk]

Yes, that's correct. Well done.

 

[StoneTemplePython]

FWIW, note that question 2 really is triangle inequality as well.

Consider two vectors $\mathbf a$ and $\mathbf x$, where $\mathbf a$ has the magnitude of the components of $\mathbf z$ and $\mathbf x$ has the magnitude of the components of $\mathbf w$

(alternatively work directly with $\mathbf z$ and $\mathbf w$ and assume WLOG that components are real non-negative)

$\left( \sum_{ j = 1}^d ( \mid z_j \mid + \mid w_j \mid )^2 \right)^{ \frac{1}{2} } = \big \Vert \mathbf a + \mathbf x\big \Vert_2 \leq \big \Vert \mathbf a \big \Vert_2 +\big \Vert \mathbf x \big \Vert_2 = \left( \sum_{ j = 1 }^d \mid z_j \mid^2 \right)^{ \frac{1}{2} } + \left( \sum_{ j = 1 }^d \mid w_j \mid^2 \right)^{ \frac{1}{2} }$

by triangle inequality.