Angular Velocity in the Rotating systems

[WMDhamnekar]

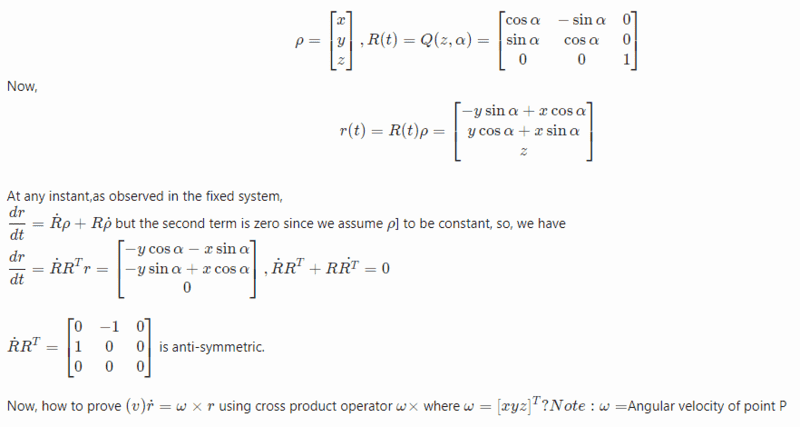
Summary: Consider a body which is rotating with constant angular velocity ω about some
axis passing through the origin. Assume the origin is fixed, and that we are sitting
in a fixed coordinate system $O_{xyz}$
If $\rho$ is a vector of constant magnitude and constant direction in the rotating system,
then its representation r in the fixed system must be a function of t.

Now how to verify $\dot{r}= \omega \times r$
My attempt:

 

[pasmith]

$\omega$ should be parallel to the axis of rotation, which here is $(0,0,1)^T$. So you need to double check your calculation of $\dot R R^T$. Remember that $\dot R = \dot\alpha \dfrac{dR}{d\alpha}$.

 

[WMDhamnekar]

$\omega$ should be parallel to the axis of rotation, which here is $(0,0,1)^T$. So you need to double check your calculation of $\dot R R^T$. Remember that $\dot R = \dot\alpha \dfrac{dR}{d\alpha}$.

I got as author said $\dot{r}(v) =\omega \times r$ So, I tagged this question ' SOLVED'

 

[pasmith]

Tour $\dot R R^T$ is correct, but needs to be multiplied by a factor of $\dor \alpha$, which is unknown. Then you have $$\dot R R^T \begin{pmatrix} x \\ y \\ z \end{pmatrix} = \dot \alpha \begin{pmatrix}-y \\ x \\ 0 \end{pmatrix}$$. Can you find $(\omega_1,\omega_2,\omega_3)$ such that $$\begin{pmatrix} \omega_1 \\ \omega_2 \\ \omega_3 \end{pmatrix} \times \begin{pmatrix} x \\ y \\ z \end{pmatrix} = \dot \alpha\begin{pmatrix} -y \\ x \\ 0\end{pmatrix}?$$

 

[WMDhamnekar]

Tour $\dot R R^T$ is correct, but needs to be multiplied by a factor of $\dor \alpha$, which is unknown. Then you have $$\dot R R^T \begin{pmatrix} x \\ y \\ z \end{pmatrix} = \dot \alpha \begin{pmatrix}-y \\ x \\ 0 \end{pmatrix}$$. Can you find $(\omega_1,\omega_2,\omega_3)$ such that $$\begin{pmatrix} \omega_1 \\ \omega_2 \\ \omega_3 \end{pmatrix} \times \begin{pmatrix} x \\ y \\ z \end{pmatrix} = \dot \alpha\begin{pmatrix} -y \\ x \\ 0\end{pmatrix}?$$

Hi,
$[ 0,0,1] \times [ -y \sin{\alpha} + x \cos{\alpha}, y\cos{\alpha} + x \sin{\alpha}, z ] = [ -y \cos{\alpha}-x\sin{\alpha}, -y\sin{\alpha}+ x\cos{\alpha}, 0 ] = \omega \times r = \dot{r}$

So, we get what author said/got.