- #1
shivam jain
- 8
- 0
Homework Statement
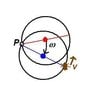
a ring of mass M and radius r lies on its side on a frictionless table.it is pivoted to table at its rim.a bug of mass m walks around the ring with speed v,starting at the pivot.what is the rotational velocity of the ring when the bug is a) halfway around b)back at the pivot
Homework Equations
initial angular momentum=final angular momentum
The Attempt at a Solution
conserving angular momentum about pivot
correct answer of a) comes like this
initial L=0
final L=2Mr^2ω-2mr(v-2rω)
then initial L=final L
i am unable to understand factor of 2mrω
it should come only when ring is rotating and translating both.but here it is fixed to pivot and only rotating.further why we are taking velocity with respect to ring not ground.please explain me this solution