Ookke
- 172
- 0
Left picture: There are two moving rails that cross at center (red dot) which itself doesn't move. The rails speeds are close to c, so that they are length contracted by factor 10 (roughly 0.995c). There are markings in rails at interval 10 length units in rails rest frame, so the interval is only 1 length unit in this frame. Markings from both rails meet the center simultaneously.
Right picture: The same from horizontal rail's rest frame. The whole vertical rail and center are moving right at speed approx. 0.995c. The vertical rail has also some velocity in y-direction downwards, but considerably less than c, about 0.1c by my approximations. In right picture, the markings of horizontal rail are at interval 10 length units (not in scale, but should illustrate the idea) because this is horizontal rails own rest frame. But the markings of vertical rail are at interval 1 (or at least close) also in this frame, which can be seen e.g. by that y-velocity is only 0.1c and markings from both rails must meet center simultaneously also in this frame.
Maybe there is no problem, but I'm curious that the vertical rail seems to be strongly length contracted also in the frame of right picture, although the vertical rail's velocity is mostly horizontal and only slightly vertical in this frame. I would expect that length contraction happens only in the direction of the motion, which would mean that vertical rail shouldn't be much length contracted in y-direction.
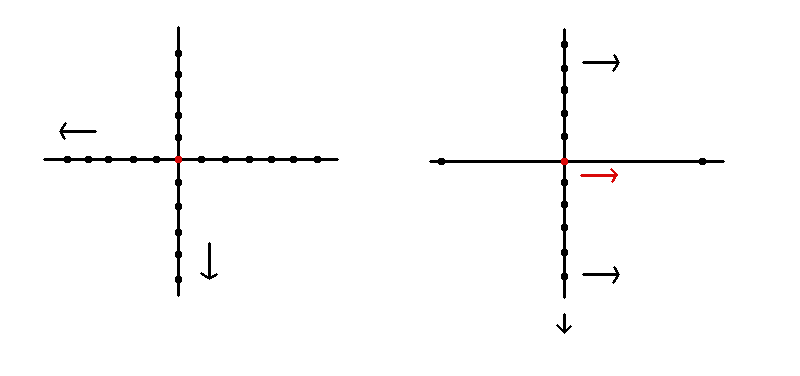
Right picture: The same from horizontal rail's rest frame. The whole vertical rail and center are moving right at speed approx. 0.995c. The vertical rail has also some velocity in y-direction downwards, but considerably less than c, about 0.1c by my approximations. In right picture, the markings of horizontal rail are at interval 10 length units (not in scale, but should illustrate the idea) because this is horizontal rails own rest frame. But the markings of vertical rail are at interval 1 (or at least close) also in this frame, which can be seen e.g. by that y-velocity is only 0.1c and markings from both rails must meet center simultaneously also in this frame.
Maybe there is no problem, but I'm curious that the vertical rail seems to be strongly length contracted also in the frame of right picture, although the vertical rail's velocity is mostly horizontal and only slightly vertical in this frame. I would expect that length contraction happens only in the direction of the motion, which would mean that vertical rail shouldn't be much length contracted in y-direction.