Draw and find the Length of x=t+4 , y=t2+1

[Michael_0039]

Homework Statement

Draw and find the Length of x=t+4 , y=t2+1

Relevant Equations

nil

Hi,

my try:

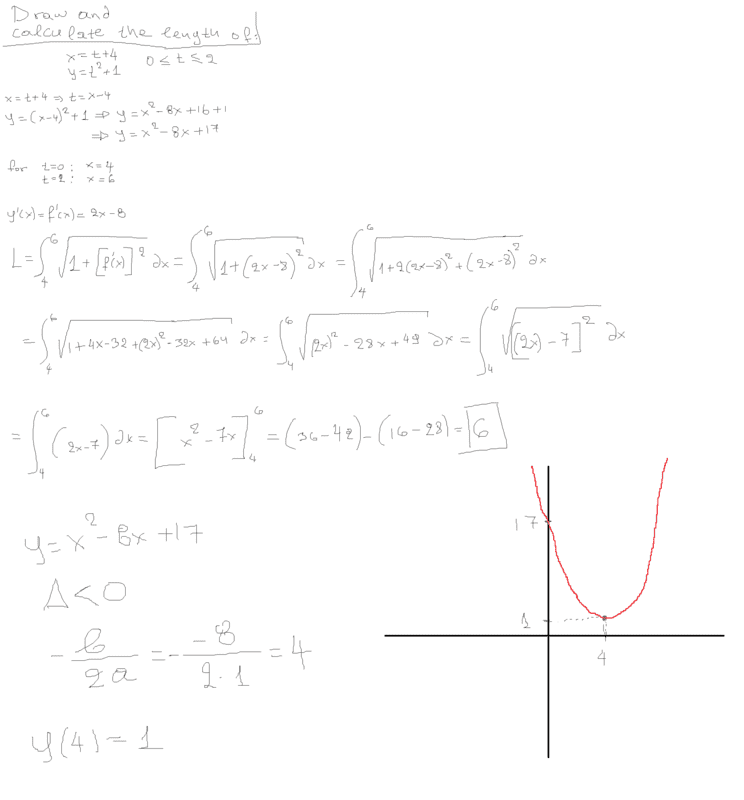
Do you agree with me?

Thanks

 

[Michael_0039]

Edit:

 

[Michael_0039]

Maybe I have to set (2x-8) = sinh(u)

https://www.wolframalpha.com/input/?i=\int_4^6\sqrt{1+((2χ-8)^2)}dχ

 

[PeroK]

Homework Statement: Draw and find the Length of x=t+4 , y=t2+1
Homework Equations: nil

Hi,

my try:

View attachment 252903
Do you agree with me?

Thanks

I don't agree. By going round in circles long enough, you finally got:

$2(x-8)^2+1 = (2x-7)^2$

Which can't be correct.

 

[PeroK]

Maybe I have to set (2x-8) = sinh(u)

https://www.wolframalpha.com/input/?i=\int_4^6\sqrt{1+((2χ-8)^2)}dχ

Yes, that looks like a good idea.

 

[PeroK]

PS note that an alternative approach was to use the parameterisation you were given:

$ds^2 = dx^2 + dy^2 = (\frac{dx}{dt}dt)^2 + (\frac{dy}{dt}dt)^2$

Hence:

$ds = \sqrt{(\frac{dx}{dt})^2 + (\frac{dy}{dt})^2} \ dt$

 

[Michael_0039]

 

[PeroK]

View attachment 252916
View attachment 252918

That looks close.

I stuck with the trig functions and got $\sqrt{17} + \frac 1 4 \sinh^{-1} (4) \approx 4.65$