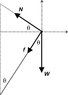
- #1
braindead101
- 162
- 0
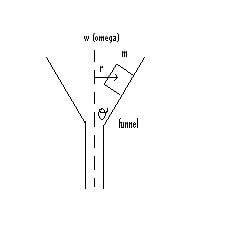
a very small cube of mass m is placed on the inside wall of a funnel. The wall of the funnel makes an angle theta with the vertical axis of rotation (dotted line). The center of the cube is a distance r from the axis of rotation. the cube is held by static friciton. The funnel is then rotated about a vertical axis at an angular speed of w (omega). it is found that the angular speed can be increased to a maximum value w(max) (omega max) at which point the cube is no longer held by the static frictional force and it flies out of the funnel. calculate w(max)
i separated everything into x and y components, but i think i did this wrong
i got equations
Fstatic - Fg cos theta = mw(max)^2r
Fn - Fg sin theta = ma
am i suppose to set a to zero? and are those even right to begin with
i separated everything into x and y components, but i think i did this wrong
i got equations
Fstatic - Fg cos theta = mw(max)^2r
Fn - Fg sin theta = ma
am i suppose to set a to zero? and are those even right to begin with
Last edited: