Multiplication of Path Classes and the Fundamental Group

[Math Amateur]

In Chapter 7 of John M. Lee's book on topological manifolds, we find the following text on composable paths and the multiplication of path classes, [f] ... ...

Lee, writes the following:

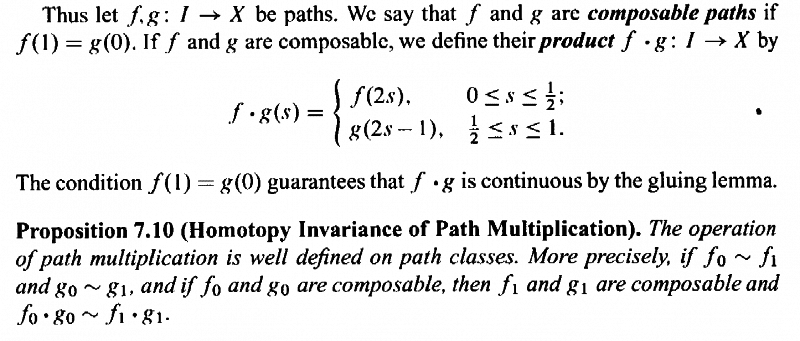In the above text, Lee defines composable paths and then defines path multiplication of path classes (not paths themselves) ...

Why does develop the theory of the fundamental group in terms of equivalence classes of paths (loops) ... why not just define a group in terms of composable paths ...?

Hope someone can clarify this matter ...

Peter

 

[Terandol]

The technical reason is simply that you don't get a group (or groupoid if you want to define it on all composable paths not just the paths with some fixed beginning=end point) because this multiplication is not associative, inverses don't exists and an identity does not exist unless you take homotopy classes. As a simple example, The path I(s)=p_0 is not the identity since for any loop \gamma at p_0, the path
( \gamma \cdot I)(s) =\begin{cases} \gamma\left(\frac{s}{2}\right) & 0\leq s\leq \frac{1}{2} \\ I(2s-1)=p_0 & \frac{1}{2}<s\leq 1 \end{cases}
is not the same as the path \gamma(s). The image of these two paths over the whole domain is the same subset of X, but a path is by definition a function and these are different functions. They are homotopic though so on equivalence classes this is an identity. Similar problems also come up for the other group properties.

If you want a more conceptual reason for considering homotopy classes, one of the main ideas in algebraic topology is to consider properties of topological spaces that are invariant under bending/stretching etc. But a path, being a function from one set to another, is completely rigid and even moving just one point the slightest bit yields a different path. Since we are trying to study only those properties which are invariant under bending/stretching etc., this means paths really aren't the appropriate thing to be concerned with and the right way to capture the idea of a path but only up to bending or stretching it is via homotopy classes of paths.

 

[Math Amateur]

The technical reason is simply that you don't get a group (or groupoid if you want to define it on all composable paths not just the paths with some fixed beginning=end point) because this multiplication is not associative, inverses don't exists and an identity does not exist unless you take homotopy classes. As a simple example, The path I(s)=p_0 is not the identity since for any loop \gamma at p_0, the path
( \gamma \cdot I)(s) =\begin{cases} \gamma\left(\frac{s}{2}\right) & 0\leq s\leq \frac{1}{2} \\ I(2s-1)=p_0 & \frac{1}{2}<s\leq 1 \end{cases}
is not the same as the path \gamma(s). The image of these two paths over the whole domain is the same subset of X, but a path is by definition a function and these are different functions. They are homotopic though so on equivalence classes this is an identity. Similar problems also come up for the other group properties.

If you want a more conceptual reason for considering homotopy classes, one of the main ideas in algebraic topology is to consider properties of topological spaces that are invariant under bending/stretching etc. But a path, being a function from one set to another, is completely rigid and even moving just one point the slightest bit yields a different path. Since we are trying to study only those properties which are invariant under bending/stretching etc., this means paths really aren't the appropriate thing to be concerned with and the right way to capture the idea of a path but only up to bending or stretching it is via homotopy classes of paths.

Thanks for the help Terandol ...

Reflecting on what you have said,

Thanks again,

Peter