- #1
nycmathguy
- Homework Statement
- Algebra & Geometry
- Relevant Equations
- Geometric Proof Using Algebra
Chapter 1, Section 1.1.
Look at the picture. Question 57.
Let me see.
To show this prove, I must find the midpoint of the diagonals. The midpoint of (b, c) and (a, 0) must be the same as the midpoint of (0, 0) and
(a + b, c).
You say?
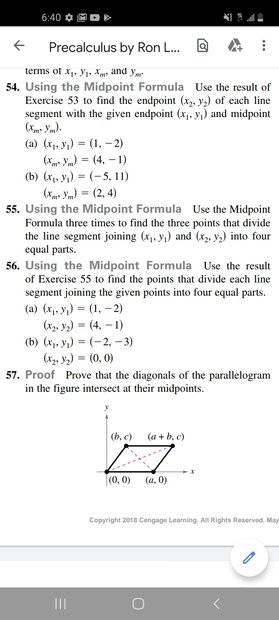
Look at the picture. Question 57.
Let me see.
To show this prove, I must find the midpoint of the diagonals. The midpoint of (b, c) and (a, 0) must be the same as the midpoint of (0, 0) and
(a + b, c).
You say?
Last edited by a moderator: