Rectangular to sphere coordinates

[EliasS]

Homework Statement

Hello,
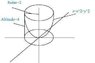
the problem ask you to pass this integral
\displaystyle\int_{-2}^{2}\int_{-\sqrt[ ]{4-x^2}}^{\sqrt[ ]{4-x^2}}\int_{x^2+y^2}^{4} x {dz}{dy}{dx}
to sphere coordinates, but I don't really know how

Homework Equations

Well, I know the basics formulas,

x=rho*sin(phi)*cos(theta)
y=rho*sin(phi)*sin(theta)
z=rho*cos(theta)

rho^2=x^2+y^2+z^2
tan theta=x/y

and all the average problems I know how to do them, but this one I can't see it

The Attempt at a Solution

The solution of the book is this, but I don't know how to get there, any clues?

\displaystyle\int_{0}^{2{\pi}}\int_{0}^{arctan(1/2)}\int_{0}^{4sec\phi} \rho^3 sin^2\phi cos\vartheta {d\rho}{d\phi}{d\vartheta}+\displaystyle\int_{0}^{2{\pi}}\int_{arctan(1/2)}^{\pi/2}\int_{0}^{cot\phi csc\phi} \rho^3sin^2\phi cos\phi{d\rho}{d\phi}{d\vartheta}

Thank you

 

[Mark44]

One of the trickier things for iterated integrals is understanding what the region over which integration takes place looks like. Do you have a good sense of what the region in your problem looks like?

 

[EliasS]

Hello, I think it's an elliptic paraboloid
like this

but from that to the sum of integrals with csc, cot etc it's where I get really confused

any hint will be great, thank you

 

[HallsofIvy]

\displaystyle\int_{-2}^{2}\int_{-\sqrt[ ]{4-x^2}}^{\sqrt[ ]{4-x^2}}\int_{x^2+y^2}^{4} x {dz}{dy}{dx}
Okay, x must vary from -2 to 2 so draw vertical lines on an xy- graph at x= -2, x= 2. Then, for each x, y goes from y= -\sqrt{4- x^2} to y= \sqrt{4- x^2}, two semi-circles. That gives the circle x^2+ y^2= 4. Finally, for each (x,y), z goes from z= x^2+ y^2 to 4. Yes, that is the region inside the paraboloid you show up to z= 4.4

Are you really asked to convert to spherical coordinates? Cylindrical coordinates would be most reasonable. In polar coordinates, the circle is just r varying from 0 to 2, \theta from 0 to 2\pi. The integral in cylindrical coordinates would be
\int_{r= 0}^2\int_{\theta= 0}^{2\pi}\int_{z= 0}^4 r^2 cos(\theta)d\theta dr dz

To change to spherical coordinates, note that the points most distant from the origin are on the circle where z= x^2+ y^2 intersects z= 4 and there distance is \sqrt{2^2+ 4^2}= 2\sqrt{4}. \rho will vary from 0 to 2\sqrt{5}. Of course, because of the circular symmetry, \theta varies from 0 to \2\pi. \phi is the hard one.

Imagine a line from (0,0,0) to (\rho, 0, \rho^2) (because of the symmetry, it enough to look at y= 0). That has slope \rho^2/\rho= \rho. Since \phi is the complement of the angle from the x-axis, \phi= cot^{-1}(\rho). \phi varies from 0 to cot^{-1}(\rho).

 

[EliasS]

Thank you HallsofIvy and Mark44, now understand everything except how to get cot\phi csc\phi in <br /> \displaystyle\int_{0}^{2{\pi}}\int_{arctan(1/2)}^{\pi/2}\int_{0}^{cot\phi csc\phi} \rho^3sin^2\phi cos\phi{d\rho}{d\phi}{d\vartheta}<br />

this integral, say in the zy plane, is something like

what I don't understand is how z=x^2+y^2 becomes cot\phi csc\phi in sphere coordinates

please this is the last thing