Secant and Tangent Angles in Circles, finding an arc length.

[Cj111]

View attachment 8161

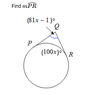
I got 138.17, but that isn't correct. I don't know how to do it, since the only way I thought, gave me the wrong answer. Can anyone help?

 

[MarkFL]

I would call point $C$ the center of the circle. So, what must the measures of $$\angle CPQ$$ and $$\angle CRQ$$ be?

 

[MarkFL]

To follow up, since $$\overline{QP}$$ and $$\overline{QR}$$ are tangent to the circle, we must have $$\angle CPQ=\angle CRQ=90^{\circ}$$. Since the sum of the interior angles of a quadrilateral is $360^{\circ}$, it follows then that:

$$100x+81x-1=180\implies x=1$$

And hence, $$\overset{\frown}{PR}=100^{\circ}$$