- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Andrew Browder's book: "Mathematical Analysis: An Introduction" ... ...
I am currently reading Chapter 12: Multilinear Algebra ... ...
I need some help in order to fully understand the proof of Theorem 12.22 on page 276 ... ...The relevant text reads as follows:
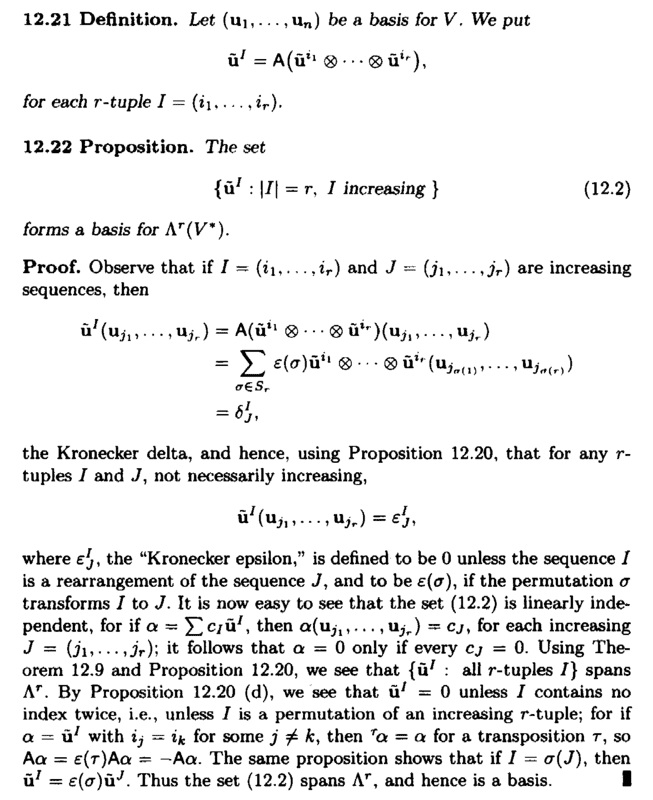
In the above proof by Browder we read the following:
" ... ... the Kronecker delta, and hence, using Proposition 12.20, that for any ##r##-tuples ##I## and ##J##, not necessarily increasing
##\tilde{u}^I ( u_{ j_1 }, \cdot \cdot \cdot u_{ j_r } ) = \varepsilon^I_J##
where ##\varepsilon^I_J##, the "Kronecker epsilon" ... ... "My question is as follows:
How is Proposition 12.20 used to show that ## \tilde{u}^I ( u_{ j_1 }, \cdot \cdot \cdot u_{ j_r } ) = \varepsilon^I_J## ... ... could someone please demonstrate the use of Proposition 12.20 to derive this result ...The above proof refers to Proposition 12.20 so I am providing the text of this proposition as follows:
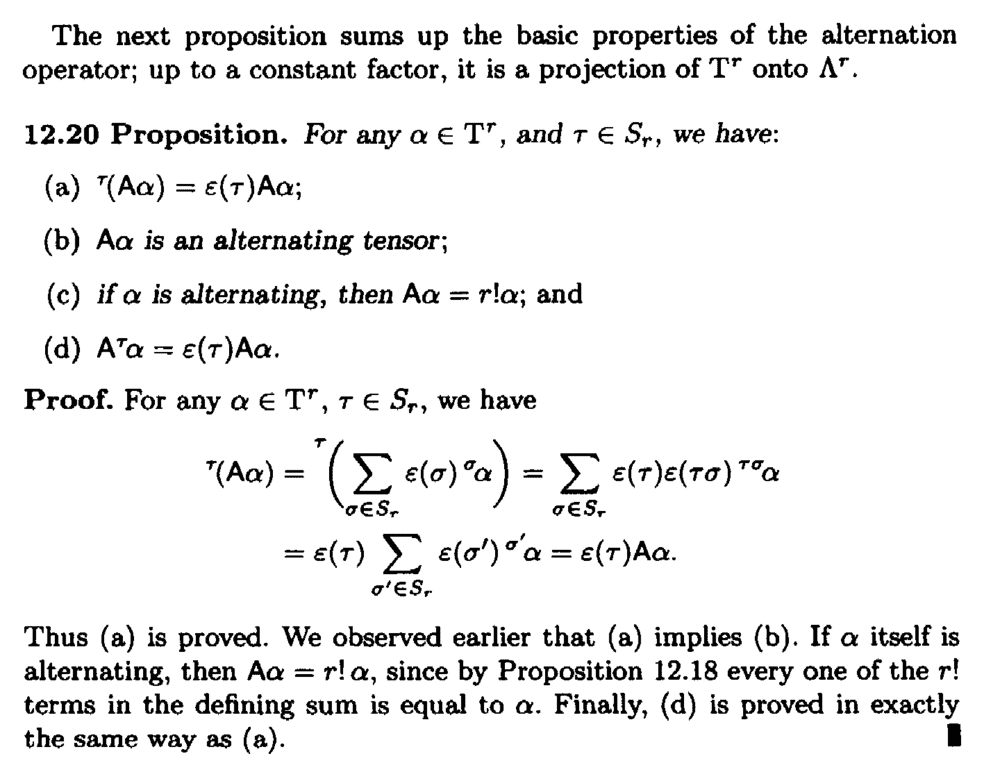
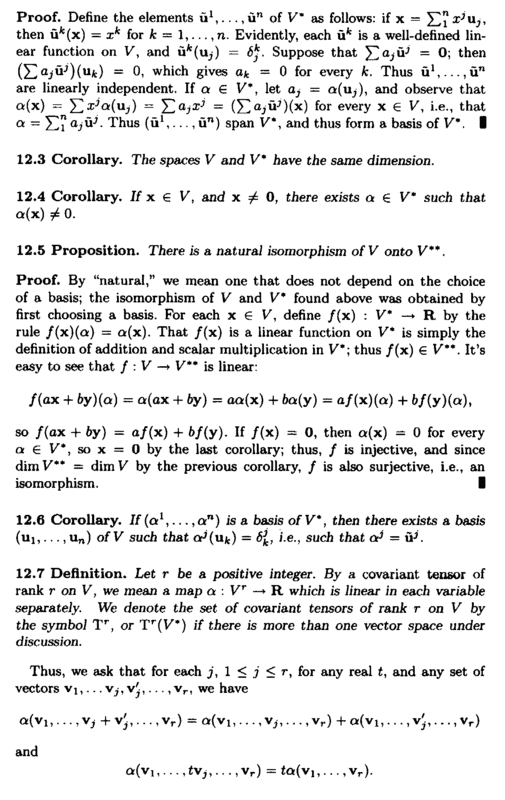
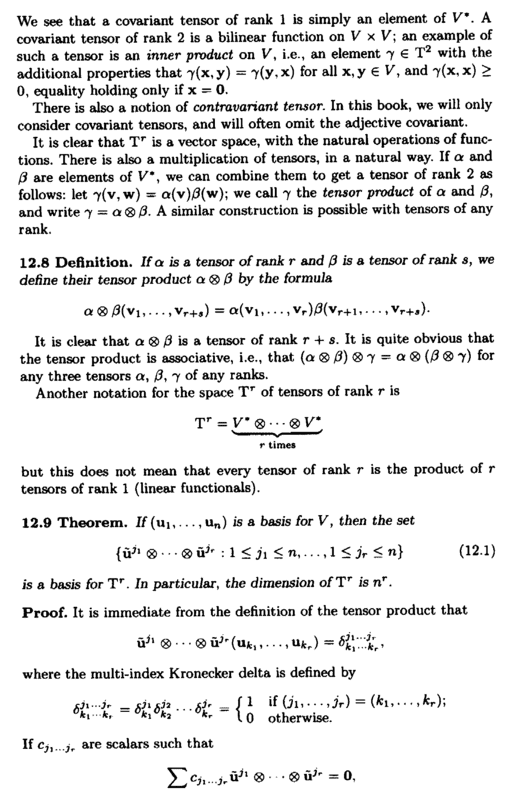
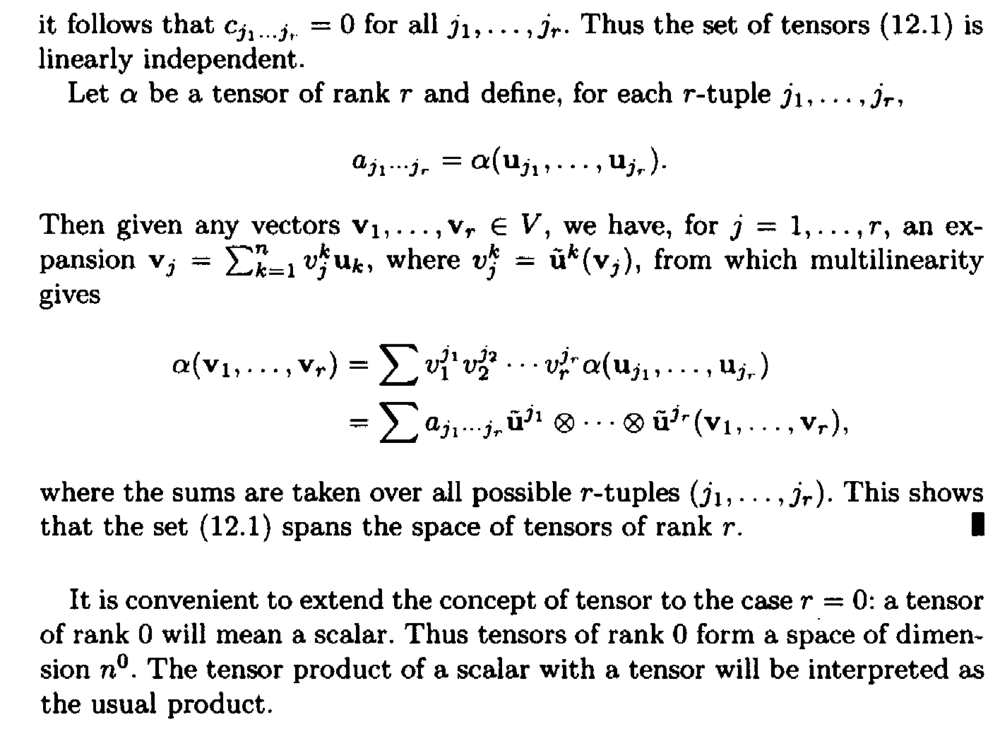
The above proof also refers (indirectly) to the basis ##( \tilde{u}^1, \cdot \cdot \cdot \tilde{u}^n )## for the dual space ##V^*## ... ... this is mentioned at the start of Section 12.1 ... so I am providing the relevant text as follows:
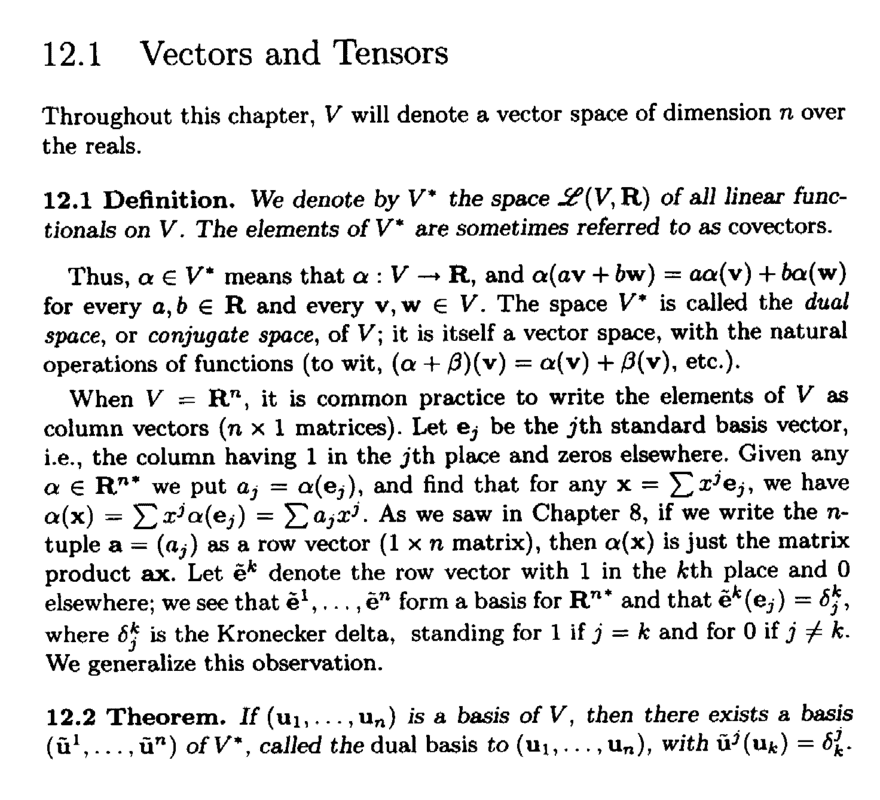
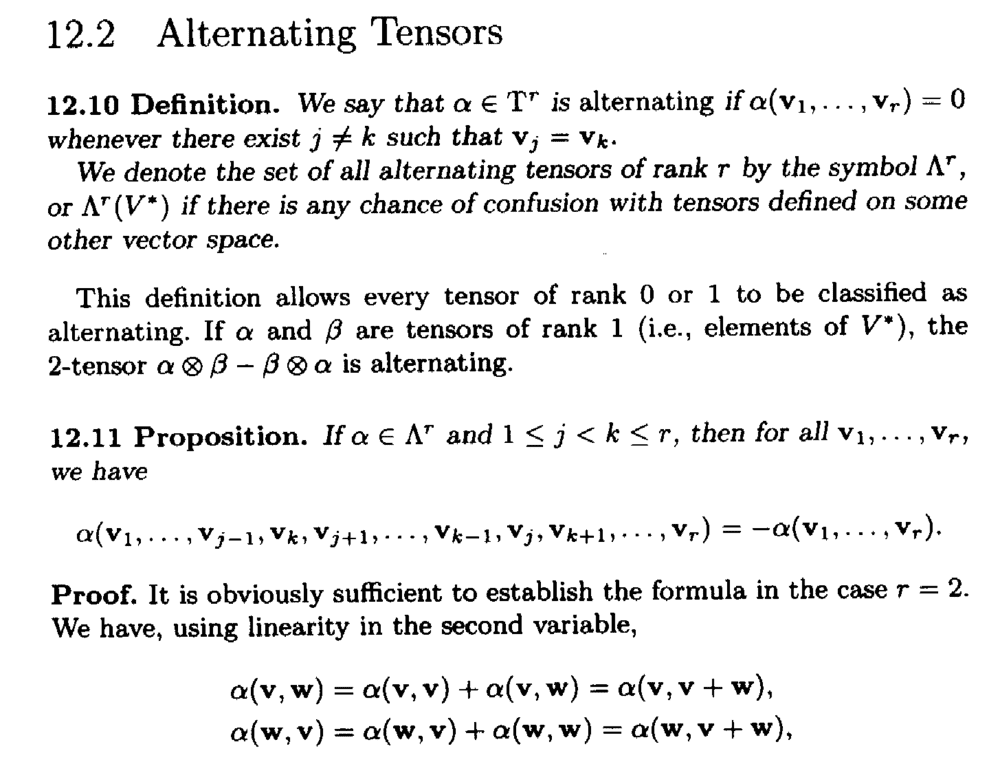
It may also be useful in order to understand the above post for Physics Forum members to have access to Section 12.2 on Alternating Tensors ... so I am providing the same as follows:
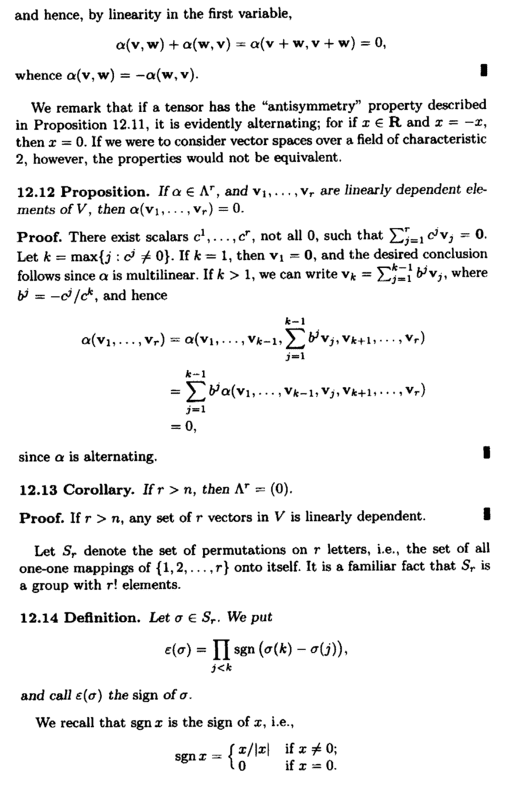
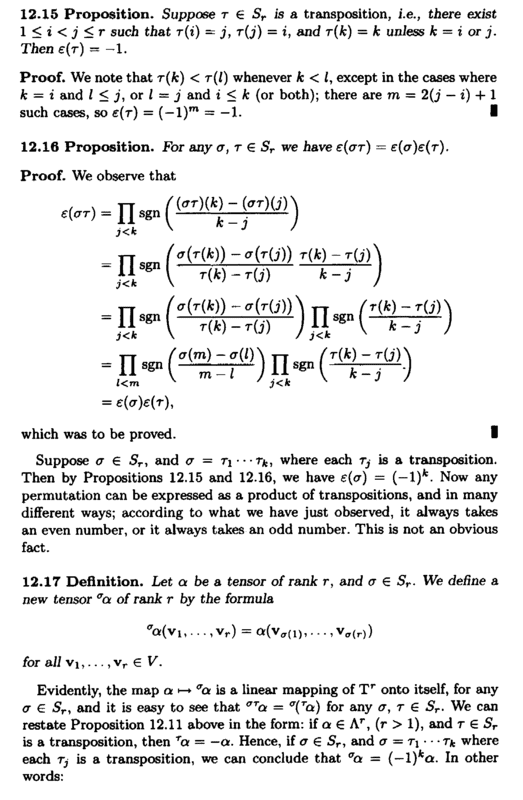
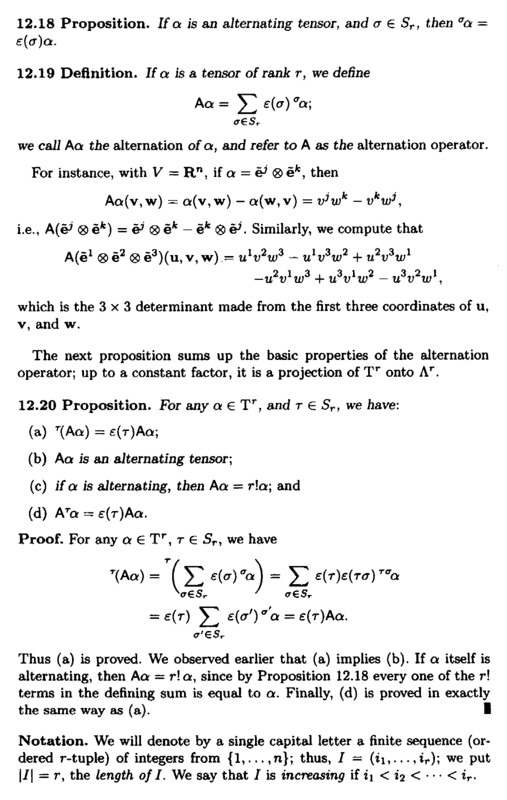 Hope access to the above text helps ...
Hope access to the above text helps ...
Peter
I am currently reading Chapter 12: Multilinear Algebra ... ...
I need some help in order to fully understand the proof of Theorem 12.22 on page 276 ... ...The relevant text reads as follows:
In the above proof by Browder we read the following:
" ... ... the Kronecker delta, and hence, using Proposition 12.20, that for any ##r##-tuples ##I## and ##J##, not necessarily increasing
##\tilde{u}^I ( u_{ j_1 }, \cdot \cdot \cdot u_{ j_r } ) = \varepsilon^I_J##
where ##\varepsilon^I_J##, the "Kronecker epsilon" ... ... "My question is as follows:
How is Proposition 12.20 used to show that ## \tilde{u}^I ( u_{ j_1 }, \cdot \cdot \cdot u_{ j_r } ) = \varepsilon^I_J## ... ... could someone please demonstrate the use of Proposition 12.20 to derive this result ...The above proof refers to Proposition 12.20 so I am providing the text of this proposition as follows:
The above proof also refers (indirectly) to the basis ##( \tilde{u}^1, \cdot \cdot \cdot \tilde{u}^n )## for the dual space ##V^*## ... ... this is mentioned at the start of Section 12.1 ... so I am providing the relevant text as follows:
It may also be useful in order to understand the above post for Physics Forum members to have access to Section 12.2 on Alternating Tensors ... so I am providing the same as follows:
Peter
Attachments
-
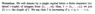 Browder - 1 - Proposition 12.22 ... ... PART 1 ... .png13.8 KB · Views: 296
Browder - 1 - Proposition 12.22 ... ... PART 1 ... .png13.8 KB · Views: 296 -
 Browder - 2 - Proposition 12.22 ... ... PART 2 ... ... .png49.6 KB · Views: 346
Browder - 2 - Proposition 12.22 ... ... PART 2 ... ... .png49.6 KB · Views: 346 -
 Browder ... Proposition 12.20 ... .png44.8 KB · Views: 321
Browder ... Proposition 12.20 ... .png44.8 KB · Views: 321 -
 Browder - 1 - Start of Chapter 12 ... ... PART 1.png75.6 KB · Views: 315
Browder - 1 - Start of Chapter 12 ... ... PART 1.png75.6 KB · Views: 315 -
 Browder - 2 - Start of Chapter 12 ... ... PART 2 .png59.5 KB · Views: 322
Browder - 2 - Start of Chapter 12 ... ... PART 2 .png59.5 KB · Views: 322 -
 Browder - 3 - Start of Chapter 12 ... ... PART 3 .png51.6 KB · Views: 320
Browder - 3 - Start of Chapter 12 ... ... PART 3 .png51.6 KB · Views: 320 -
 Browder - 3 - Start of Chapter 12 ... ... PART 3 .png51.6 KB · Views: 309
Browder - 3 - Start of Chapter 12 ... ... PART 3 .png51.6 KB · Views: 309 -
 Browder - 4 - Start of Chapter 12 ... ... PART 4 .png49.2 KB · Views: 299
Browder - 4 - Start of Chapter 12 ... ... PART 4 .png49.2 KB · Views: 299 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png13.8 KB · Views: 542
?temp_hash=2efdb9106079a85a3568e58731f43740.png13.8 KB · Views: 542 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png49.6 KB · Views: 630
?temp_hash=2efdb9106079a85a3568e58731f43740.png49.6 KB · Views: 630 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png44.8 KB · Views: 656
?temp_hash=2efdb9106079a85a3568e58731f43740.png44.8 KB · Views: 656 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png75.6 KB · Views: 550
?temp_hash=2efdb9106079a85a3568e58731f43740.png75.6 KB · Views: 550 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png59.5 KB · Views: 524
?temp_hash=2efdb9106079a85a3568e58731f43740.png59.5 KB · Views: 524 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png51.6 KB · Views: 526
?temp_hash=2efdb9106079a85a3568e58731f43740.png51.6 KB · Views: 526 -
 ?temp_hash=2efdb9106079a85a3568e58731f43740.png49.2 KB · Views: 556
?temp_hash=2efdb9106079a85a3568e58731f43740.png49.2 KB · Views: 556 -
 Browder - 1 - Start of Section 12.2 ... ... PART 1 .... .png53.8 KB · Views: 357
Browder - 1 - Start of Section 12.2 ... ... PART 1 .... .png53.8 KB · Views: 357 -
 Browder - 2 - Start of Section 12.2 ... ... PART 2 .... .png36.4 KB · Views: 303
Browder - 2 - Start of Section 12.2 ... ... PART 2 .... .png36.4 KB · Views: 303 -
 Browder - 3 - Start of Section 12.2 ... ... PART 3 .... .png53.2 KB · Views: 329
Browder - 3 - Start of Section 12.2 ... ... PART 3 .... .png53.2 KB · Views: 329 -
 Browder - 3 - Start of Section 12.2 ... ... PART 3 .... .png53.2 KB · Views: 292
Browder - 3 - Start of Section 12.2 ... ... PART 3 .... .png53.2 KB · Views: 292 -
 Browder - 4 - Start of Section 12.2 ... ... PART 4 .... .png41.8 KB · Views: 307
Browder - 4 - Start of Section 12.2 ... ... PART 4 .... .png41.8 KB · Views: 307 -
 ?temp_hash=2932f319424fbb980ad6d60a210b478c.png53.8 KB · Views: 573
?temp_hash=2932f319424fbb980ad6d60a210b478c.png53.8 KB · Views: 573 -
 ?temp_hash=2932f319424fbb980ad6d60a210b478c.png36.4 KB · Views: 554
?temp_hash=2932f319424fbb980ad6d60a210b478c.png36.4 KB · Views: 554 -
 ?temp_hash=2932f319424fbb980ad6d60a210b478c.png53.2 KB · Views: 512
?temp_hash=2932f319424fbb980ad6d60a210b478c.png53.2 KB · Views: 512 -
 ?temp_hash=2932f319424fbb980ad6d60a210b478c.png41.8 KB · Views: 534
?temp_hash=2932f319424fbb980ad6d60a210b478c.png41.8 KB · Views: 534
Last edited: