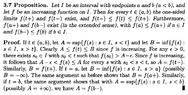- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading Andrew Browder's book: "Mathematical Analysis: An Introduction" ... ...
I am currently reading Chapter 3: Continuous Functions on Intervals and am currently focused on Section 3.1 Limits and Continuity ... ...
I need some help in understanding the proof of Proposition 3.14 ...Proposition 3.14 and its proof read as follows:
View attachment 9536
In the above proof by Browder we read the following:
" ... ... For any \(\displaystyle d \in I, d\) not an endpoint of \(\displaystyle I\) we know (Proposition 3.7) that \(\displaystyle f(d-)\) and \(\displaystyle f(d+)\) exist with \(\displaystyle f(d-) \leq f(d) \leq f(d+)\), so \(\displaystyle d \in D\) if and only if \(\displaystyle f(d-) \lt f(d+)\). ... ... "My question is as follows:
Can someone please demonstrate (rigorously) exactly why/how it follows that \(\displaystyle d \in D\) if and only if \(\displaystyle f(d-) \lt f(d+)\). ... ... Help will be much appreciated ...
Peter
=======================================================================================The above post mentions Browder Proposition 3.7 ... so I am providing the text of that proposition ... as follows:
View attachment 9537
Hope that helps ...
Peter
I am currently reading Chapter 3: Continuous Functions on Intervals and am currently focused on Section 3.1 Limits and Continuity ... ...
I need some help in understanding the proof of Proposition 3.14 ...Proposition 3.14 and its proof read as follows:
View attachment 9536
In the above proof by Browder we read the following:
" ... ... For any \(\displaystyle d \in I, d\) not an endpoint of \(\displaystyle I\) we know (Proposition 3.7) that \(\displaystyle f(d-)\) and \(\displaystyle f(d+)\) exist with \(\displaystyle f(d-) \leq f(d) \leq f(d+)\), so \(\displaystyle d \in D\) if and only if \(\displaystyle f(d-) \lt f(d+)\). ... ... "My question is as follows:
Can someone please demonstrate (rigorously) exactly why/how it follows that \(\displaystyle d \in D\) if and only if \(\displaystyle f(d-) \lt f(d+)\). ... ... Help will be much appreciated ...
Peter
=======================================================================================The above post mentions Browder Proposition 3.7 ... so I am providing the text of that proposition ... as follows:
View attachment 9537
Hope that helps ...
Peter
Attachments
Last edited: