- #1
Pekkeri
- 5
- 0
Hi,
I need to choose a hydraulic hub motor for a vehicle which weighs 25 000 N when fully loaded. The vehicle must be able to start moving (fully loaded) on a inclined surface (30 degrees angle). So I need to determine the torque needed to get vehicle moving. The vehicle has three wheels from which only one (diam. 450 mm) will be driving (ohter 2 are driven). This has to be an approximate calculation so I have only taken into account the weight of the vehicle and the force needed to overcome rolling friction. The maximum speed to be achieved is 4 km/h but as a first step I just need to know how much torque is needed to get the thing moving at all.
Is my solution valid or am I doing it wrong, because i get a pretty big torque value? Any help and opinion is welcome !
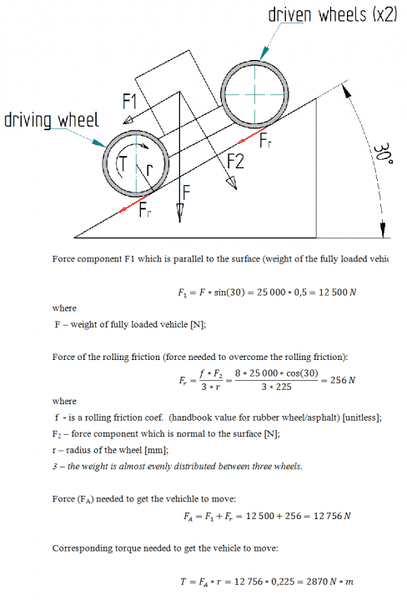
I need to choose a hydraulic hub motor for a vehicle which weighs 25 000 N when fully loaded. The vehicle must be able to start moving (fully loaded) on a inclined surface (30 degrees angle). So I need to determine the torque needed to get vehicle moving. The vehicle has three wheels from which only one (diam. 450 mm) will be driving (ohter 2 are driven). This has to be an approximate calculation so I have only taken into account the weight of the vehicle and the force needed to overcome rolling friction. The maximum speed to be achieved is 4 km/h but as a first step I just need to know how much torque is needed to get the thing moving at all.
Is my solution valid or am I doing it wrong, because i get a pretty big torque value? Any help and opinion is welcome !