Transformer induced voltage

[nemesiswes]

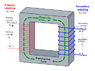
Why is the Voltage induced on the secondary of a transformer in phase with the primary? As far as I know, lens law states that any induced voltage on a coil is 180 degrees out of phase with the coil that generated magnetic field. Otherwise you could have infinite energy systems, lol.

Is the reason the voltage is always seen to be in phase on the secondary with the primary because of the windings, when it reality the Voltage is actually 180 degrees out of phase? I always figured since the Back-emf is opposite the primary voltage, the secondary is as well since it is created from that same magnetic field.

Please help, I have read plenty on transformers and this is just one thing I do not get?

Here is one good source I found but I just don't get why.

http://www.allaboutcircuits.com/vol_2/chpt_9/1.html

 

[vk6kro]

Try looking at it like this:

A coil with an AC voltage across it will have an opposing voltage developed in it that opposes the applied EMF.
This is the "back EMF". Its polarity is the same as the applied voltage.

This voltage comes from the flux in the core of a transformer.

So, if you had another identical coil in almost the same position, it is reasonable that it would have almost the same voltage developed in it, and this voltage would have the same polarity as the applied voltage too.

 

[nemesiswes]

Wait I thought the Back-EMF (voltage) has the opposite polarity of the applied voltage? Otherwise it would just add to the Applied voltage. The back-emf acts to decrease the effect of the applied voltage resulting in a net voltage

Applied = 12V
Back-emf = -11V

net voltage = 1v

Basically like that.

 

[nemesiswes]

I even read on wiki that the Back-emf is opposite in polarity to that of the applied voltage

http://en.wikipedia.org/wiki/Counter-electromotive_force


Did I not read that right or not get it?

 

[vk6kro]

No, the back emf has the same polarity as the applied voltage, but this opposes current from the source flowing into the inductor.

http://dl.dropbox.com/u/4222062/transformer%20back%20EMF.PNG

Note the arrows showing the polarity.

Notice that the net voltage in the circuit depends on the difference between the applied EMF and the back EMF.
Around the loop at the left, the back EMF does oppose the applied EMF, but the two voltages are almost the same when viewed as a parallel circuit.

 

[nemesiswes]

How can the back-EMF have the same polarity as the applied voltage yet still oppose the current?

 

[nemesiswes]

Wait I think I have a idea why I didn't get it. I am slow, lol.

But really I think I got it, you see I took your picture too literally, lol. The back-emf you showed in the picture, I viewed as another source separate from the coil. Obviously you can see the problem then. What you really meant which I did not see was that the back-emf in the picture is what the coil produces to counter the applied voltage.

So the back-emf is and would have to be in phase with the applied voltage. If it were not then like I said in one of the older post's, you could have a infinite energy machine, lol.

It makes sense now and I see why the secondary is in phase with the Primary. Because the Back-Emf is in phase with the applied voltage and thus since the Back-Emf is created by the changing magnetic flux, the Secondary must also be in phase with the back-emf which just so happens to be in phase with the applied voltage.

So is this right.

 

[vk6kro]

Yes, I think you have it now.

The back EMF generated in the coil is only slightly less than the applied voltage so there is only a very small voltage (the difference between the two) driving current around the left hand loop.

 

[nemesiswes]

Yea I got that part too, lol.

Thank you for your help, you really helped me understand why the back-emf is in phase with the applied voltage, I don't know how I ever thought otherwise, lol.

 

[truesearch]

If there is no resistance in the circuit the induced (back emf) in the coil must be equal to the applied emf. It does not make sense to say it is 'slightly less' than the applied voltage. How much is 'slightly less'...how do you calculate the difference.
This misunderstanding comes about because it is difficult to realize that there can be a current without a 'resultant' emf. Faraday's law states e = LdI/dt and if there is no resistance e = E.
It is the electrical analogy of constant velocity with no need for a resultant force.
If there is resistance then e = LdI/dt = E - Ir...'resultant' emf = e

 

[vk6kro]

Since we were talking about transformers, it is reasonable to allow for the losses in the iron core.

Also, nobody said there was no resistance in either of the windings, even though that diagram doesn't show it.

 

[truesearch]

In post 3 nemes.. has applied emf = 12 V, back emf = 11V. He is on the wrong track.
You diagram is perfectly correct with the 2 arrows representing applied emf and back emf...the 2 red arrows. These must be equal ! It is the way induction works and a basic fact in physics. The applied emf does not need to be 'slightly bigger' than the back emf to 'drive current'.
As an example: if an inductance of 10H experiences a current increasing at 1A/sec what emf must be connected to enable this to occur. What is the back emf in such a case.

 

[vk6kro]

Draw yourself a voltage vector diagram showing voltages across an inductor, across the series resistance in the inductor and the voltage across the reactance of the inductor.

You can see that unless the resistance is zero (which it can't be) then the voltage across the combined impedance is greater than that across the reactance alone.
The voltage across the reactance is the back EMF and the applied voltage appears across the impedance of the coil.

http://dl.dropbox.com/u/4222062/Series%20R%20L%20vector.PNG

You can see that VL is smaller than Vz.

Incidentally, you might like to fix the typo in this post:
https://www.physicsforums.com/showpost.php?p=3915830&postcount=5

 

[truesearch]

What you have drawn here is a phasor diagram for an AC circuit. These 3 voltage phasors are either rms or peak voltages. They are not instantaneous voltages. If you have a way of measuring instantaneous voltages (a cro will do the job) you will find that
vs = vr + vl These are instantaneous voltages.
At the instant shown in your diagram Vr = 0, Vl = max and Vs = Vsmax Sin(phi)
Vl = Vs AT THIS INSTANT...applied emf = back emf

 

[jimalex]

Please correct me if i am wrong, but the problem here as i see it is we are mincing our terms with what is a back-voltage and what is an induced voltage.

the back voltage is caused mainly in the primary, for example, reacting to the transformer's power supply from whereever. as the current in the primary tries to rise or fall, the field in the primary will either rob it for energy or deliver back the previously robbed energy respectively.

once the current in the primary does rise, after overcoming the back-voltage, the field builds up in the primary and core and secondary simultaneously, and the voltage induced in the secondary is practically instantaneous, hence the polarity relationship.

Sound right?
J

 

[vk6kro]

Please correct me if i am wrong, but the problem here as i see it is we are mincing our terms with what is a back-voltage and what is an induced voltage.

the back voltage is caused mainly in the primary, for example, reacting to the transformer's power supply from whereever. as the current in the primary tries to rise or fall, the field in the primary will either rob it for energy or deliver back the previously robbed energy respectively.

once the current in the primary does rise, after overcoming the back-voltage, the field builds up in the primary and core and secondary simultaneously, and the voltage induced in the secondary is practically instantaneous, hence the polarity relationship.

Sound right?
J

The current in the primary is a function of the inductance of the primary and this produces a flux in the core of the transformer which is 90 degrees out of phase with the applied voltage. This then causes a back EMF in the primary (and the secondary) which is a further 90 degrees out of phase with the flux. This voltage is the "back EMF".

 

[vk6kro]

What you have drawn here is a phasor diagram for an AC circuit. These 3 voltage phasors are either rms or peak voltages. They are not instantaneous voltages. If you have a way of measuring instantaneous voltages (a cro will do the job) you will find that
vs = vr + vl These are instantaneous voltages.
At the instant shown in your diagram Vr = 0, Vl = max and Vs = Vsmax Sin(phi)
Vl = Vs AT THIS INSTANT...applied emf = back emf

And when VL = 0 then Vz = Vr but so what?

Voltage is measured as RMS volts not instantaneous volts chosen at the instant you choose to accept.

 

[jimalex]

vk6kro,

i knew the back voltage on the primary would be 180 degrees out of phase, but I think now i understand better why you refer to the voltage induced in the secondary as back-voltage, since it is in time (phase) with the backvoltage in the primary.

however, I thought the right-hand rule would indicate the secondary current flowing in the other direction in this picture, so I'm still off on this somewhere. what am i missing?

thanks for y'alls patience.

 

[truesearch]

The 3 voltage phasors in these diagrams show the values of the voltages (max or rms) at different times.
If you want to know anyof the voltages at a particular instant, so you can see how they are related, then you need the projection of each phasor on the vertical axis.
So...in answer to '...but so what?...' when VL =0, Vr = maxVr and Vz(Vs) = VsCos(phi)
So Vr = VsCos(phi) and VL =0. The supply voltage only produces Vr, the supply voltage produces zero VL...confirmation that VL = Induced emf = back emf
There are 358 degrees left to work through. I can guarantee you that you will always find that VL = the component of Vs.
It is not necessary to revert to this phasor diagram to bring out Faradays Law.
Induced emf = e = LdI/dt = applied emf. The phasor diagram shows Faradays law in operation.

A current increases at a rate of 1A/s through an inductance of 10H. What emf is required for this to happen, what is the induced (back) emf... any offers?

PS: what typo did I make? is it spelling, punctuation or physics knowledge? Point it out please but I think I cannot correct it now in the original.

 

[vk6kro]

vk6kro,

i knew the back voltage on the primary would be 180 degrees out of phase, but I think now i understand better why you refer to the voltage induced in the secondary as back-voltage, since it is in time (phase) with the backvoltage in the primary.

however, I thought the right-hand rule would indicate the secondary current flowing in the other direction in this picture, so I'm still off on this somewhere. what am i missing?

thanks for y'alls patience.

Yes, I think the secondary voltage shown in that diagram is wrong too.

If you follow the winding direction around the top of the core, the secondary is a continuation of the primary winding, (with turns missing, of course), so the bottom of the secondary winding should have the same polarity as the top of the primary.

I notice in the Wikipedia article, in the "Talk" section at the top of the article, there is discussion that a similar diagram is wrong. As far as I can see, the diagrams are identical in function so this one is probably wrong too.
This is the one the author admits is wrong:

But he still claims this one is right:

I can't see any difference.

 

[truesearch]

I think the diagrams are correct. The polarity of the applied primary voltage is to cause current to flow in the primary (The primary back emf will have the same poarity).
The polarity of the secondary emf is the polarity of the voltage generated IN THE COIL...this is an emf source and current flows from - to + through the emf source.
Hope this helps

 

[Jony130]

I also think that the diagram are correct. Simply use right-hand rule and you will see that every think is OK. Also kept in mind that the voltage on the secondary winding is the induced voltage (emf). And the polarity of this induced voltage is determined by Lenz's Law. The secondary winding will generate the emf voltage in such a direction to create magnetics field that is oppose the primary winding magnetic field .
See this example

Also see this short video
http://www.youtube.com/watch?v=qWu82nJS42I&feature=relmfu

 

[truesearch]

The only difference between the top diagram and the bottom diagram is that no polarity is shown on the terminals in the top diagram. If they are both marked + then that will be correct and both diagrams will match.

 

[jimalex]

Thanks for the replies, hope nobody minds I kinda hijacked Nemesiswes' thread

The diagrams in Jony130's post I think I understand- the polarity of the magnet being reversed changes the polarity of the induced voltage.

Where you may have to help me again is to understand the polarity of the transformer core as a ring. I thought it wasn't that it had any magnetism of its own (except for the small amount in the magnetic hysteresis loop) but was just a conductor for the flux coming out of the primary. I think that when currents make a magnetic field the right-hand rule applies to the direction to the flux. is the reverse true for a flux inducing a current?

this is great - thanks for the answers.

 

[jimalex]

Also, I see no difference in the wikipedia diagrams, except that the leakage flux in the secondary is opposed to the flux coming directly through the core into the secondary. probably just showing my ignorance here, again...

 

[truesearch]

Remember that if any current is flowing in the secondary coil it is opposing the change in flux caused by the primary current.
If no current is being drawn from the secondary there will be no flux due to the secondary.