Tricky problem - Find E-field of a sheet of charge

[mindarson]

Homework Statement

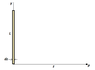
Consider a uniformly charged, infinitesimally thin rectangular sheet with total charge Q extending from x = 0 to x = -w and from y = 0 to y = L. Find the electric field at an arbitrary distance x along the positive x-axis.

Homework Equations

Superposition; E = ∫kdq/r²

The Attempt at a Solution

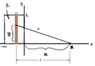
The picture shows how I'm approaching this problem. In the integral, 'dR' just means I'm doing a double integral over the rectangular region labeled R. I've pulled the charge density σ out of the integral, since the problem states it is uniform, therefore constant.

The confusion I'm having is that I need a pair of variables to integrate with respect to over the rectangular region. THEN I need to express the distance r in terms of those variables, so that I can actually do the integral. Since the region I'm integrating over is rectangular, I'd like to use typical x and y as variables, and I already have the limits of integration for those variables. BUT I haven't been able to express the distance, r, from source point to my point on the positive x-axis in terms of the variables x and y. I tried to use trig but that just seems to confuse things.

Finally, I don't know if this is important or not, but I haven't been able to find a way to account for that bit of horizontal distance on the left side of the y-axis, i.e. what would be the x-coordinate of the vector from the origin to my (arbitrary) source point. If I could find an expression for that, then maybe I could just use Pythagoras's Theorem to get an expression for r in terms of x and y?

Can anyone offer any pointers?

 

[TSny]

You could try breaking the rectangular area into vertical strips of infinitesimal width. So, you might first want to solve the problem of the electric field at a point p on the x-axis a distance z from one of the strips.

 

[Simon Bridge]

Define a surface element $dS$ at position $(y,z)$ charge $\sigma dS$
Then you are integrating over y and z as well as accounting for the out-of-plane displacements.

As TSny suggests, it can be easier to work out the strips first.

 

[mindarson]

So if I understand what you're getting at, I will need to take a 'representative strip' and treat it as a line charge, computing the electric field due to that line charge. Which will be an integral over the y-interval [0, L]. Then I need to take that integral and integrate it wrt z (i.e. from 0 to -w).

Hopefully that's correct?

I'll give it a try and post my further attempts. Thanks, guys!

 

[TSny]

So if I understand what you're getting at, I will need to take a 'representative strip' and treat it as a line charge, computing the electric field due to that line charge. Which will be an integral over the y-interval [0, L]. Then I need to take that integral and integrate it wrt z (i.e. from 0 to -w).

That sounds good except for the range of integration of z.

 

[Simon Bridge]

Oh - and remember that the electric field is a vector.
The examples usually put the x-axis through the center so symmetry arguments can come into play more easily.

 

[mindarson]

Alright, here's what I have so far.

For the E-field at p due to a 'representative strip' as you guys advised, I have

E_line = kλ∫(y²+z²)^-1/2dy (this is a definite integral, from O to L, and λ is the constant linear charge density).

Then I need to integrate that over the horizontal (z?) axis from -w to 0:

E_total = ∫[∫(y²+z²)^-1/2dy]dz

Where the first (outer) integral has limits -w to 0 and the inner has limits 0 to L.

Am I on the right track here? Thanks for your help!

UPDATE: When I try the above, the limits I put on z (which TSny questioned) require me to take the natural log of 0. So it seems clear to me that my limits of integration on that variable are messed up. But I don't know what else to use? It seems to me I need to integrate over the interval [-w,0] on the horizontal axis.

 

[mindarson]

The integral I end up with is (integrated from -w to 0):

∫(1/z)tan^-1(L/z)dz

But the integrand is not defined/continuous at the rightmost limit of integration. So I'm thinking maybe I need to use a method for improper integrals, from basic calculus, such as

lim_t→0^-∫(1/z)tan^-1(L/z)dz

where the integration limits are -w to t.

Or am I over-thinking this?

 

[TSny]

Alright, here's what I have so far.

For the E-field at p due to a 'representative strip' as you guys advised, I have

E_line = kλ∫(y²+z²)^-1/2dy (this is a definite integral, from O to L, and λ is the constant linear charge density).

Does the electric field of a point charge vary inversely with the distance or inversely with the square of the distance?

As Simon pointed out, the electric field is a vector quantity. So, you will need to work out the x and y components separately.

Then I need to integrate that over the horizontal (z?) axis from -w to 0:

These are not the correct limits for z. Note that z represents the horizontal distance from the vertical strip to the point of observation.
What is the minimum value of z? the maximum value?

 

[mindarson]

Does the electric field of a point charge vary inversely with the distance or inversely with the square of the distance?

Yeah, I should have caught that!

These are not the correct limits for z. Note that z represents the horizontal distance from the vertical strip to the point of observation.

I'm not understanding why I would integrate over z, if the variable is defined as the horizontal distance from the vertical strip to the point of observation. Don't I ultimately want to integrate over the charge distribution, to get the contribution to the field from each infinitesimal bit of charge?

UPDATE: I think I get the integration over z. The integration over the entire charge distribution is already taken care of by the integration of the strips, right?

 

[TSny]

Yes, the integration over z is the integration over the strips.

 

[mindarson]

Sorry to weary you guys with this problem, but I can't get it to work out.

For the electric field due to a representative line charge, I have

E_line = kλ∫dy/(y²+z²) = (1/z) tan^-1(L/z)

(λ is the charge density)

To get the total Electric field at a point x on the positive horizontal axis, where z is defined as the horizontal distance from the strip of width dz to the point x, I integrate

E_total = (kλ)∫(1/z)tan^-1(L/z)dz

Now, what interval do I integrate this over? The only one that makes sense to me is from -w to x (where x is the point where the field is being evaluated). However, when I carry out the calculution (using integration by parts and u-substitution), my answer includes a term tan^-1(L/z). But this is not defined at z = 0, so I have no idea what to do with that. I don't know how to avoid the division by zero problem.

 

[Simon Bridge]

I don't see how you have accounted for the electric field being a vector...

Note:
The sheet is in the y-z plane.
A single element at point $\small (0,y,z)$ has area $\small dydz$ and charge $$dq=\frac{Q}{wL}dydz$$.
The electric field at point $\small (x,0,0)$ due to that element is ...

$$d\vec{E}=\frac{kdq}{r^3}\vec{r}$$ where $\small \vec{r}$ points from $\small (0,y,z)$ to $\small (x,0,0)$.

You could brute-force the calculation from there. OR you can finesse it by starting out with the equation for the field at $\small (x,0,0)$due to a strip of charge, width dz, through the origin ... write it out in vector notation.

Then consider how the geometry changes as the line moves to a location $\small z\neq 0$. The magnitude and the direction will change right? How?

The fields due to all the strips will add up - this will be a vector sum so be careful.
Use your powers of reason and deduction to figure out how this affects the integral.

Now possibly I just didn't follow how you set up the integral.

 

[TSny]

Here is how I was interpreting the quantity "z". It's just the distance from a strip to the point of observation. Note z is never 0. What are the minimum and maximum values of z?

You need to set up separate integrals for the x and y components of E.

 

[mindarson]

I'm actually fairly lost now, but not hopelessly so. Simon Bridge, you're right that I didn't treat the field as a vector. I just mindlessly started using equations without considering what kind of object I'm dealing with.

I'm pretty confused about the coordinates/variables now. I thought at first that this was a purely 2-dimensional problem, all in the plane. TSny defined the variable z (tracked along the horizontal axis, defined as the horizontal distance between charge elements and point of interest, labeled x in the original problem). And now you are talking about points with 3 coordinates, but I don't even know how another axis (aside from those pictured) even comes into play here.

So I'm finding it hard, right off the bat, to talk about vectors here, since I'm not even sure which variables stand for what or where the coordinate axes are directed.

 

[mindarson]

TSny, thanks for clarifying what you meant by the z variable. I let myself get confused, because it's hard for me to think outside the box of the usual, conventionally defined {x,y,z} variables.

And yeah, I really never even thought about the vector nature of the field and how that needs to be reflected in my calculation.

I will give it another go. Thanks to both of you for your patience.

 

[TSny]

I probably should have used a symbol different from z. Feel free to change it to whatever you want.

 

[Simon Bridge]

Since z is used in the problem statement for a coordinate axis, it is not surprising TSny's use confused you.
The trick to doing these things is to go one step at a time and be clear at each stage about what you are dealing with. It helps if you are thinking geometrically rather than in terms of finding equations to put numbers into.

Try using the notation in post #13.
Explore the brute-force approach first, since you are having trouble with the geometry.
Do you understand where the expression for dq came from?

 

[TSny]

Since z is used in the problem statement for a coordinate axis, it is not surprising TSny's use confused you.

I don't see a reference to z in the problem statement. But, I agree that I made a poor choice in using z.

 

[mindarson]

The components thing is really throwing me for a loop. The only way I know how to approach it is in a roundabout, clumsy way using trig (?). Kind of like so:

E = Ecosθ e1 + Esinθ e2

where e1 is i hat and e2 is j hat (sorry I don't know very well how to format the math-speak.)

θ is the angle between the vector r and the horizontal axis.

cosθ = z/r, sinθ = y/r

Then E = Ez/r e1 + Ey/r e2

Since E = kdq/(r³) = kdq/((y²+z²)^3/2)

I can write the component form as

kdq/((y²+z²)^3/2)[z e1 - y e2]

IF this is useful (highly dubious), it's not clear to me how. I don't even know what I would use as my length element to multiply by the charge density to sub in for dq. Do I still want to use my original strategy of integrating this initial integral over y on [0,L], then integrating that result over z on an interval such as [x, x+w]?

 

[mindarson]

Try using the notation in post #13.
Explore the brute-force approach first, since you are having trouble with the geometry.
Do you understand where the expression for dq came from?

Umm, only in a vague, not-very-useful kind of way. If I rearrange it algebraically I get

wLdq = Qdydz

I interpret this as

(area of charge distribution)*(charge element) = (total charge)*(area element)

which makes perfect sense to me. Two integrals that are equal, just done with respect to different variables, right? But I would never have arrived at that on my own, nor do I immediately see the usefulness.

 

[Simon Bridge]

Rechecks ... hey you're right! The sheet is in the x-y plane.
In that case we have a lot of fun ... the z in #13 should be an x ... what fun!
That will be why you changed the variable name huh?
But at least that makes it easier.

@mindarson - post #13 assumes that the sheet of charge in in the y-z plane.
But your diagram does have it in the x-y plane.

Lets adjust the notation a bit.
See next post.

 

[TSny]

kdq/((y²+z²)^3/2)[z e1 - y e2]

IF this is useful (highly dubious), it's not clear to me how. I don't even know what I would use as my length element to multiply by the charge density to sub in for dq. Do I still want to use my original strategy of integrating this initial integral over y on [0,L], then integrating that result over z on an interval such as [x, x+w]?

This looks very good. Write dq in terms of the area of a small piece of a strip.

 

[mindarson]

Okay, I have pondered the expression for dq in post #13 in light of post #23, and I think I have some understanding of it.

The expression is

dq = (Q/wL)dxdy

so (Q/wL) is just the charge density (per unit area), right?

 

[Simon Bridge]

The reasoning follows the same approach - put the observer at $\small (x_0,0,0)$ to distinguish from the coordinates you are to integrate over.

Then the element at $\small (x,y,0)$ : $\small 0<x<-w, 0<y<L$ has area $\small dxdy$ and charge $$dq=\frac{Q}{wL}dxdy$$
You don't understand why that is?
You are given a surface of area A=wL (width times height) that holds a total charge of Q.
Therefore - what is the charge density?
[edit: you got it! Well done.]

You need this because you need to know the field due to a small element of charge dq that occupies the infinitesimal area dxdy. This replaces the point charge that you first learned to use.

The electric field is given by: $$d\vec{E}=\frac{kdq}{r^3}\vec{r}$$ where $\small \vec{r}$ points from $\small (x,y,0)$ to $\small (x_0,0,0)$

You'll end up with an equation in terms of $\small x_0$ instead of x. Don't worry about that just yet.
[edit]OTOH: if you are following TSny again and it now makes sense - just keep going. ;)

 

[TSny]

Okay, I have pondered the expression for dq in post #13 in light of post #23, and I think I have some understanding of it.

The expression is

dq = (Q/wL)dxdy

so (Q/wL) is just the charge density (per unit area), right?

Right. But since I forced you into writing the width of a strip as dz, you will want to write it as dq = (Q/wL)dydz.

As Simon says, "this is fun".

 

[mindarson]

Using dq = (Q/wL)dzdy, this gives me for my integral (I'm using the z variable as defined by TSny)

E = kQ/(wL)∫1/(y²+z²)^3/2(z e1 + y e2)dzdy

(Sorry it's so ugly!)

The integral is clearly a double integral, and I'm thinking the integration limits for dy are 0 and L, while the integration limits for dz are x and x + w, since x ≤ z ≤ x + w.

(x being the horizontal distance from origin to point of interest.)

 

[TSny]

E = kQ/(wL)∫1/(y²+z²)^3/2(z e1 + y e2)dzdy

The integral is clearly a double integral, and I'm thinking the integration limits for dy are 0 and L, while the integration limits for dz are x and x + w, since x ≤ z ≤ x + w.

(x being the horizontal distance from origin to point of interest.)

Looks good to me.

 

[mindarson]

Wow, huge thanks to both of you for getting me this far on this problem! 'Kindness of strangers', eh? I've learned some good physics from this discussion.

 

[TSny]

Good. But I just noticed that you now have the e₂ component as positive. Is that right?

 

[mindarson]

Good. But I just noticed that you now have the e₂ component as positive. Is that right?

No, you're right, it points downwards so should be < 0.