- #1
babaliaris
- 116
- 15
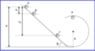
I'm in the chapter of Uniform Circular Motion and I have a hard time understating centipetal acceleration. Until now I knew that acceleration describes "how fast velocity changes in magnitude" except projectile motion because when it reaches maximum height then g is going to change the direction on the Vy and make it move downwards but now in this kind of motion, acceleration describes only "how fast the direction of the velocity changes" and not the magnitude. Ok I understand that but what I really don't understand is when I'm in a car and take a turn I feel pushed in the opposite direction of the circles center. But acceleration is pointing at the center of the circle

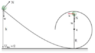
 The projectile moves horizontally with a constant velocity but vertically accelerates with a = -g, so it will hit the ground sometime. The spinning particle accelerates all the time and moves in an orbit where it's velocity changes due to the acceleration. Maybe this is the reason? But there is no way to see it with mathematics? I can't just believe words... There's must be a force on the particle except it's weight (Fg) so ∑ƒy = 0 ⇔ -m⋅g + F = 0 ⇔ F = m⋅g and maybe this F has to due something with the Centripetal force. Probably I'm wrong but this the most logical thing to think of.
The projectile moves horizontally with a constant velocity but vertically accelerates with a = -g, so it will hit the ground sometime. The spinning particle accelerates all the time and moves in an orbit where it's velocity changes due to the acceleration. Maybe this is the reason? But there is no way to see it with mathematics? I can't just believe words... There's must be a force on the particle except it's weight (Fg) so ∑ƒy = 0 ⇔ -m⋅g + F = 0 ⇔ F = m⋅g and maybe this F has to due something with the Centripetal force. Probably I'm wrong but this the most logical thing to think of.