- #1
fonseh
- 529
- 2
Homework Statement
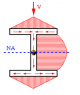
In the notes , I don't understand why the shear stress is maximum at the edge ( circle part) .
Homework Equations
The Attempt at a Solution
I think it's wrong . Refer to another diagram attached , i found that the shear stress varies parabolically across the vertical length of the beam ... Or they are different case ? Can you explain the case in photo 1 ? [/B]