Wheel on a vertical plane. Find tangential speen and acceleration.

[genioaddis]

Homework Statement

Hey all! I'm new here but I think I'll like it. :) I have a question that I can't seem to figure out. Here is the question in short:
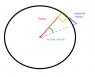
GIVEN: A wheel of radius 1m on a vertical plane rotates with an angular acceleration of 4rad/sec². The wheel starts from rest (ω_o = 0). Point P, on the wheel, is at 57.3° from the horizontal.
REQUIRED: At t=2sec, (a) what is the angular acceleration of the wheel? (b) What is the tangential speed and the total acceleration at P? and (c) What is the angular position of P?

Homework Equations

Some equations needed might be:
ω_f=αt + ω_o
v=ωr
θ=s/r

The Attempt at a Solution

I have attempted all of them. For (a) my answer is to use the first formula I wrote to find 8rad/sec. For (b) I am confused because I don't know if the rotation is Clockwise or Counter Clockwise. Since the rotation is vertical, gravity must have some role in it. Does gravity help the rotation at P or is it against it? (c) I think this one is easy. Solve for s in the third formula. 57.3 radians is my answer.

Please help me out. Thanks so much!

 

[mafagafo]

angular acceleration of 4rad/sec.

To start off, this is not an acceleration. (The units are wrong.)

at 57.3° from the horizontal.

Can you approximate this value in radians?

ω_f=αt + _o

What is this?

v=ωr

Right.

θ=s/r

Right. Here, if you use degrees, you will get that the circumference is equal to the product of the radius by 360°. SEE? Use radians. Reread this last sentence.

Does gravity help the rotation at P or is it against it?

Think about it. If it is clockwise, what does that imply? If it is not, what can you conclude from that? What is the gravity force doing in one side of the wheel? And in the other?

57.3 radians is my answer.

Simply redo your work and PICK EITHER degrees or radians.

 

[genioaddis]

Thanks @mafagafo. I see what you mean. But one last question, which way is the wheel turning?

 

[mafagafo]

I don't like to give answers. You are supposed to learn from here (and this makes this forum so awesome).

If you still have questions, ask them. Don't be ashamed.

If it had an angular velocity of 4 rad/s, the wheel was turning... (go on your own, TIP: wikipedia has it).

It is a standard, as you may have supposed. The negative velocity (-4 rad/s) indicates that the wheel is moving in the other direction.

 

[BiGyElLoWhAt]

First off, Wecome to PF. I think you will like it here =]

gravity acts through the center of mass. Therefore it doesn't exert a torque on an object that is rotating about its center of mass (r=0 thus rxF=0)
Is it's acceleration 4rad/sec^2? If that's the case, given the information above, I don't see a reason why that would change, do you? Are there any torques acting on the wheel? your first equation is "good", but I think you meant $\omega_{f} = \alpha t + \omega_{0}$
I'm not sure waht you're 3rd equation is, is that some kind of trig representation? Or what is 's'?

Anyway, for (b), for the tangential speed, you can use trig to figure that out. See the diagram, I think you can figure it out.
(c) Do you know calculus? $\theta (t) = \int \omega (t)dt$ If not, use the kinematic they gave you in class (the calculus is done for you)

 

[BiGyElLoWhAt]

Oh wow... Forgot to post the diagram XD

 

[genioaddis]

Thanks! I see gravity has no effect. I thought of it as a ball rotating on string.

Or what is 's'?

's' the linear distance.