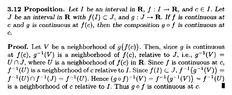Composition of Two Continuous Functions .... Browder, Proposition 3.12 .... ....

In summary, the composition of two continuous functions is a new function created by applying one function to the output of another function. Browder's Proposition 3.12 states that the composition of two continuous functions is also continuous. The conditions for this to be true are that both functions must be continuous, the domain of the inner function must be contained in the domain of the outer function, and the range of the inner function must be contained in the domain of the outer function. The composition is calculated by plugging the inner function into the outer function. This has significance because it allows us to create new functions and make predictions about the behavior of the original functions.
  • #1
Math Amateur
Gold Member
MHB
3,990
48
I am reading Andrew Browder's book: "Mathematical Analysis: An Introduction" ... ...

I am currently reading Chapter 3: Continuous Functions on Intervals and am currently focused on Section 3.1 Limits and Continuity ... ...

I need some help in understanding the proof of Proposition 3.12 ...Proposition 3.12 and its proof read as follows:

View attachment 9519
In the above proof by Browder we read the following:

" ... ... Since \(\displaystyle f(I) \subset J\), \(\displaystyle f^{ -1 } ( g^{ -1 }(V) ) = f^{ -1 } (U) \cap f^{ -1 } (J) = f^{ -1 } (U)\) ... ... "My question is as follows:

Can someone please explain exactly why/how \(\displaystyle f^{ -1 } (U) \cap f^{ -1 } (J) = f^{ -1 } (U)\) ... ...
Help will be much appreciated ...

Peter
 

Attachments

  • Browder - Proposition 3.12 ... .png
    Browder - Proposition 3.12 ... .png
    16.4 KB · Views: 80
Physics news on Phys.org
  • #2
.The reason why f^{ -1 } (U) \cap f^{ -1 } (J) = f^{ -1 } (U) is because the inverse of a function is a one-to-one mapping, meaning that the same value can only map to one other value. Since J is a subset of I, any element in U will necessarily be in J, and thus the intersection of f^{ -1 } (U) and f^{ -1 } (J) will be f^{ -1 }(U).
 
  • #3


Hi Peter,

I can try to help you understand this proof. First, let's define some notation. In this proof, f is a function mapping from interval I to interval J, and g is a function mapping from interval J to some other set V. U is a subset of J, and V is a subset of some other set W. The notation f^{-1}(U) means the set of all points in I that map to U under the function f.

Now, in the proof, we are trying to show that f^{-1}(g^{-1}(V)) = f^{-1}(U). In other words, we want to show that the set of points in I that map to V under the composition of functions g and f is the same as the set of points in I that map to U under f alone.

To do this, we first note that since f(I) is a subset of J, we can say that f^{-1}(J) is a subset of I. This means that any point in I that maps to J under f must also be in I itself. Now, since we know that f^{-1}(U) is a subset of I (since U is a subset of J), we can say that f^{-1}(U) intersect f^{-1}(J) must also be a subset of I. In other words, any point in I that maps to both U and J under f must also be in I itself.

This is where the key step comes in. Since f^{-1}(U) intersect f^{-1}(J) is a subset of I, we can say that it is also a subset of f^{-1}(U). In other words, any point in I that maps to both U and J under f must also map to U alone under f. This is why f^{-1}(U) intersect f^{-1}(J) = f^{-1}(U).

I hope this helps clarify the proof for you. Let me know if you have any other questions. Happy reading!

 

1. What is the significance of Proposition 3.12 in the composition of two continuous functions?

Proposition 3.12 in Browder's work discusses the continuity of the composition of two continuous functions. It states that if two functions, f and g, are both continuous at a point c, then their composition, f(g(x)), is also continuous at c. This is a fundamental result in the study of continuous functions and is frequently used in mathematical analysis and other areas of mathematics.

2. Can Proposition 3.12 be extended to the composition of more than two continuous functions?

Yes, Proposition 3.12 can be extended to the composition of any finite number of continuous functions. This means that if f1, f2, ..., fn are all continuous at a point c, then their composition, f1(f2(...(fn(x)))), is also continuous at c. This is known as the continuity of finite compositions and is a powerful tool in mathematical analysis.

3. What is the proof for Proposition 3.12 in Browder's work?

The proof for Proposition 3.12 is based on the epsilon-delta definition of continuity. It involves showing that for any given epsilon greater than 0, there exists a delta greater than 0 such that if the distance between x and c is less than delta, then the distance between f(g(x)) and f(g(c)) is less than epsilon. This proof is a standard one and can be found in many textbooks on analysis.

4. Are there any conditions in which Proposition 3.12 does not hold?

Yes, there are certain conditions in which Proposition 3.12 does not hold. For example, if one of the functions f or g is not continuous at c, then their composition, f(g(x)), may not be continuous at c. Additionally, if g is not defined at c, then the composition may not be continuous at c. It is important to check for these conditions before applying Proposition 3.12.

5. How is Proposition 3.12 used in real-world applications?

Proposition 3.12 is used in various real-world applications, such as in physics, engineering, and economics. It is particularly useful in the analysis of functions that model natural phenomena, such as the movement of objects or the flow of fluids. In economics, it can be used to analyze the relationships between different variables in a system. Overall, Proposition 3.12 is a powerful tool in understanding the behavior of continuous functions and their compositions.

Similar threads

I Composition of Two Continuous Functions .... Browder, Proposition 3.12
    • Topology and Analysis
Replies
5
Views
2K
MHB Understanding Browder Proposition 3.14: Increasing Function & Discontinuities
    • Topology and Analysis
Replies
2
Views
2K
MHB Increasing Function on an Interval .... Browder, Proposition 3.7 .... ....
    • Topology and Analysis
Replies
3
Views
1K
I Continuity of f^+ .... Browder Corollary 3.13
    • Topology and Analysis
Replies
18
Views
2K
MHB Help Understanding Andrew Browder's Proposition 8.14
    • Topology and Analysis
Replies
2
Views
1K
I Intermediate Value Theorem .... Browder, Theorem 3.16 .... ....
    • Topology and Analysis
Replies
4
Views
2K
MHB Continuity of f^+ .... Browder Corollary 3.13
    • Topology and Analysis
Replies
4
Views
1K
MHB Operator Norm and Cauchy Sequence .... Browder, Proposition 8.7 ....
    • Topology and Analysis
Replies
2
Views
1K
I Riemann Integration ... Existence Result .... Browder, Theorem 5.12 ....
    • Topology and Analysis
Replies
2
Views
2K
MHB Differentials and Jacobians .... Remarks After Browder Prposition 8.21 ....
    • Topology and Analysis
Replies
2
Views
1K

Hot Threads

Recent Insights

Back
Top