- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
- TL;DR Summary
- I am reading Wilson A. Sutherland's book: "Introduction to Metric & Topological Spaces" (Second Edition) ...
I am currently focused on Chapter 8: Continuity in Topological Spaces; bases ...
I need some help in order to prove Definition 8.1 is essentially equivalent to Definition 8.2 ... ... see text below ...
I am reading Wilson A. Sutherland's book: "Introduction to Metric & Topological Spaces" (Second Edition) ...
I am currently focused on Chapter 8: Continuity in Topological Spaces; bases ...
I need some help in order to prove Definition 8.1 is essentially equivalent to Definition 8.2 ... ...
Definitions 8.1 and 8.2 read as follows: ... ...

In the above text we read the following:
" ... ... Then one can prove that ##f## is continuous iff it is continuous at every point of ##X##. ... ... "I sketched out a proof of the above statement ... but am unsure of the correctness/validity of my proof ...
My sketch of the proof is as follows ...First assume f is continuous (Definition 8.1 holds true) ... ...
We are given (Definition 8.2) that ##U' \in T_Y## where ##f(x) \in U'## ... ...
Take ##U = f^{-1} (U')##
Then ##x \in U## ...
Also from Definition 8.1 we have ##U \in T_X## ...
and further ##f(U) = f(f^{-1}(U')) \subseteq U'## ...
... that is Definition 8.2 holds at any ##x \in X## ...Now assume that Definition 8.2 holds true at every x \in X ... that is f is continuous at every point x \in X ...
Let ##V \in T_Y## ... need to show ##f^{-1} (V) \in T_X## ... ...
Now ##x \in f^{-1} (V) \Longrightarrow f(x) \in V##
##\Longrightarrow## there exists a set ##U_x \in T_X## such that ##f(U_x) \subseteq V## by Definition 8.2 ...
But ##f(U_x) \subseteq V \Longrightarrow U_x \subseteq f^{-1} (V)##
Therefore for all ##x \in f^{-1} (V)## we have ##x \in U_x \subseteq f^{-1} (V)##
Therefore ##f^{-1} (V)## is open by Proposition 7.2 ...
Therefore ##f^{-1} (V) \in T_X## ... ...Can someone please confirm that the above proof is correct ... and/or point out the shortcomings/errors ... Hope someone can help ... ...
Peter====================================================================================The above post mentions Proposition 7.2 so I am providing text of the same together with the start of Chapter 7 in order to provide necessary context, definitions and notation ... as follows ... ...

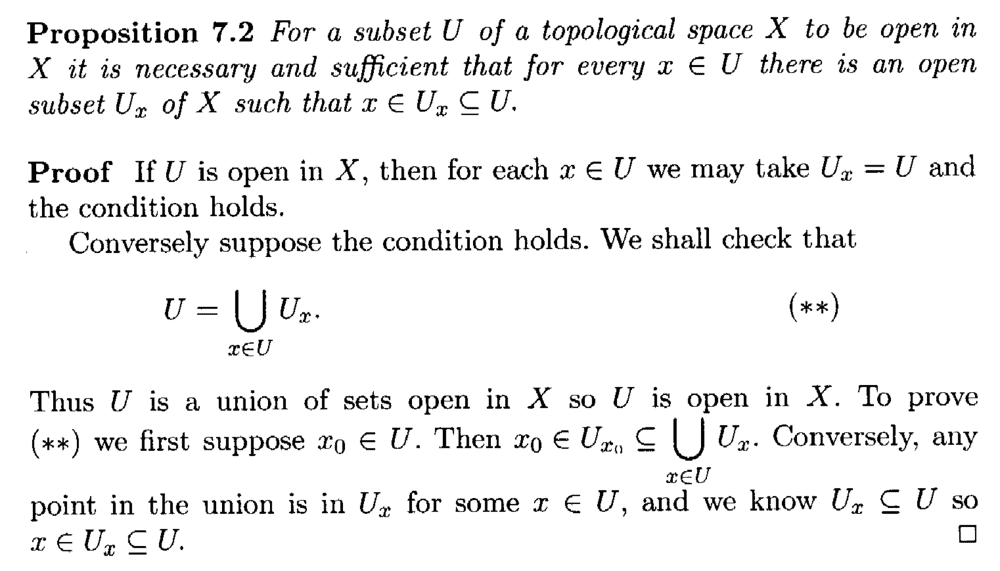
Hope that helps ...
Peter
I am currently focused on Chapter 8: Continuity in Topological Spaces; bases ...
I need some help in order to prove Definition 8.1 is essentially equivalent to Definition 8.2 ... ...
Definitions 8.1 and 8.2 read as follows: ... ...
In the above text we read the following:
" ... ... Then one can prove that ##f## is continuous iff it is continuous at every point of ##X##. ... ... "I sketched out a proof of the above statement ... but am unsure of the correctness/validity of my proof ...
My sketch of the proof is as follows ...First assume f is continuous (Definition 8.1 holds true) ... ...
We are given (Definition 8.2) that ##U' \in T_Y## where ##f(x) \in U'## ... ...
Take ##U = f^{-1} (U')##
Then ##x \in U## ...
Also from Definition 8.1 we have ##U \in T_X## ...
and further ##f(U) = f(f^{-1}(U')) \subseteq U'## ...
... that is Definition 8.2 holds at any ##x \in X## ...Now assume that Definition 8.2 holds true at every x \in X ... that is f is continuous at every point x \in X ...
Let ##V \in T_Y## ... need to show ##f^{-1} (V) \in T_X## ... ...
Now ##x \in f^{-1} (V) \Longrightarrow f(x) \in V##
##\Longrightarrow## there exists a set ##U_x \in T_X## such that ##f(U_x) \subseteq V## by Definition 8.2 ...
But ##f(U_x) \subseteq V \Longrightarrow U_x \subseteq f^{-1} (V)##
Therefore for all ##x \in f^{-1} (V)## we have ##x \in U_x \subseteq f^{-1} (V)##
Therefore ##f^{-1} (V)## is open by Proposition 7.2 ...
Therefore ##f^{-1} (V) \in T_X## ... ...Can someone please confirm that the above proof is correct ... and/or point out the shortcomings/errors ... Hope someone can help ... ...
Peter====================================================================================The above post mentions Proposition 7.2 so I am providing text of the same together with the start of Chapter 7 in order to provide necessary context, definitions and notation ... as follows ... ...
Hope that helps ...
Peter