Fields and Field Extensions - Dummit and Foote, Ch. 13 .... .

[Math Amateur]

I am reading Dummit and Foote, Chapter 13 - Field Theory.

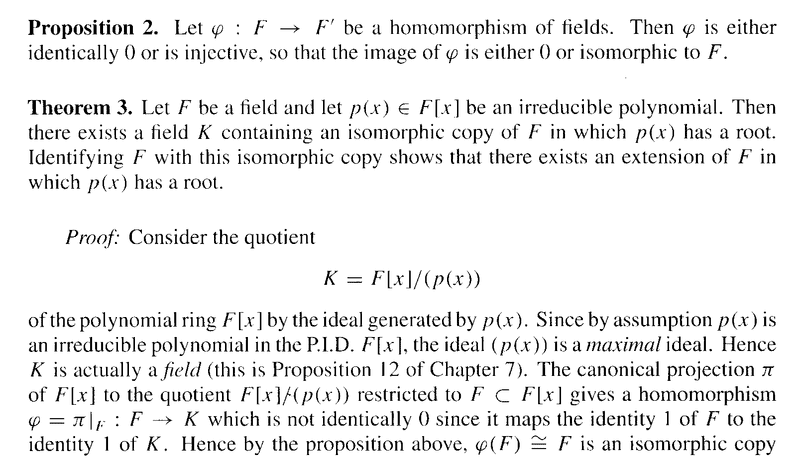
I am currently studying Theorem 3 [pages 512 - 513]

I need some help with an aspect the proof of Theorem 3 ... ...

Theorem 3 on pages 512-513 reads as follows:

In the above text from Dummit and Foote, we read the following:

" ... ... We identify $F$ with its isomorphic image in $K$ and view $F$ as a subfield of $K$. If $\overline{x} = \pi (x)$ denotes the image of $x$ in the quotient $K$, then

$p( \overline{x} ) = \overline{ p(x) }$ ... ... (since $\pi$ is a homomorphism)

... ... "My question is as follows: ... where in the proof of $p( \overline{x} ) = \overline{ p(x) }$ does it depend on $\pi$ being a homomorphism ... ...

... indeed, how does one formally and rigorously demonstrate that $p( \overline{x} ) = \overline{ p(x) }$ ... ... and how does this proof depend on $\pi$ being a homomorphism ...
To make my question clearer consider the case of $p(x) = x^2 - 5$ ... ...

Then ...

$p( \overline{x} ) = \overline{x}^2 - 5_K$

$= ( x + ( p(x) ) ( x + ( p(x) ) - ( 5 + ( p(x) )$

$= ( x^2 + ( p(x) ) - ( 5 + ( p(x) )$

$= (x^2 - 5) + ( p(x) ) = 0$

$= \overline{ p(x) }$... ... in the above case, my question is ... where does the above calculation depend on $\pi$ being a homomorphism ... ?
Hope someone can help ...

Peter

 

[andrewkirk]

The proof does not demonstrate that $p(\overline x)=\overline{p(x)}$ *. It is left 'as an exercise for the reader'.

Here are the missing steps. We write $p(x)=\sum_{k=0}^n a_kx^k$:

\begin{align*}
p(\overline x)&=
\sum_{k=0}^n a_k\overline x^k\\
&=\sum_{k=0}^n a_k\pi(x)^k\\
&=\pi\left(\sum_{k=0}^n a_kx^k\right)\\
&\quad\quad\quad\textrm{[HERE we have used the homomorphism property to move the additions and multiplications inside the }\pi\textrm{ operator}]\\
&=\left(\sum_{k=0}^n a_kx^k\right) + (p(x))\\
&=p(x)+(px(x))\\
&=(p(x))\\
&=0_K
\end{align*}

* Indeed, depending on how one interprets the author's statement that $\overline x=\pi(x)$, the symbol string $\overline{p(x)}$ may not even be defined.

 

[Math Amateur]

The proof does not demonstrate that $p(\overline x)=\overline{p(x)}$ *. It is left 'as an exercise for the reader'.

Here are the missing steps. We write $p(x)=\sum_{k=0}^n a_kx^k$:

\begin{align*}
p(\overline x)&=
\sum_{k=0}^n a_k\overline x^k\\
&=\sum_{k=0}^n a_k\pi(x)^k\\
&=\pi\left(\sum_{k=0}^n a_kx^k\right)\\
&\quad\quad\quad\textrm{[HERE we have used the homomorphism property to move the additions and multiplications inside the }\pi\textrm{ operator}]\\
&=\left(\sum_{k=0}^n a_kx^k\right) + (p(x))\\
&=p(x)+(px(x))\\
&=(p(x))\\
&=0_K
\end{align*}

* Indeed, depending on how one interprets the author's statement that $\overline x=\pi(x)$, the symbol string $\overline{p(x)}$ may not even be defined.

Thanks for the help, Andrew ...

Just now reflecting on what you have written... but at first sight, it seems very clear ...

Thanks again,

Peter

 

[Math Amateur]

Hi Andrew,

Thanks for you help ... BUT ... just a further issue on this topic ...

Why do we need to consider a mapping/homomorphism at all in proving that $p( \overline{x} ) = \overline{ p(x) }$ ...

Surely we can just prove that $p( \overline{x} ) = \overline{ p(x) }$ in the field $F[x] / ( p(x) )$ by simply considering the nature of the cosets of the quotient ... and in particular the rules for adding and multiplying cosets ...

I wonder whether we need $\pi$ at all in the proof of Theorem 3 ...

Can you comment ... ?

Peter

 

[andrewkirk]

Surely we can just prove that $p( \overline{x} ) = \overline{ p(x) }$ in the field $F[x] / ( p(x) )$ by simply considering the nature of the cosets of the quotient ... and in particular the rules for adding and multiplying cosets ...

The difficulty is that $\overline x$ is defined as $\pi(x)$, so we cannot even state the claim $p( \overline{x} ) = \overline{ p(x) }$, let alone prove it, unless we have defined $\pi$.

Also, recall that the key claim of Theorem 3 is that $K$ contains an isomorphic copy of $F$. The easiest way to demonstrate that is to create an injective homomorphism into $K$ from a field that is isomorphic to $F$. That injective homomorphism is $\pi|_{F'}$ where $F'$ is the subring of $F[x]$ consisting of polynomials of degree 0, which is isomorphic to $F$.

So we have $F\cong F'\cong Im\ \pi|_{F'}$. In the book they identify $F'$ with $F$ but I find it clearer in this context to highlight the difference.