Splitting Fields: Anderson and Feil, Theorem 45.5 ....

[Math Amateur]

I am reading Anderson and Feil - A First Course in Abstract Algebra.

I am currently focused on Ch. 45: The Splitting Field ... ...

I need some help with some aspects of the proof of Theorem 45.5 ...

Theorem 45.5 and its proof read as follows:

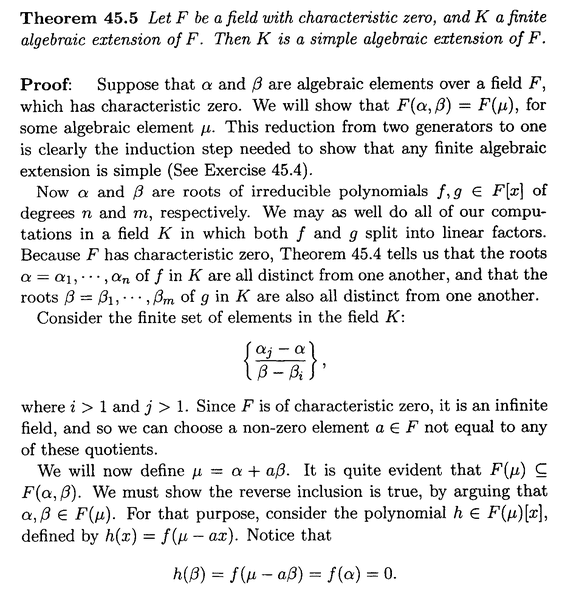
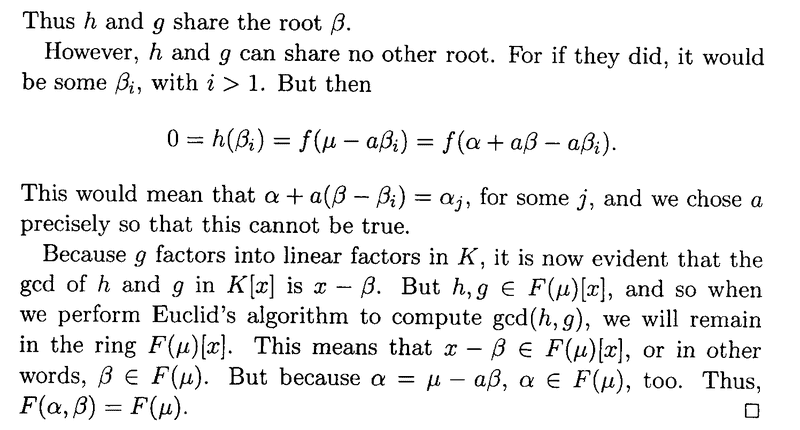
In the above text from Anderson and Feil we read the following:

"... ... Now $\alpha$ and $\beta$ are roots of irreducible polynomials $f, g \in F[x]$ ... ...Now, we are just given that \alpha and \beta are algebraic elements of a field $F$ ... ... how, exactly, do we know that they are roots of irreducible polynomials in $F[x]$... .,.. ?
"( NOTE: A&F's definition of algebraic over $F$ does not mention irreducible polynomials but says:

"If $E$ is an extension field of a field $F$ and $\alpha \in E$ is a root of a polynomial in $F[x]$, we say $\alpha$ is algebraic over $F$. ...)

Hope someone can help ...

PeterEdit: Hmm ... wonder if Kronecker's Theorem has something to do with irreducible polynomials entering this discussion ...

 

[fresh_42]

Now, we are just given that $\alpha$ and $\beta$ are algebraic elements of a field $F$ ... ... how, exactly, do we know that they are roots of irreducible polynomials in $F[x]$ ... .,.. ?

"If $E$ is an extension field of a field $F$ and $\alpha \in E$ is a root of a polynomial in $F[x]$, we say $\alpha$ is algebraic over $F$. ...)

What happens, if you consider a polynomial $p(x) \in F[x]$ that is reducible, say $p(x)=f(x)\cdot g(x)$ and $p(\alpha)=0\,$? Remember that $\operatorname{char} F =0$.

 

[Math Amateur]

What happens, if you consider a polynomial $p(x) \in F[x]$ that is reducible, say $p(x)=f(x)\cdot g(x)$ and $p(\alpha)=0\,$? Remember that $\operatorname{char} F =0$.

... ... well ... I guess $\alpha$ must be a root of either $f$ or $g$ ... and if it is a root of $f$ then $f$ is irreducible ... if it is not just continue your process until we end up with an irreducible ... so we end up with an irreducible polynomial ... so, given this, assume one at the start

Is that correct ...?

Peter

 

[fresh_42]

... ... well ... I guess $\alpha$ must be a root of either $f$ or $g$ ... and if it is a root of $f$ then $f$ is irreducible ... if it is not just continue your process until we end up with an irreducible ... so we end up with an irreducible polynomial ... so, given this, assume one at the start

Is that correct ...?

Peter

Yes, this is true. You just can assume a minimal (and irreducible) polynomial. I wonder how it is, if the characteristic is not zero, then we cannot conclude that either $f(\alpha)=0$ or $g(\alpha)=0$. At least not as easy, but I don't remember this special case.

Edit: I think I got it. If $\operatorname{char} F = p$ and $f(\alpha)\cdot g(\alpha)=0$ then $p$ divides this product, and the property of $p$ being a prime means it divides one of the factors, which in return translates to $f(\alpha)=0$ or $g(\alpha)=0$.

 

[Math Amateur]

Yes, this is true. You just can assume a minimal (and irreducible) polynomial. I wonder how it is, if the characteristic is not zero, then we cannot conclude that either $f(\alpha)=0$ or $g(\alpha)=0$. At least not as easy, but I don't remember this special case.

Thanks fresh_42 ... ...

Hmm ... very interesting point ... cannot see reason yet ... reflecting ...

Thanks again ...

Peter

 

[fresh_42]

Thanks fresh_42 ... ...

Hmm ... very interesting point ... cannot see reason yet ... reflecting ...

Thanks again ...

Peter

No, not really. Read my edit.

 

[Math Amateur]

No, not really. Read my edit.

... oh ... OK ... yes ...

Peter

 

[Math Amateur]

... oh ... OK ... yes ...

Peter

Hi fresh_42 ...

Just confirming something ...

You write:"... ... If $\operatorname{char} F = p$ and $f(\alpha)\cdot g(\alpha)=0$ then $p$ divides this product... ... "

I am assuming that $p$ divides $f(\alpha)\cdot g(\alpha)$ because $p \cdot 1 = f (\alpha)\cdot g(\alpha) = 0$ ... ...Is that correct?

Peter

 

[fresh_42]

Hi fresh_42 ...

Just confirming something ...

You write:"... ... If $\operatorname{char} F = p$ and $f(\alpha)\cdot g(\alpha)=0$ then $p$ divides this product... ... "

I am assuming that $p$ divides $f(\alpha)\cdot g(\alpha)$ because $p \cdot 1 = f (\alpha)\cdot g(\alpha) = 0$ ... ...Is that correct?

Peter

Yes, this is correct. If $\operatorname{char}F = p$, then $p=\operatorname{char}F = \underbrace{1+\ldots +1}_{p-times} =0$ in this field. Think of $\mathbb{Z}_2$ or $\mathbb{Z}_3$ where the elements are $\{0,1\}\, , \,\{0,1,2\},$ resp., which means $1+1=2=0$ in the first case and $1+1+1=3=0$ in the second case.

 

[Math Amateur]

Thanks for all your help and support on the above posts!

Most grateful for your help!

Peter