Tangent vector fields and covariant derivatives of the 3-sphere

[docnet]

Homework Statement

compute the metrics, Lie brackets, and covariant derivatives in the Levi - Civita connection of the 3-sphere

Relevant Equations

{x^2+y^2+u^2+v^2=1}

This week, I've been assigned a problem about a 3-sphere. I am confused how to approach this problem and any comments would be greatly appreciated.

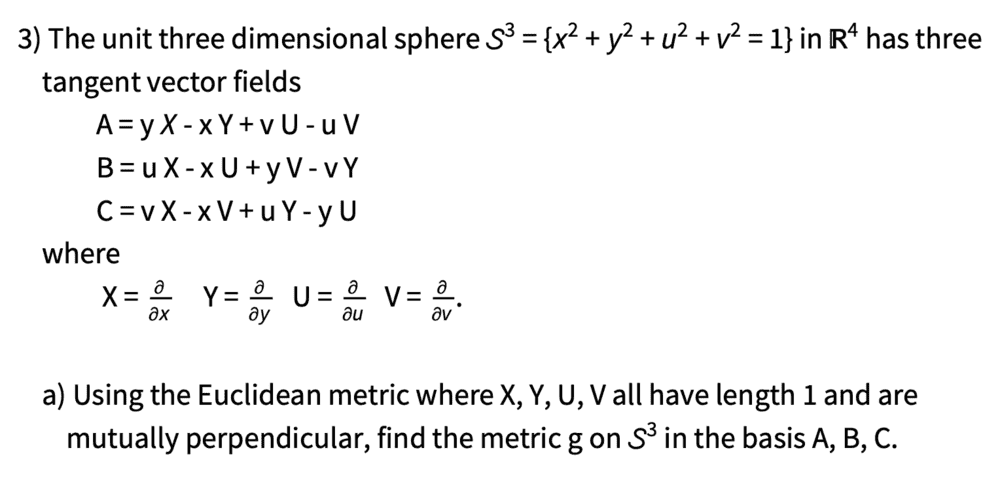
(a) - would I be correct to assume the metric G is simply the dot product of two vector fields with dx^2 dy^2 du^2 and dv^2 next to their corresponding terms?

for example:

g(A,A) = (ydx)^2 + (xdy)^2 + (vdu)^2 + (udv)^2

g(A,B) = (uv)dx^2 + (xdy)^2 + vy(du)^2 + uv(dv^2)

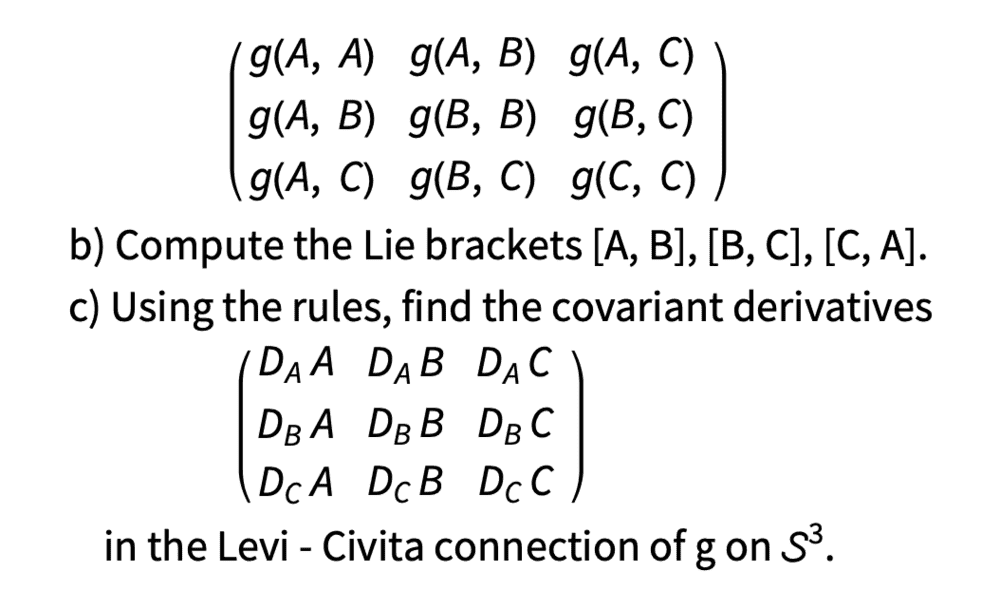
why are the metric arranged in a 3x3 pattern?(b) computing the lie brackets with the chain rule

[A,B] = operate on B with A - operate on A with B
[B,C] = operate on C with B - operate on B with C
[A,C] = operate on C with A - operate on A with C

are these computations entirely independent of the metric g?

(c) does this part involve information from the Lie brackets?thank you in advance for ideas.

 

[docnet]

anyone?

 

[Infrared]

a) No. The inner product of two vectors should be a number at each point (shouldn't have terms like $dx^2$). The vector fields $X,Y,U,V$ are orthonormal, so to compute $g(A,B),$ you should just expand and use linearity. If $e_1,\ldots,e_n$ is a basis for a tangent space of a Riemannian manifold, you can define the matrix $g_{ij}:=g(e_i,e_j)$. It's standard to represent bilinear forms by matrices like this.

b) Yes, Lie brackets of vector fields are independent of any metric.

c) I think the point is just that knowing the Lie brackets let's you save time by using the fact $\nabla_XY-\nabla_YX=[X,Y],$ so three of the covariant derivatives come "for free" once you've done the others.

 

[docnet]

a) No. The inner product of two vectors should be a number at each point (shouldn't have terms like dx2). The vector fields X,Y,U,V are orthonormal, so to compute g(A,B), you should just expand and use linearity. If e1,…,en is a basis for a tangent space of a Riemannian manifold, you can define the matrix gij:=g(ei,ej). It's standard to represent bilinear forms by matrices like this.

b) Yes, Lie brackets of vector fields are independent of any metric.

c) I think the point is just that knowing the Lie brackets let's you save time by using the fact ∇XY−∇YX=[X,Y], so three of the covariant derivatives come "for free" once you've done the others.

Thank you for the reply. I really appreciate it.

So we can relate the inner product of two vectors A and B to the metric g(A,B)?

Is the metric a function like this?

g(A, B)
= uy - xy + y^2 - vy - ux + x^2 - yx + vx + uv - vx + yv - v^2 - u^2 + ux -uy +uv
= x^2 +y^2 -u^2 -v^2 -2xy +2uv

or a matrix like this?

g(A, B) =

uy -yx y^2 -yv
-ux x^2 -xy vx
uv -vx vy -v^2
-u^2 ux -uy uvTo compute the covarient derivatives in the Levi-civita connection, should we use the metric to compute the christoffel symbols?

If the derivatives are covarient, will the Lie brackets be 0?

thank you,

 

[docnet]

For now, I'm reading up on coursework and trying to understand the concepts.. I'll post an update when I solved the problem or gotten closer.

 

[docnet]

We are posting the solution below, please type something if you see an error, thanks.

(3a)

The metric g in the basis A, B, C is a symmetric 3 x 3 matrix, with entries the inner (dot) products of the basis vectors.

y^2 + x^2 + v^2 + u^2_______ uy + x^2 + vy + uv_______ vy + x^2 + uv + uy

uy + x^2 + vy + uv_______ u^2 + x^2 + uy + vy_______ uv + x^2 + uy + vy

vy + x^2 + uv + uy_______ uv + x^2 + uy + vy_______v^2 + x^2 + u^2 + y^2

(b)

Each lie bracket [A, B] is computed by taking the derivative in the direction A of B, derivative in the direction B of A, and taking the difference. One vector operates on the other using the chain rule, with 12/16 terms in each derivative coming out as 0.

[A, B] = 2v+2u-2y-2x
[B, C] = 2y-2x-2u+2v
[A, C] = 2x+2y+2u-2v

(c)

The covariant derivatives in the Levi-Civita connection are the ordinary derivatives in the flat Euclidian connection. This is because our basis vector fields A, B, C are linear combinations of the Euclidian Basis vectors X, Y, U, V. This means we computed most the derivatives while calculating the lie brackets. Except the derivative A in the direction of A, B in the direction B, and C in the direction C. After computing these, we obtain the nine ordinary derivatives of g.

-x-y-u-v_______ v-y-x+u_______ -u-y+v+x

-v-u+y+x_______ -x-u-v-y _______ y-u-x+v

u-v-x+y _______ -y-v+u+x _______ -x-v-y-u

 

[fresh_42]

You should not omit the basis vectors and express the results in terms of $A,B,C$. What I mean is: $[A,B]=2(vX+uY-yU-xV)=2C.$

 

[docnet]

You should not omit the basis vectors and express the results in terms of A,B,C. What I mean is: [A,B]=2(vX+uY−yU−xV)=2C.

Much thanks!

The answer is then,

(b)

[A, B] = 2(vX - uY - yU - xV) = 2C
[B, C] = 2(yX - xY + vU -uV) = 2A
[A, C] = 2(uX - vY - xU + yV) = 2B(c)

-xX-yY-uU-vV_______ vX+uY-yU-xV_______ -uX+vY+xU-yV

-vX-uY+yU+xV_______ -xX-yY-uU-vV _______ yX-xY+vU-uV

uX-vY-xU+yV _______ -yX+xY-vU+uV _______ -xX-yY-vV-uUor

-xX-yY-uU-vV_______ C_______________________-B
-C____________________-xX-yY-uU-vV___________A
B_____________________-A_______________________-xX-yY-uU-vV