- #1
- 21,911
- 6,338
https://www.technologyreview.com/2019/12/06/131673/a-new-way-to-make-quadratic-equations-easy/
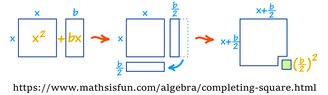
An interesting article about solving ax2 + bx + c = 0 = (x-R)(x-S), where R and S are the roots.
## x = \frac{-b ± \sqrt{b^2 - 4ac}}{2a} ##
In my classes, we were never 'spoon fed' any formula, but rather, we worked through a derivation.
An interesting article about solving ax2 + bx + c = 0 = (x-R)(x-S), where R and S are the roots.
## x = \frac{-b ± \sqrt{b^2 - 4ac}}{2a} ##
In my classes, we were never 'spoon fed' any formula, but rather, we worked through a derivation.