Understanding Compactness in ##R^2##: Analyzing Boundaries and Open Disks

[FallenApple]

Say I have a disk in $R^2$. How would I know if it is compact? I mean, if the disk has no boundary, then we can have a limit that is outside the set. On the other hand, a disk with a boundary contains all limit points. But this seems unsatisfactory as for the open disk, we are assuming that the limit is outside the set. If we ignore the embedding space and look only at the universe of the disk, then one wouldn't even be able to speak of approaching a point outside the set.

So I tried to analyze it from using the definition of compact set.

A subset

of a topological space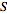

is compact if for every open cover of

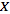there exists a finite subcover of

.

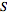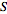
If the set of all points in the disk is in the union of some finite number of open sets, which holds for every open cover of the disk, then by definition, the set is compact.

But I don't see how this would account for whether the disk has a boundary or not. We know a open disk isn't compact intuitively. Yet, how does the boundary arise from the definition of compactness? I mean, all we have are all the possible finite subcovers of the disk. We have nothing but open sets covering the set. But how would a closed set such as the closed disk arrive from such a definition. What is the point of having finite covers then?

 

[fresh_42]

If $X=(0,1)$, is $\bigcup (\frac{1}{n},1)$ an open coverage? This makes it different from $[0,1]$. The general concept is a manifold with boundary, which borrows the boundary concept from the boundary of its charts in the surrounding Euclidean space.

 

[FallenApple]

If $X=(0,1)$, is $\bigcup (\frac{1}{n},1)$ an open coverage? This makes it different from $[0,1]$. The general concept is a manifold with boundary, which borrows the boundary concept from the boundary of its charts in the surrounding Euclidean space.

So the unions, $\bigcup (\frac{1}{n},1)$ cannot contain $X=(0,1)$? Makes sense since $(0,1)$ is bottomless in the rationals. But in the case of $[0,1]$ I can take infinite union, $\bigcup (\frac{1}{n},1)$, using all n, and it will cover $[0,1]$, if we operate with the assumption that 1/infinity=0

 

[fresh_42]

So the unions of $\bigcup (\frac{1}{n},1)$ cannot contain $X=(0,1)$?

It equals $X$.

But in the case of $[0,1]$ I can take infinite union of $\bigcup (\frac{1}{n},1)$, using all n, and it will cover $[0,1]$, if we operate with the assumption that 1/infinity=0

This is no assumption, this is simply not allowed. The $0$ will always be missing, and if we add a set which contains it, we will also have a finite sub-coverage.

 

[FallenApple]

It equals $X$.

This is no assumption, this is simply not allowed. The $0$ will always be missing, and if we add a set which contains it, we will also have a finite sub-coverage.

Oh ok got it. The equality is synonymous with the left limit approaching 0 but never attaining zero. And the set $[0,1]$ is the finite subcover that contains $(0,1)$ and itself since $[0,1]\cup\bigcup (\frac{1}{n},1)={{0}}\cup{{1}}\cup\bigcup (\frac{1}{n},1)=[0,1]$ So It is that set that contains $(0,1)$ and is the boundary. But it's not just any finite sub cover. It is the bare minimum sub cover. And this bare minimum sub cover is the boundary?

 

[fresh_42]

In any open cover of $[0,1]$ - at least with the standard topology - there is a finite subcover. Which one depends on how the cover is given. Therefore $[0,1]$ is compact. $(0,1)$ is not compact, since $\bigcup (\frac{1}{n},1)$ is a cover of $(0,1)$ which doesn't have a finite subcover. This is also true as subsets of $\mathbb{R}$.

 

[WWGD]

In $\mathbb R^2$ or in $\mathbb R^n$ for finite $n$ compactness coincides (iff) being closed and bounded. This is Heine-Borel. You can look up proofs.

 

[FallenApple]

In any open cover of $[0,1]$ - at least with the standard topology - there is a finite subcover. Which one depends on how the cover is given. Therefore $[0,1]$ is compact. $(0,1)$ is not compact, since $\bigcup (\frac{1}{n},1)$ is a cover of $(0,1)$ which doesn't have a finite subcover. This is also true as subsets of $\mathbb{R}$.

When you say standard topology, it means $\mathbb{R}$ equipped with the Euclidean metric? It make sense that $\bigcup (\frac{1}{n},1)$ has no finite sub cover since using the Euclidean metric, any smaller interval would not cover, but the idea of not covering is predicated on the notion of Euclidean distance working the way it does in the first place. So compactness is metric dependent?

 

[WWGD]

When you say standard topology, it means $\mathbb{R}$ equipped with the Euclidean metric? It make sense that $\bigcup (\frac{1}{n},1)$ has no finite sub cover since using the Euclidean metric, any smaller interval would not cover, but the idea of not covering is predicated on the notion of Euclidean distance working the way it does in the first place. So compactness is metric dependent?

It is Topology dependent and therefore, if you are using the topology "induced" by a metric, it is metric-dependent. It depends on the open sets you are working with.

 

[FallenApple]

It is Topology dependent and therefore, if you are using the topology "induced" by a metric, it is metric-dependent. It depends on the open sets you are working with.

Does the set of real numbers have no topology if no metric is specified? Without a metric, the reals is just an uncountable set of points.

 

[WWGD]

Does the set of real numbers have no topology if no metric is specified?

If you want to talk about Compactness, you need to have a topology defined; Compactness is a topological property. I would say the default topology on the Reals is the metric topology, but you can always define a topology as/if you wish. EDIT: Often you want the choice of topology to agree with other type of structure, depending on the situation at hand.

 

[FallenApple]

If you want to talk about Compactness, you need to have a topology defined; Compactness is a topological property. I would say the default topology on the Reals is the metric topology, but you can always define a topology as/if you wish.

Ah ok. I could take the powerset, which satisfies all 3 axioms. That would give me a topology. But it wouldn't behave in anyway like the reals with the Euclidean metric, since it wouldn't even be a line. So all along, in Analysis, when the reals are spoken of, it's not just the reals, it's the reals with the usual Euclidean metric. That gives it the continuous geometric structure, via open intervals obtained using the metric.

 

[WWGD]

Ah ok. I could take the powerset, which satisfies all 3 axioms. That would give me a topology. But it wouldn't behave in anyway like the reals with the Euclidean metric, since it wouldn't even be a line. So all along, in Analysis, when the reals are spoken of, it's not just the reals, it's the reals with the usual Euclidean metric. That gives it the continuous geometric structure, via open intervals obtained using the metric.

Exactly. It is an exercise in old-school Math ( meaning 50s, 60s) to find the number of topologies definable in a given set/space (giving you different chains of inclusion). Maybe useful in areas like Functional Analysis, but not , AFAIK, beyond that.