Why does a symmetric wavefunction imply the angular momentum is even?

[mjmnr3]

Homework Statement

1.8 Consider the strong interaction $\pi^{-} d \rightarrow n n,$ where $d$ is a spin- 1 S-wave bound state of a proton and a neutron called the deuteron and the initial pion is at rest. Deduce the intrinsic parity of the negative pion.

Relevant Equations

$$\hat{P}\psi_{nlm}=p(-1)^l \psi_{nlm}$$

I looked in the instructor solutions, which are given by:

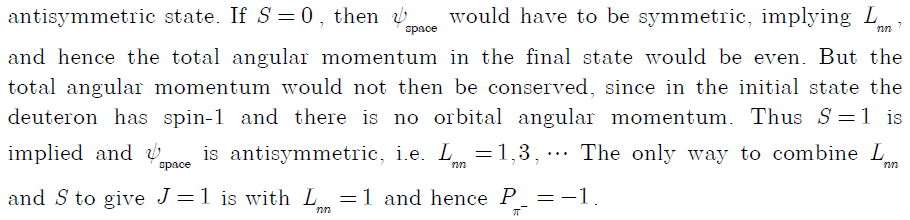
But I don't quite understand the solution, so I hope you can help me understand it.

First. Why do we even know we are working with wavefunctions with the quantum numbers n,l,m? Don't we only get these quantum numbers if the particles we're working with, is in a spherical symmetric potential?
So in other words, why do we need to consider the quantum number l (angular momentum)? Is that because strong force gives rise to a spherical potential?

When using the parity operator, why do we get the the combined angular momentum $(-1)^{L_{\pi d}}$ and not just: $(-1)^{L_{\pi}}\cdot (-1)^{L_d}$. Can I always just couple the angular momentum when working with multiple particle wavefunctions, and assume operators only works on the coupled angular momentum?

The most important question, is how the angular momentum relates to the symmetry of a wave function.
Why does a symmetric space wavefunction $\psi_{space}=symmetric$ implies that the angular momentum $L_{nn}$ has to be even?
And the opposite. Why does an antisymmetric wavefunction imply uneven angular momentum?

 

[DrClaude]

First. Why do we even know we are working with wavefunctions with the quantum numbers n,l,m? Don't we only get these quantum numbers if the particles we're working with, is in a spherical symmetric potential?
So in other words, why do we need to consider the quantum number l (angular momentum)? Is that because strong force gives rise to a spherical potential?

I don't see n, l, and m in there. Rather, you have particles scattering off each other, for which the potential is going to be spherically symmetric, and the overall scattering process will have cylindrical symmetry. It is usual to treat such problem by decomposing the angular part of the wave function into spherical harmonics $Y_{lm}$.

When using the parity operator, why do we get the the combined angular momentum $(-1)^{L_{\pi d}}$ and not just: $(-1)^{L_{\pi}}\cdot (-1)^{L_d}$. Can I always just couple the angular momentum when working with multiple particle wavefunctions, and assume operators only works on the coupled angular momentum?

You are considering the entire system, so you must take into account the total angular momentum of the pion-deuteron system.

The most important question, is how the angular momentum relates to the symmetry of a wave function.
Why does a symmetric space wavefunction $\psi_{space}=symmetric$ implies that the angular momentum $L_{nn}$ has to be even?
And the opposite. Why does an antisymmetric wavefunction imply uneven angular momentum?

The final state is two neutrons, so two identical fermions. By the Pauli principle, the total wave function must be anti-symmetric.

 

[vela]

The most important question, is how the angular momentum relates to the symmetry of a wave function.
Why does a symmetric space wavefunction $\psi_{space}=symmetric$ implies that the angular momentum $L_{nn}$ has to be even? And the opposite. Why does an antisymmetric wavefunction imply uneven angular momentum?

It's because of the parity of the spherical harmonics: $\hat P Y_l^m(\theta,\phi) = Y_l^m(\pi-\theta,\phi+\pi) = (-1)^l Y_l^m(\theta,\phi)$.

 

[mjmnr3]

It's because of the parity of the spherical harmonics: $\hat P Y_l^m(\theta,\phi) = Y_l^m(\pi-\theta,\phi+\pi) = (-1)^l Y_l^m(\theta,\phi)$.

But I believe there is a distinction between a spatial symmetric/even function ($\hat{P} \psi_{nlm}=+\psi_{nlm}$, for even $l$) and a symmetric wavefunction?

Pauli's exclusion principle tells us that the total wavefunction should be antisymmetric for identical fermions. So if the spin wavefunction is antisymmetric, the spatial wavefunction needs to be symmetric. But what a symmetric wave function means, is just that it needs to satisfy: $$\psi(\vec{r_1},\vec{r_2})=\psi(\vec{r_2},\vec{r_1})$$ Where $\vec{r_1},\vec{r_2}$ is the position vectors for particle 1 and 2, respectively.

But that kind of symmetry is not directly related to the parity and thus a spatial symmetric wavefunction?

 

[mjmnr3]

I don't see n, l, and m in there. Rather, you have particles scattering off each other, for which the potential is going to be spherically symmetric, and the overall scattering process will have cylindrical symmetry. It is usual to treat such problem by decomposing the angular part of the wave function into spherical harmonics $Y_{lm}$.You are considering the entire system, so you must take into account the total angular momentum of the pion-deuteron system.The final state is two neutrons, so two identical fermions. By the Pauli principle, the total wave function must be anti-symmetric.

Thank you very much for your reply. What I meant by "n,l,m" is that I have only seen how the angular momentum $l$ appears in the parity operator when acting on wavefunctions as solutions to a spherically symmetric potential. And typically the quantum number $l$ arises when solving the Schrodinger equation for a spherically symmetric potential.

So if the wavefunctions were solutions to an asymmetric potential, I believe it would be wrong to assume, that we would be working with the same quantum numbers $n,l,m$ and that the parity operator would act the same way, as we see with solutions to a symmetric potential.

Note, that I'm not saying that particles in asymmetric potentials don't have angular momentum. Just that I wouldn't know if solutions to such a Hamilton would have the same type of quantum numbers, and how the parity operator would work on such a system.

 

[vela]

But I believe there is a distinction between a spatial symmetric/even function ($\hat{P} \psi_{nlm}=+\psi_{nlm}$, for even $l$) and a symmetric wavefunction?

It's been awhile for me, so I could be misremembering. I'm sure someone will correct me if that's the case.

The potential for the interaction depends on $\lvert \vec r_1 - \vec r_2 \rvert$, so you can analyze the interaction in terms of the center of mass of the system and the relative position of the particles, $\vec r = \vec r_1 - \vec r_2$. Exchanging identical particles then is the same as replacing $\vec r$ by $-\vec r$. Hence, a symmetric wave function is one which is even parity, and an antisymmetric wave function is one that is odd parity.

 

[vanhees71]

The last point is now to also take into account the spin state! In other words, think about what means that the d is a "1S bound state" concerning the symmetry properties of the spin?