Why is Bernoulli's Equation Isentropic

[Tom79Tom]

I have trouble understanding why we classify an inviscid adiabatic incompressible flow along a streamline as isentropic
I understand this from a Thermodynamic definition/explanation
$$dS = dQ/T$$
Adiabatic Invsicid
$$dQ =0= dS$$
So no heat added or lost no change in entropy I'm fine with that

From a Boltzman definition I am less clear how is it possible that we can have additional gradients within in the volume and more ordered momentum yet have no change in available entropy

$$S=k_BlnW$$If we looked at a two different control volumes a straight pipe and a venturi separating a pressure difference

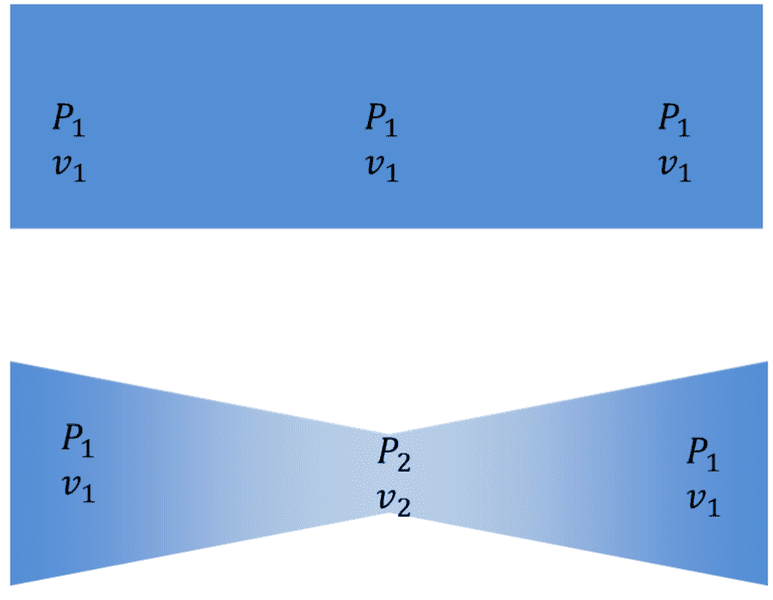
In an inviscid adiabatic flow, Starting pressure P₁ and final pressure P₁ are the same and equal , the flow rate Q in and out of the volume are the same but the presence of the Venturi causes additional gradients P₁ to P₂ and an increase in ordered kinetic energy from v₁ to v₂To me follow that this is a reduction in available microstates ∑{x,y,z,px,py,pz} compared to an inviscid flow without a venturi.

Understanding that no flow is not in thermodynamic equilibrium and that within each example flow the sum of microstates is constant so Entropy within the flow is constant how can we say that these flows have the same available microstates and entropy ?

Wouldn't it be better to say that it is a reversible isochoric process between differing macrostates along the flow ?

[1]: https://i.stack.imgur.com/N9lfB.png

 

[Chestermiller]

I don't know how to do this in terms of statistical thermodynamics, but, in terms of classical thermodynamics, maybe this will help.

The open-system (control volume) version of the first law of thermodynamics is derived directly from the usual closed-system version (no mass in or out). For steady state flow of a fluid through a control control volume (such as the control volume shown in your second figure), the first law reduces to:
$$\dot{Q}-\dot{W_s}=\dot{m}(\Delta h+\Delta \frac{v^2}{2}+g\Delta z)$$where $\dot{Q}$ is the rate of adding heat to the control volume, $\dot{W_s}$ is the rate of doing shaft work (work other than that required to push fluid into and out of the control volume), $\dot{m}$ is the rate of fluid flow into and out of the control volume, h is the enthalpy per unit mass of the flowing fluid, v is the fluid velocity, and z is the elevation. The $\Delta$ refers to the change between the inlet and outlet of the control volume.

For the adiabatic system shown in your second figure, the rate of heat addition and of doing shaft work are zero, so the first law equation reduces simply to: $$\Delta h+\Delta \frac{v^2}{2}+g\Delta z=0$$
This equation applies not only to the overall control volume but also to all smaller sections (which can likewise be regarded as control volumes) between the inlet and outlet on your second figure. Therefore, we can also write:$$dh+d\left(\frac{v^2}{2}\right)+gd z=0$$
Now, for dh between neighboring cross sections is expressible as: $$dh=Tds+\frac{dP}{\rho}$$where $\rho$ is the fluid density (which is the reciprocal of the specific volume). So, combining these relationships, we obtain:
$$Tds+\frac{dP}{\rho}+d\left(\frac{v^2}{2}\right)+gd z=0$$
If the flow is inviscid, such that the rate of generation of entropy within the differential control volume is zero (ds = 0), we are left with the Bernoulli equation: $$d\left(\frac{P}{\rho}+\frac{v^2}{2}+g z\right)=0$$
This equation tells us that, in the absence of viscous dissipation (i.e., entropy generation), mechanical energy is conserved.

Hope this helps a little.

 

[Tom79Tom]

Hi Chet, thanks so much for your explanation, always appreciate the time people on this site are willing to take to enlighten us.

I guess the stumbling block i see is that within the derivation is the same assumption (Q =0, W=0 ds=0) and I'm completely fine that entropy exchange across the boundary is zero , however internally within the flow there must be an exchange to create the less probable more ordered state in the constriction?

If I could ask another question ,
If the sum of Entropy in a flow or control volume is constant does it also hold that every point/parcel along an adiabatic invisicd streamline has the same entropy ?

To me the work done within the control volume to accelerate a parcel is a decrease in Entropy internally within the flow and the work done against the parcel is to slow it is an increase in entropy internally within the flow.

Under adiabatic inviscid conditions these variations in Entropy cancel each other and it holds that the total flow can be considered Isentropic
but is it fair to say an internal variation/exchange between neighboring parcels occurs?

 

[Chestermiller]

Hi Chet, thanks so much for your explanation, always appreciate the time people on this site are willing to take to enlighten us.

I guess the stumbling block i see is that within the derivation is the same assumption (Q =0, W=0 ds=0) and I'm completely fine that entropy exchange across the boundary is zero , however internally within the flow there must be an exchange to create the less probable more ordered state in the constriction?

If I could ask another question ,
If the sum of Entropy in a flow or control volume is constant does it also hold that every point/parcel along an adiabatic invisicd streamline has the same entropy ?

Every parcel along an adiabatic inviscid streamline has constant entropy.

To me the work done within the control volume to accelerate a parcel is a decrease in Entropy internally within the flow and the work done against the parcel is to slow it is an increase in entropy internally within the flow.

No. Think of a spring-mass system. The driving force (analogous to pressure in the fluid), the kinetic energy, and the elastic energy of the spring (analogous to gravitational energy in the fluid), interact so that no mechanical energy is lost, and the system will exhibit simple harmonic motion forever. So no entropy is generated in such a system. But, if you add a viscous damper to the mixture, entropy is generated within the viscous damper as a result of dissipation of the mechanical energy to internal energy.

Under adiabatic inviscid conditions these variations in Entropy cancel each other and it holds that the total flow can be considered Isentropic
but is it fair to say an internal variation/exchange between neighboring parcels occurs?

No. No entropy is generated anywhere within the control volume.

Here's how it works. You are correct about no entropy entering or leaving through the external boundaries or across neighboring streamlines forming a flow channel for the train of parcels. However, the mechanism for entropy generation within the parcels of a real fluid is the result of viscous dissipation of mechanical energy; this viscous dissipation is produced by deformation of the fluid in conjunction with viscous stresses. If the fluid viscosity approaches zero, viscous stresses and viscous dissipation both approach zero, and no entropy is generated. Here is the actual equation for the local viscous rate of entropy generation per unit volume within the flow: $$G=\frac{2\eta (\vec{E}:\vec{E})}{T}$$where $\vec{E}$ is the local rate of deformation tensor within the fluid, T is the local tempeature, and $\eta$ is the local viscosity. The symbol : in the equation represents the double dot tensor product (double contraction). According to this equation, the viscous rate of entropy generation is positive definite. Note that, even if the fluid is deforming, if $\eta$ is zero, G is locally zero everywhere in the flow.

For an excellent discussion of entropy generation in a fluid flow, see Transport Phenomena by Bird, Stewart, and Lightfoot, Chapter 11, page 372, Problem 11D.1 Equation of change for entropy. This provides wonderful insight.

Also, the following link supplements what I said: http://physics.stackexchange.com/questions/306482/is-viscosity-a-consequence-of-entropy

 

[Tom79Tom]

No. Think of a spring-mass system. The driving force (analogous to pressure in the fluid), the kinetic energy, and the elastic energy of the spring (analogous to gravitational energy in the fluid), interact so that no mechanical energy is lost, and the system will exhibit simple harmonic motion forever. So no entropy is generated in such a system

Thanks Chester . I think i have it , so in the absence of a damper (viscous dissapation) the conversion between potential energy and kinetic energy within a fluid is in itself always an isentropic process as no mechanical energy is lost.

I guess I am concentrating too much on the view of a more ordered, useful (can do more work) state when kinetic energy has increased -
$$W=ΔKe$$

To counter that argument I suppose we say the stagnation pressure~ is the same at all points so the amount of work the fluid could do is unchanged.
$$W=ΔKe+ΔPe=0$$
Does that hold ?
As a further question would it still hold in the viscous case (where shear forces would increase with increase KE), that the increase KE can do no more work ?

 

[Chestermiller]

Thanks Chester . I think i have it , so in the absence of a damper (viscous dissapation) the conversion between potential energy and kinetic energy within a fluid is in itself always an isentropic process as no mechanical energy is lost.

I guess I am concentrating too much on the view of a more ordered, useful (can do more work) state when kinetic energy has increased -
$$W=ΔKe$$

To counter that argument I suppose we say the stagnation pressure~ is the same at all points so the amount of work the fluid could do is unchanged.
$$W=ΔKe+ΔPe=0$$
Does that hold ?
As a further question would it still hold in the viscous case (where shear forces would increase with increase KE), that the increase KE can do no more work ?

No. This is not how it plays out mechanistically. There is work being done at the inlet and the outlet to push material into and out of the control volume. So the amount of work being done is not zero. The term $P/\rho$ represents the amount of work per unit mass flowing in or out. This work alters the sum of the kinetic and potential energy (via the work-energy theorem). If you also wanted viscous effects to be accounted for, you would have to modify the Bernoulli equation to the form: $$\left(\frac{P}{\rho}+\frac{v^2}{2}+g z\right)_1=\left(\frac{P}{\rho}+\frac{v^2}{2}+g z\right)_2+D$$where D is the amount of viscous dissipation of mechanical energy per unit mass passing through the control volume (i.e., entropy generation within the control volume). For viscous flow in a tube, for example, there is an actual equation for D.

 

[Tom79Tom]

Thanks Chester
Is the first part a correct interpratation of what you are explaining ?

In the absence of a damper (viscous dissipation) the conversion between potential energy and kinetic energy within a fluid is in itself always an isentropic process- as no mechanical energy is lost.

 

[Chestermiller]

Thanks Chester
Is the first part a correct interpratation of what you are explaining ?

Yes, if you include in the first part the change in potential energy as well.

In the absence of a damper (viscous dissipation) the conversion between potential energy and kinetic energy within a fluid is in itself always an isentropic process- as no mechanical energy is lost.

Yes. Here is a link to a Physics Forums Insights article I wrote that you might find interesting: https://www.physicsforums.com/insights/reversible-vs-irreversible-gas-compressionexpansion-work/ It relates to similar irreversibilities occurring in the compression or expansion of a gas, and shows the close similarity to a spring-damper system.