2 trucks pulling a third truck by the means of a pulley

In summary: The one used to answer the question above...so x1+x2-2xp.I get that the length of the rope is x1+x2-2xp but I don't understand how you differentiated it and how that led to the...You took the double derivative of the equation x1+x2-2xp to get x1+x2-4x.
  • #1
Jkyou
6
0

Homework Statement


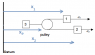
Two trucks tow a third one by means of inextensible ropes and a pulley attached to them (fig. 1). The accelerations of the two trucks are a1 and a2. What is the acceleration of the third truck that is being towed?
(fig.1) is in this link (I'm sorry, I don't know how to post images on websites):
http://www.physics.ox.ac.uk/olympiad/Downloads/PastPapers/BPhO_AS_2007_QP.pdf

m=mass of truck
a1,a2 respective accelerations
a=the shared acceleration (whole system)
T= Tension

Homework Equations


F = ma
Free body diagrams

The Attempt at a Solution


Although the problem didn't specify, I'm assuming the masses of the trucks are equal
Since the total acceleration of the two trucks must be the same,

2ma = ma1-ma2
Thus, a= (a1-a2)/2
Furthermore, ma =T - ma2 for the second truck, so

T = ma + ma2 -> T = m(a1+a2)/2

The force of the tension on the pulley and 3rd truck = 2T

And total force on the pulley, which pulls the third truck = ma

Thus ma = 2T -> a = a1+a2, but the answer key says it's a = (a1+a2)/2
 
Physics news on Phys.org
  • #2
Hello. Welcome to PF!

The accelerations of trucks 1 and 2 are not necessarily the same. This is a kinematics problem. You do not need to work with forces.

For problems like this, it is often helpful to use the fact that the total length of a rope must remain constant. Consider introducing distances from a fixed line ("datum") as shown below. Can you relate the distances x1, x2, and xp to the length of the rope connecting trucks 1 and 2?
 

Attachments

  • three trucks.png
    three trucks.png
    2.5 KB · Views: 1,073
  • #3
So that'll be length = x1 + x2 -2xp ?
 
  • #4
TSny said:
Hello. Welcome to PF!

The accelerations of trucks 1 and 2 are not necessarily the same. This is a kinematics problem. You do not need to work with forces.

For problems like this, it is often helpful to use the fact that the total length of a rope must remain constant. Consider introducing distances from a fixed line ("datum") as shown below. Can you relate the distances x1, x2, and xp to the length of the rope connecting trucks 1 and 2?

Will it be then length of rope = x1 + x2 - 2xp = constant

And perhaps take the double derivative of that?
 
  • #5
Jkyou said:
Will it be then length of rope = x1 + x2 - 2xp = constant

And perhaps take the double derivative of that?
Yes!
 
  • #6
TSny said:
Yes!
Ohh I see! That's a really elegant way to do that. Do you think it's possible with forces, just for the fun of it? Or will it be difficult to do that?
 
  • #7
Jkyou said:
Ohh I see! That's a really elegant way to do that. Do you think it's possible with forces, just for the fun of it? Or will it be difficult to do that?
The kinematic constraint is what determines the relation between the accelerations, not the forces. But, you can then use the relation between the accelerations and F = ma to relate various forces in the problem.
 
  • #8
TSny said:
The kinematic constraint is what determines the relation between the accelerations, not the forces. But, you can then use the relation between the accelerations and F = ma to relate various forces in the problem.

I see, so the accelerations are dependent on the constraint and not always on the forces situations such as this one. So for example if I were to determine the tension in the string, since 2T = ma where a must be the acceleration of the pulley, I can determine T = m(a1+a2)/4 and etc. Am I on the right track?
 
  • #9
Jkyou said:
I see, so the accelerations are dependent on the constraint and not always on the forces situations such as this one. So for example if I were to determine the tension in the string, since 2T = ma where a must be the acceleration of the pulley, I can determine T = m(a1+a2)/4 and etc. Am I on the right track?
Yes. The details would depend on what information you are given and what you are trying to determine.
 
  • #10
TSny said:
Yes. The details would depend on what information you are given and what you are trying to determine.

Ahh I see, thank you so much! I am glad I came here for help ^^
 
  • #11
OK. Glad I could help.
 
  • #12
TSny said:
Yes!
How do you take the double derivate of that and come to the answer?
 
  • #13
Seneka said:
How do you take the double derivate of that and come to the answer?
Welcome to PF!

Please write out the specific equation that you wish to differentiate.
 
  • #14
TSny said:
Welcome to PF!

Please write out the specific equation that you wish to differentiate.

The one used to answer the question above...so x1+x2-2xp.I get that the length of the rope is x1+x2-2xp but I don't understand how you differentiated it and how that led to the answer.
 
  • #15
Seneka said:
I get that the length of the rope is x1+x2-2xp but I don't understand how you differentiated it and how that led to the answer.
So the equation is L = x1 + x2 - 2xp, where L is the length of the rope. What do you get if you take one time derivative of each side?
 
  • #16
TSny said:
So the equation is L = x1 + x2 - 2xp, where L is the length of the rope. What do you get if you take one time derivative of each side?

I don't know.
 
  • #17
What does the time derivative of x1 represent?
 
  • #18
TSny said:
What does the time derivative of x1 represent?

Velocity?
 
  • #19
Seneka said:
Velocity?
Yes. So, you could write the time derivative of x1 as v1.
 
  • #20
Where are you going with this?

So, x1+x2-2xp=l
v1+v2-2vp=vl?
a1+a2-2ap=al?
a1+a2-al=2ap?
(a1+a2-al)/2=ap?
 
  • #21
Seneka said:
Where are you going with this?
Hopefully to some insight :oldsmile:

So, x1+x2-2xp=l
v1+v2-2vp=vl?
What does vl represent? The ropes are given to be "inextensible".
What does this imply about the right hand side of your equation x1+x2-2xp=l?
 
  • #22
TSny said:
Hopefully to some insight :oldsmile:

I appreciate your patience.

What does vl represent? The ropes are given to be "inextensible".
What does this imply about the right hand side of your equation x1+x2-2xp=l?

vl=0 there is no change is l
you could rearrange to get vp=(v1+v2)/2
I guess if you do the second derivative you get ap=(a1+a2)/2
Am I right?
 
  • #23
Seneka said:
vl=0 there is no change is l
you could rearrange to get vp=(v1+v2)/2
I guess if you do the second derivative you get ap=(a1+a2)/2
Am I right?
Yes. Good work.
 
  • #24
TSny said:
Yes. Good work.

OMD! I finally got there. Thanks again for your patience and quick reply! Is there a name for this technique using this 'datum' thing or is it just logic?
 
  • #25
I don't know of any name for the technique.
 

1. How does the pulley system work?

The pulley system works by using a combination of ropes and wheels to redirect the force of the pulling trucks onto the third truck. The ropes are looped around the wheels and connected to the trucks, allowing them to pull together in the same direction.

2. What is the purpose of using a pulley in this situation?

The pulley allows for the force of the pulling trucks to be distributed evenly, making it easier to pull the third truck. It also reduces the amount of force needed by the pulling trucks, making the task more efficient.

3. Is there a limit to the number of trucks that can be pulled using this pulley system?

The number of trucks that can be pulled using a pulley system is dependent on the strength of the ropes and wheels being used. If these components are strong enough, multiple trucks can be pulled.

4. How does friction affect the efficiency of the pulley system?

Friction can affect the efficiency of the pulley system by creating resistance, which can make it harder for the trucks to move. To increase efficiency, lubricants or smoother materials can be used to reduce friction.

5. Are there any safety precautions that need to be taken when using a pulley system in this way?

Yes, it is important to make sure that the ropes and wheels are in good condition and able to support the weight of the trucks. Also, proper training and supervision should be provided to ensure safe operation of the pulley system.

Similar threads

What if check: Am I calculating tension wrong?
    • Introductory Physics Homework Help
Replies
3
Views
1K
Why is tension (T) only added to one side of an Atwood Machine?
    • Introductory Physics Homework Help
Replies
3
Views
2K
Finding acceleration in multiple pulley system
    • Introductory Physics Homework Help
Replies
1
Views
5K
Understanding Newton's Third Law in Action: A Truck Pushing an SUV
    • Introductory Physics Homework Help
Replies
4
Views
1K
Laws of Motion (Pulleys, blocks)
    • Introductory Physics Homework Help
Replies
21
Views
8K
Double pulley Atwood machine (with 3 masses)
    • Introductory Physics Homework Help
3
Replies
97
Views
14K
Newton's laws of motion -- Constaint motion
    • Introductory Physics Homework Help
Replies
13
Views
4K
What is the acceleration of the blocks in a pulley constraint problem?
    • Introductory Physics Homework Help
Replies
21
Views
4K
Acceleration in a pulley system
    • Introductory Physics Homework Help
Replies
8
Views
6K
How to calculate tension in straight-line motion
    • Introductory Physics Homework Help
Replies
3
Views
3K

Hot Threads

Recent Insights

Back
Top