- #1
Engineer_Phil
- 27
- 0
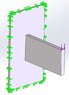
I have a design where a beam gets welded to a plate. The plate is not against a wall or foundation, but for simplicity I can say that the edges of the plate are fixed. I am concerned about the stress in the plate, and the deflection in the beam. I have access to solidworks simulation, but I want to know if anyone knows of an analytical solution or experimental solution that I can compare to. I don't like to just blindly trust a simulation.
I have attached an image of my setup. In the future I want to vary the beam cross section size and style.
I have attached an image of my setup. In the future I want to vary the beam cross section size and style.