- #71
- 2,355
- 10
BRS: Hubble expansion (right) versus "expanding Earth" (wrong)
In "Universal expansion"
Landrew admits
The Earth and Moon are very slowly moving further apart, but this is due to something else entirely. The Hubble expansion has almost no effect on the Earth-Moon system.
Because science is honest by design, as it were, scientists working at the frontiers uncover apparent inconsistencies with previous knowledge, and one of the most characteristic features of science is that science provides a powerful error-correction/inconsistency-resolution method, which may take time but seems to get us there in the end, if we simply work hard enough. One key aspect of the inconsistency-resolution method is that scientists try to make minimal changes to well-established theories in order to resolve apparent contradictions at the frontiers of scientfic knowledge. Their first attempts often involve tentative inference of the existence of something with unexpected properties, followed by attempts to verify that this stuff actually exists. This is exactly what is happening wrt dark matter and dark energy.
For more information about how science works, Landrew should see the UCB website "Misunderstandings of Science"
and Tom Bridgman's blog
Re "Schwarzschild Effective Potentials"
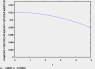
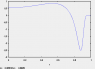
no, it is simply a function such that the roots of the derivative V'(r_c) = 0 help to organize turning points r=r_c for the radial motion of trajectories. That is, for particular values of E,L (energy and momentum of the test particle), the graph of V typically has a local minimum, and if your particle has energy E just a bit larger than that minimum, when you draw a horizontal line with height E on the graph of V, it will intersection the curve V(r) at two turning points. This means that the radial motion of the particle will oscillate between these two values. Simulataneously, of course, it is has nonzero angular motion, so the result is that a particle with suitable L, E will orbits in a quasi-elliptical trajectory which turns out not to quite return to the same location at the maximal radius--- this is the famous precession of the periastria.
Most gtr textbooks offer very clear explanations of this; see for example MTW.
In "Universal expansion"
Code:
www.physicsforums.com/showthread.php?t=449723That is exactly the problem, but he gets points for recognizing this!If a little knowledge is a confusing thing, I certainly have the prerequisites to be confused about Universal Expansion.
The language of physics is mathematical reasoning. The mathematics of cosmological models formulated in gtr is unambiguous, but gtr rests upon the mathematics of curved manifolds, which laypeople don't know anything about. Thus when physicists speak to a lay audience they must "dumb down" the truth into statements in natural language ("plain English"). In different contexts, physicists may consider different and apparently inconsistent partial reformulations in natural language to be appropriate, but laypersons should not assume from "obvious contradictions" that there is anything wrong with the actual mathematics. In particular, both of these statements intuitively capture some aspects of the actual mathematicsSome physicists seem to be saying that all the stars and galaxies are flying apart like shrapnel from a large explosion, and other physicists seem to be saying that space itself is expanding metrically, thereby accounting for the fact that the more distant the object we observe, the faster it seems to be moving away, even apparently exceeding the speed of light.
- "stars and galaxies are flying apart like shrapnel from a large explosion"
- "space itself is expanding"
That is a VCM (Very Common Misunderstanding); seeIf space itself is expanding over time, then matter itself would have to be expanding at the same rate... if the metric expansion model is correct, millions of years ago, our solar system was a smaller scale model of how it is now.
Code:
www.astro.ucla.edu/~wright/cosmology_faq.html#MXBrooklyn is not expanding. The Earth is not expanding. The Sun is not expanding. The Solar System is not expanding (much). Landrew's body is not expanding either, and he shouldn't expect otherwise, because his body is held together by chemical forces, not gravitation. It is true that planets and stars are held together by gravitation but the Sun, the Earth, and the Solar System are all more dense than the average volume in the current epoch of the Universe, and this has been true throughout their history. Thus it should not be surprising that they are almost immune to cosmological expansion, as mathematical analysis verifies. OTH, on a very large scale, distant pairs of galaxies interact only weakly with each other and these will be subject to the Hubble expansion (on top of various motions "wrt the CMB" they may possesses by chance).otherwise the Earth wouldn't have remained in the "Goldilocks Zone" which has allowed life to exist in this planet for billions of years.
The Earth and Moon are very slowly moving further apart, but this is due to something else entirely. The Hubble expansion has almost no effect on the Earth-Moon system.
Nice try, but no. The pteranodons simply had some tricks for getting into the air which the old analyses Landrew refers to did not take into account. The surface of the Earth has never differed from its current value during the 4.5 billion year history of biotic life on Earth.If our Earth was indeed smaller, the gravity of our planet would have also been less. The flying dinosaurs would have had less difficulty flying in lesser gravity. Perhaps this explains why when scientists examined their skeletons, they determined that they were built much too heavy to ever get off the ground today.
Sigh... ignorant indeed. And it is dark energy plus dark matter, not just dark matter. And these are not theories, but inferences drawn from several very well established theories (gtr, hot Big Bang theory). And radioactivity is invisible to the naked eye, but not long after its existence was inferred from chemical reactions (in photographic plates), scientists figured out how to measure the amount and nature of radioactivity from substances like Radium, thus confirming that it does exist, and later devised a now well-established theory explaining why it exists.Or is a better solution to invent a theory that 96% of our universe is invisible dark matter, to make things seem to work out?
Because science is honest by design, as it were, scientists working at the frontiers uncover apparent inconsistencies with previous knowledge, and one of the most characteristic features of science is that science provides a powerful error-correction/inconsistency-resolution method, which may take time but seems to get us there in the end, if we simply work hard enough. One key aspect of the inconsistency-resolution method is that scientists try to make minimal changes to well-established theories in order to resolve apparent contradictions at the frontiers of scientfic knowledge. Their first attempts often involve tentative inference of the existence of something with unexpected properties, followed by attempts to verify that this stuff actually exists. This is exactly what is happening wrt dark matter and dark energy.
For more information about how science works, Landrew should see the UCB website "Misunderstandings of Science"
Code:
undsci.berkeley.edu/teaching/misconceptions.php
Code:
dealingwithcreationisminastronomy.blogspot.com/Re "Schwarzschild Effective Potentials"
Code:
[PLAIN]https://www.physicsforums.com/showthread.php?p=2995779#post2995779[/PLAIN]Most gtr textbooks offer very clear explanations of this; see for example MTW.
Last edited by a moderator:


 ] is the size of the right coset space H\G, so if H is the stabilizer of some "geometric configuration" implicit in some action by G on some set X, there are [G
] is the size of the right coset space H\G, so if H is the stabilizer of some "geometric configuration" implicit in some action by G on some set X, there are [G