Calculating force on a syringe plunger for a viscous fluid?

[DadoNoah]

TL;DR Summary

Trying to derive an equation for the force applied to a syringe plunger as a function of desired flow rate, fluid viscosity, and syringe geometry. Am I using the Poiseuille equation correctly?

I'm currently working on a precise glue/resin dispenser, and I'm trying to derive an equation for the force one must exert on a syringe plunger as a function of the desired flow rate Q, and also accounting for the fluid viscosity and the syringe barrel and needle geometry. I've attached a scan of my calculations using the Poiseuille equation, but it's been quite a while since I've used it, so I've got no idea if I'm doing it correctly. Are you even allowed to daisy-chain the two pipe sections together like I have? If someone could take a look and tell me if I'm on the right track, I'd really appreciate it!

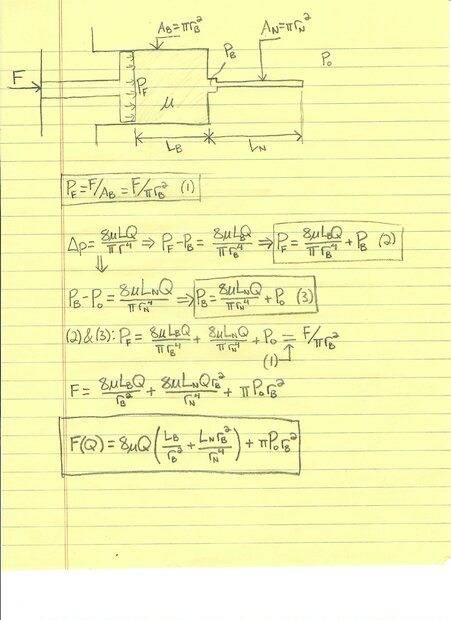
If you're having trouble with my handwriting, the final equation I came up with is:
F(Q) = 8μQ ( L_B/r_B² + L_Nr_B²/r_N⁴ ) + πP₀r_B²

 

[Arjan82]

I think applying Poisseuille on the needle is correct, but on the first part is a bit dodgy since the flow will contract towards the needle and becomes far removed from Poisseuille flow. However, the contribution of that part also might be low.

What you are now forgetting is the acceleration of the flow. This might have a significant contribution (although, for high viscosity fluids maybe not, but you should at least check). By forcing the fluid through the needle you are accelerating it significantly which give rise to a dynamic pressure (0.5*rho*v^2) that will lead to an extra force.

Otherwise I think it is pretty ok.

 

[DadoNoah]

I think applying Poisseuille on the needle is correct, but on the first part is a bit dodgy since the flow will contract towards the needle and becomes far removed from Poisseuille flow. However, the contribution of that part also might be low.

What you are now forgetting is the acceleration of the flow. This might have a significant contribution (although, for high viscosity fluids maybe not, but you should at least check). By forcing the fluid through the needle you are accelerating it significantly which give rise to a dynamic pressure (0.5*rho*v^2) that will lead to an extra force.

Otherwise I think it is pretty ok.

Thanks for the reply Arjan! So if I'm understanding correctly, my current solution will underestimate pressure P_B, and by extension will underestimate force F?

If it helps, I'll be using this setup to apply very small quantities of resin at a time, so my target flow rate Q will likely be very small-- Something under 0.5 mL/s, which, for most syringes I'm considering, translates to a flow speed on the scale of 1 to 2.5 mm/s in the barrel and around .2 to 1 m/s in the needle. For such low fluid velocities, would I be safe in considering the contribution of dynamic pressure to be negligible, or am I just falling victim to wishful thinking?

EDIT: I should also clarify that my solution doesn't need to be exact, it really only needs to tell me whether the syringe will be usable, e.g. F < 30N will be a breeze, 31-50N should be easy enough, 51-60N will take some firm pressure, 61-75N will cramp the hell out of my hand after a few minutes, and 76+N will probably call for superhuman thumbs.

 

[Arjan82]

Well, I was already expecting something like that. You are probably right, but it never hurts to check I guess.

 

[DadoNoah]

Well, I was already expecting something like that. You are probably right, but it never hurts to check I guess.

I couldn't agree more, and I thank you for making me double-check! I feel much more confident now picking out a syringe and taking the plunge *ba-dum tiss*

 

[Chestermiller]

The pressure drop in the needle is going to dominate (by far). And you don't need to include the atmospheric pressure because that is going to cancel out.

What is the viscosity, the needle length, and needle diameter?

 

[DadoNoah]

The pressure drop in the needle is going to dominate (by far). And you don't need to include the atmospheric pressure because that is going to cancel out.

Yeah, I see what you mean about the pressure drop in the needle. That 1/r_N⁴ term really blows up. But could you elaborate about atmospheric pressure balancing out? Am I missing an additional P₀ term acting on the plunger?

What is the viscosity, the needle length, and needle diameter?

The viscosity of the resin is around ~930 cP, or ~0.93 Pa*s. I'm still shopping around for which syringe I'll be using, but most of them are 2-3 cm long, and the gauge can be any standard needle gauge:

Based on the numbers I've plugged in so far, it seems unlikely that anything over 18ga will work.

 

[Chestermiller]

Yeah, I see what you mean about the pressure drop in the needle. That 1/r_N⁴ term really blows up. But could you elaborate about atmospheric pressure balancing out? Am I missing an additional P₀ term acting on the plunger?

Sure. Without pushing on the plunger, your thumb is already exerting a pressure of 1 bar on the surrounding air, and the air is already exerting a force of 1 bar on the plunger. The force your thumb feels when you press on the plunger is the part that exceeds the atmospheric pressure force.

The viscosity of the resin is around ~930 cP, or ~0.93 Pa*s. I'm still shopping around for which syringe I'll be using, but most of them are 2-3 cm long, and the gauge can be any standard needle gauge: View attachment 292063
Based on the numbers I've plugged in so far, it seems unlikely that anything over 18ga will work.

At 0.5 cc/sec, for 18 gauge, the shear rate at the wall will be 8600 sec^-1, and the shear stress at the wall will be 80000 dynes/cm^2. So the pressure drop for a 2 cm long needle will be $7.6\times 10^6\ dynes/cm^2=760000\ Pa=7.6\ bars$. That seems like a pretty high pressure. For a 1 cm^2 piston, that would give a force of 76 N (if I did the math correctly).

 

[DadoNoah]

At 0.5 cc/sec, for 18 gauge, the shear rate at the wall will be 8600 sec^-1, and the shear stress at the wall will be 80000 dynes/cm^2. So the pressure drop for a 2 cm long needle will be $7.6\times 10^6\ dynes/cm^2=760000\ Pa=7.6\ bars$. That seems like a pretty high pressure. For a 1 cm^2 piston, that would give a force of 76 N (if I did the math correctly).

Yeah, I think you're right-- I updated my formula to cancel out the P₀ term per your advice, and it gives me about 79 N for a 1 cm² piston. However, I should note that 0.5 cc/sec is the absolute maximum flow rate I foresee needing. Plugging 0.4 cc/sec into my Poiseuille-based formula gives me a much more reasonable force of 59 N, and .25 cc/sec gives an even more reasonable 37 N. Does that jive with your shear rate-based calculations as well?

 

[Chestermiller]

Yeah, I think you're right-- I updated my formula to cancel out the P₀ term per your advice, and it gives me about 79 N for a 1 cm² piston. However, I should note that 0.5 cc/sec is the absolute maximum flow rate I foresee needing. Plugging 0.4 cc/sec into my Poiseuille-based formula gives me a much more reasonable force of 59 N, and .25 cc/sec gives an even more reasonable 37 N. Does that jive with your shear rate-based calculations as well?

Well, the force is directly proportional to the flow rate.

 

[DadoNoah]

Well, the force is directly proportional to the flow rate.

Certainly, but I meant more like, do your shear rate calculations yield similar force values to my Poiseuille calculations at 0.4 cc/sec and 0.25 cc/sec?