Calculating Half life Decay in an Open system

[pac0master]

[Question]
So I was thinking about Physics for some time and for the sake of
curiosity I've came with this problem:

Let's say we have a liquid flowing into a system with infinite
space.

The flow is constant ( F )
The liquid decays over time with a half life ( λ )
We're looking for the Total mass (or volume (M') left in the system
after a certain amount of time ( T )

Example:
If a liquid with a Half life of 5 Days flows in a container at a
rate of 250'000L per days, How much will we have left after 27
Days

[Difficulty]
I've been told that I need Calculus, Something like Integral,
Unfortunately I never learned it.
This is also simply a question from Curiosity, There is no plan in my near future to learn or use Calculus. meaning that to solve it myself, I would need to works months or Years learning a new type of mathematics.

I'm just curious to know how to solve this.
It was inspired by the Fukushima disaster where some nuclear contamination is dumped into the Ocean
Of course it's oversimplified.

[Thoughts]
I could calculate it if it was a Closed system, (where no matter
enters or leave the system(other than the decay))

Here I did an image with the formula I've "created" (was bored at school)

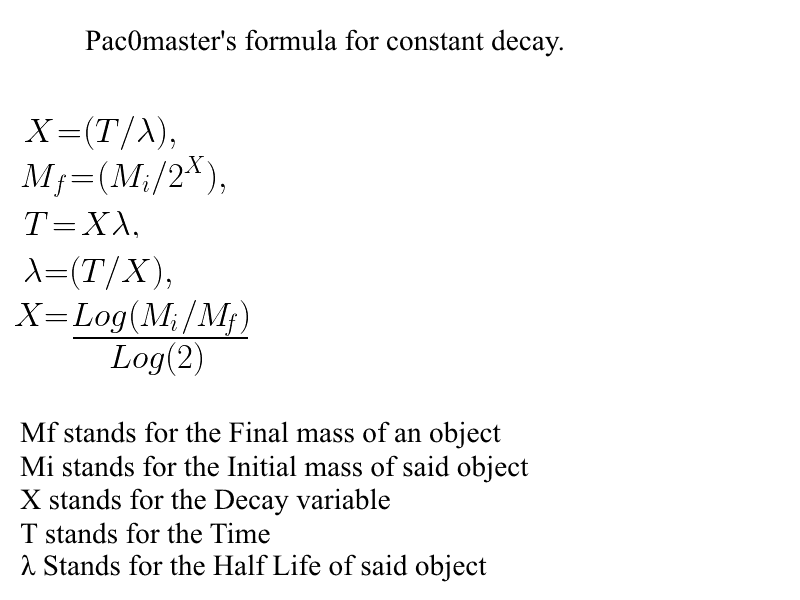
It's basic but it works. I've also made it into an Excel Sheet which is pretty cool.
So it rise the question. Is it possible to solve my problem in Excel as well, or perhaps softwares like Wolfram Alpha?
But just like I've stated above, It only works for closed system, where we start with an initial amount and let the decay do it job.
It's a simplified version of the C14 Dating method..Thanks for the help

 

[mfb]

The initial amount of matter is zero? You can simulate it in small time steps, but you can also find an analytic formula. It has the form $M(t)=a(1-e^{-ct})$ where you have to find a and c as function of the half-life and flow rate. Note the similarity to exponential decay with a fixed initial amount and no inflow, $M(t)=M_0 e^{-ct}$

 

[pac0master]

The initial amount of matter is zero? You can simulate it in small time steps, but you can also find an analytic formula. It has the form $M(t)=a(1-e^{-ct})$ where you have to find a and c as function of the half-life and flow rate. Note the similarity to exponential decay with a fixed initial amount and no inflow, $M(t)=M_0 e^{-ct}$

Yeah, to simplify it, the initial amount of matter is zero, otherwise just add as much as you wish.

Can you please give me a little example of how the formula works by solving the example I've wrote above?
Just so I get a good idea how this works.

 

[mfb]

It is your task. The usual way to derive this is to set up a differential equation and to solve it. I already gave the general solution to this equation here, you just have to find coefficients that work for your specific example.

Adding initial material works by modifying the "1" in the formula.

 

[pac0master]

Well, I never studied Differential equation.
It's a little mathematical problems I've created based on the Fukushima disaster.

Some people might think I'm lazy, but I'd rather ask for a quick answer over the internet rather than works months or years to learn something new just to manage to answer one single question.
Well, there is no plan in my near future to learn Calculus in any form as it won't be required in my area of work. (Industrial Drafting and Video game Design)
I ask this purely out of curiosity because I would like to know the answer

Sorry if I bother anyone.
It's just a little thing that come to my mind a while ago.
I figured how to solve the simple version (closed system) using algebra and logarithm. but this one is above my current skills.